0708-1300/Class notes for Tuesday, November 27

0708-1300/Navigation Panel   Add your name / see who's in! # Week of... Links Fall Semester 1 Sep 10 About, Tue, Thu 2 Sep 17 Tue, HW1, Thu 3 Sep 24 Tue, Photo, Thu 4 Oct 1 Questionnaire, Tue, HW2, Thu 5 Oct 8 Thanksgiving, Tue, Thu 6 Oct 15 Tue, HW3, Thu 7 Oct 22 Tue, Thu 8 Oct 29 Tue, HW4, Thu, Hilbert sphere 9 Nov 5 Tue,Thu, TE1 10 Nov 12 Tue, Thu 11 Nov 19 Tue, Thu, HW5 12 Nov 26 Tue, Thu 13 Dec 3 Tue, Thu, HW6 Spring Semester 14 Jan 7 Tue, Thu, HW7 15 Jan 14 Tue, Thu 16 Jan 21 Tue, Thu, HW8 17 Jan 28 Tue, Thu 18 Feb 4 Tue 19 Feb 11 TE2, Tue, HW9, Thu, Feb 17: last chance to drop class R Feb 18 Reading week 20 Feb 25 Tue, Thu, HW10 21 Mar 3 Tue, Thu 22 Mar 10 Tue, Thu, HW11 23 Mar 17 Tue, Thu 24 Mar 24 Tue, HW12, Thu 25 Mar 31 Referendum,Tue, Thu 26 Apr 7 Tue, Thu R Apr 14 Office hours R Apr 21 Office hours F Apr 28 Office hours, Final (Fri, May 2) Register of Good Deeds Errata to Bredon's Book

Announcements go here

Today's Agenda

• The planimeter with a picture from http://whistleralley.com/planimeter/planimeter.htm but our very own plane geometry and Stokes' theorem.
• Completion of the proof of Stokes' theorem.
• Completion of the discussion of the two- and three-dimensional cases of Stokes' theorem.
• With luck, a discussion of de-Rham cohomology, homotopy invariance and Poincaré's lemma.

Class Notes

The notes below are by the students and for the students. Hopefully they are useful, but they come with no guarantee of any kind.

First Hour

Planimeter

A planimeter consists of two rods connected with a join where the end of one rod is fixed (but free to rotate) and the opposing end of the second rod traces out the boundary of some surface on the plane. I.e., the planimeter is kind of like a 1 legged roach. At the join of the two rods is a wheel which rotates (and measures the rotation) when the rod tracing the boundary moves in the normal direction and simply slides back an forth when moved in a tangential direction.

Now we recall from plane geometry that we can locate points in the polar form Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (r,\theta)} and have the equations Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = rcos\theta} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y = rsin\theta}

Hence,

$dx=cos\theta dr-rsin\theta d\theta$

$dy=sin\theta dr+rcos\theta d\theta$

Hence $dx\wedge dy=r(cos^{2}\theta +sin^{2}\theta )dr\wedge d\theta =rdr\wedge d\theta$

Now, the planimeter is essentially a 1 form corresponding to the speed of the wheel. We consider a diagram where the angle from the horizontal at the fixed end of the planimeter to the measuring end is $\theta$ and the angle from the horizontal to the first rod (the one connected to the fixed point) is $\theta +\phi$. Hence $r=2cos\phi$ and $dr=-2sin\phi d\phi$

With a little plane geometry we can see that $\omega =cos2\phi d(\theta +\phi )$

Computing,

$d\omega =-2sin2\phi d\phi \wedge (d\theta +d\phi )=-4sin\phi cos\phi d\phi \wedge d\theta =2cos\phi dr\wedge d\theta =rdr\wedge d\theta =dx\wedge dy$

Now applying stokes theorem, the the planimeter integrates $\omega$ over the boundary of our surface and hence this is just the integral of $d\omega$ over the surface. But this is just the integral of the area form.

Hence the planimeter measure the area of a surface.

Back to Stokes Theorem

Firstly recall that $\partial M$ is oriented so that if you prepend the outward normal to its orientation you get the orientation of M

Alternatively we recall that neighborhoods of points on the boundary look like the half space. Hence we can choose to restrict our attention to atlas's where all charts look like $H=\{x\in \mathbb {R} ^{n}:x_{1}\leq 0\}$

We can see that these orientations are the same, i.e., just prepend the outward normal to the half space.

Proof of Stokes

We have now defined all the terms. WLOG $\omega$ is supported in one chart (by linearity)

For a compactly supported n-1 form on H need to show that $\int _{\partial H}\omega =\int _{H}d\omega$

We let $\omega =\sum f_{i}dx_{1}\wedge \cdots \wedge {\hat {dx_{i}}}\wedge \cdots \wedge dx_{n}$ (where the hat means it is omitted)

$d\omega =\sum (-1)^{i-1}{\frac {\partial f_{i}}{\partial x_{i}}}dx_{1}\wedge \cdots \wedge dx_{n}$

So, $\int _{H}d\omega =\sum \int _{[x_{1}\leq 0]}(-1)^{i-1}{\frac {\partial f_{i}}{\partial x_{i}}}dx_{1}\wedge \cdots \wedge dx_{n}=\sum (-1)^{i-1}\int _{[x_{1}\leq 0]}{\frac {\partial f_{i}}{\partial x_{i}}}$

via fundamental theorem of calculus and that the $f_{i}$'s are compactly supported we get

$=\int _{[x_{1}\leq 0]}{\frac {\partial f_{1}}{\partial x_{1}}}=\int _{[x_{1}=0]}f_{1}$

Hence with the standard inclusion of $\partial H=\mathbb {R} _{x_{2}\cdots x_{n}}^{n-1}$ we get

$\int _{\partial H}\omega =\int _{\mathbb {R} _{x_{2}\cdots x_{n}}^{n-1}}\iota ^{*}(\sum f_{i}dx_{1}\wedge \cdots \wedge {\hat {dx_{i}}}\wedge \cdots \wedge dx_{n})=\int _{[x_{1}=0]}f_{1}$

Thus these are the same and the theorem is proved Q.E.D.

Real Plane

Consider $\Omega ^{0}(\mathbb {R} ^{2})\rightarrow ^{d}\Omega ^{1}(\mathbb {R} ^{2})\rightarrow ^{d}\Omega ^{2}(\mathbb {R} ^{2})$

Forms in $\Omega ^{1}(\mathbb {R} ^{2})$ look like $Fdx+Gdy$ and map under d to $(G_{x}-F_{y})dx\wedge dy$

Hence applying Stokes' Theorem:

$\int _{\partial D}Fdx+Gdy=\int _{D}(G_{x}-F_{y})dxdy$

This is known as Greens Theorem

In complex analysis we also have a similar result Cauchy's Theorem where the integral of an analytic function around a closed path is zero. This is because analytic functions obey the Cauchy-Riemann equations and hence $G_{x}-F_{y}$ is identically zero.

Second Hour

Example 2

$\Omega ^{k}(\mathbb {R} ^{3})$

Recall previous we had consider the spaces $\Omega ^{k}(\mathbb {R} ^{3})$ and showed that $\Omega ^{0}$ and $\Omega ^{3}$ corresponded with functions and that $\Omega ^{2}$ and $\Omega ^{1}$ corresponded with triples of functions (i.e. vector fields). We also showed that the d functions between these spaces are the gradient, curl and divergence functions from vector calculus.

We are now interested in integrating, using Stokes Theorem, forms in these spaces.

First, note that to a 0 manifold, assigning an orientation to the manifold is just assigning a plus or minus sign to the manifold as a result of it having a trivial basis.

This is consistent with 0 manifolds being the boundary of 1 manifolds. Indeed,

$\int _{\pm p_{0}}\omega _{0}=\sum \pm f(p_{i})$

Now consider a path $\gamma :[0,1]\rightarrow \mathbb {R} ^{3}$

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_{\gamma}\omega_1 = \int_{[0,1]}\gamma^*\omega_1 = \int_{[0,1]}\sum f_i d\gamma^*(x_i) = \int_{[0,1]}\sum f_i d\gamma_i}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle = \int_{[0,1]}\sum f_i\dot{\gamma}_i dt = \int_{\gamma}\vec F\cdot \vec T_{\gamma}}

Now lets compute Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_2(v,w)}

First, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle dx_2\wedge dx_3 (v,w) = v_2 w_3 - v_3 w_2}

Likewise for each component of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_2} we thus get

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_2(v,w) = \vec G(p)\cdot (v\times w)} where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec G(p)} is the vector of coefficients of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_2}

Now we know that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle v\times w} is a vector perpendicular to v and w with magnitude equal to the area of the defined parallelogram. So,

$\int _{\Sigma }\omega _{2}=\int _{\Sigma }{\vec {G}}\cdot {\vec {n}}d\sigma$

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec n} denotes the normal vector and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\sigma} is the area form and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Sigma} is a surface

Now for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_3} ,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_D \omega_3 = \int_D g}

now, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(\gamma(1)) - f(\gamma(0)) = \int_{\gamma} (grad\ f)\cdot\vec T}

and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_D div\ G = \int_{\partial D} G\cdot\vec n d\sigma}

This is Gauss' Divergence Theorem.

We can think about this as saying that the flow from each point in a domain, when summed up, will be just the flow out of the boundary of the domain.

We also get Stokes' Theorem:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_{\partial\Sigma} F\cdot\vec T = \int_{\Sigma} curl\ F\cdot\vec n d\sigma}

End of Example

We recall that since Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d^2 = 0} , if Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega = d\lambda} then Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\omega = 0} . But is the converse true? The following Lemma says 'yes', if the domain is $\mathbb {R} ^{n}$

Poincare's Lemma

On Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^n, d\omega = 0} iff Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exists\lambda} such that $\omega =d\lambda$

This is NOT true for general M, as our homework assignment showed since we had a form Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\theta} that had Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d(d\theta) = 0} but was not d of a form.

Likewise, on Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbb{R}^n-\{0\}} we have Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega = \frac{1}{||x||^{\alpha}}\sum_{i=1}^{n}x_i dx_1\wedge\cdots\wedge\hat{dx_i}\wedge\cdots\wedge dx_n \in\Omega^{n-1}(\mathbb{R}^n-\{0\})}

Claim:

For appropriate Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha,\ d\omega = 0} but Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exists} no Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} such that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\lambda = \omega}

This is in our next homework assignment.

Now, if there was such a Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_{\Sigma}\omega = \int_{\sigma}d\lambda = \int_{\partial\Sigma}\lambda = 0}

If Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \partial\Sigma = \empty} (such as any sphere)

But, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_{S^2}\omega = 4\pi}

Definition

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z^k(M) := ker d|_{\Omega^k(M)}}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B^k(M) := im d|_{\Omega^{k-1}(M)}}

Clearly Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle B^k\subset Z^k} so the following definition makes sense:

Definition (de-Rham Cohomology)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H^k(M):= Z^k(M)/B^k(M)}

Claim

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H^k(\mathbb{R}^n) = 0} yet Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H^1(S^1)\neq 0} .

Also, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H^{n-1}(\mathbb{R}^n - \{x\})\neq 0}

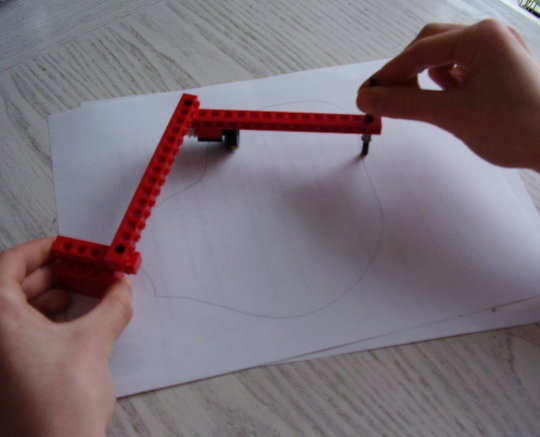
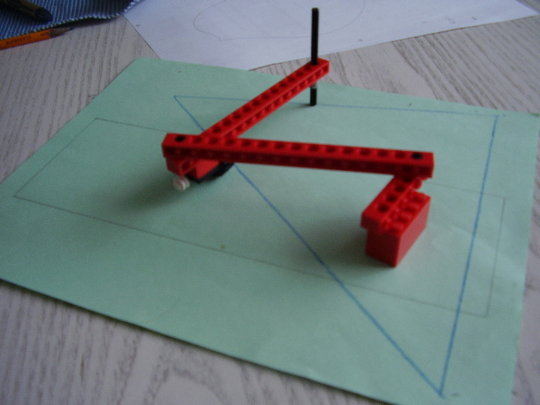
More fun with the planimeter

You can build your own low-tech planimeter out of lego!

Here my left hand is holding the fixed point steady while the right hand traces the shape. The net number of times the wheel turns while the shape is traced out indicates the area.

The empirical test: the two shapes below have the same area, and while tracing them the wheel turned almost exactly the same number of times (about 2.3 rotations). Moreover, tracing smaller shapes caused it to turn fewer times, larger shapes more.

The problem: I couldn't do better than this. Calibrating it (calculating how many rotations corresponds to exactly what area) was a nightmare. Mathematically it was possible, but my planimeter is not accurate enough to agree with my math. If you wish to build your own more accurate one, you might want to try using a thinner wheel or one that grips the table better so you don't accidently lose any turning motion.