0708-1300/Class notes for Tuesday, September 11

0708-1300/Navigation Panel   Add your name / see who's in! # Week of... Links Fall Semester 1 Sep 10 About, Tue, Thu 2 Sep 17 Tue, HW1, Thu 3 Sep 24 Tue, Photo, Thu 4 Oct 1 Questionnaire, Tue, HW2, Thu 5 Oct 8 Thanksgiving, Tue, Thu 6 Oct 15 Tue, HW3, Thu 7 Oct 22 Tue, Thu 8 Oct 29 Tue, HW4, Thu, Hilbert sphere 9 Nov 5 Tue,Thu, TE1 10 Nov 12 Tue, Thu 11 Nov 19 Tue, Thu, HW5 12 Nov 26 Tue, Thu 13 Dec 3 Tue, Thu, HW6 Spring Semester 14 Jan 7 Tue, Thu, HW7 15 Jan 14 Tue, Thu 16 Jan 21 Tue, Thu, HW8 17 Jan 28 Tue, Thu 18 Feb 4 Tue 19 Feb 11 TE2, Tue, HW9, Thu, Feb 17: last chance to drop class R Feb 18 Reading week 20 Feb 25 Tue, Thu, HW10 21 Mar 3 Tue, Thu 22 Mar 10 Tue, Thu, HW11 23 Mar 17 Tue, Thu 24 Mar 24 Tue, HW12, Thu 25 Mar 31 Referendum,Tue, Thu 26 Apr 7 Tue, Thu R Apr 14 Office hours R Apr 21 Office hours F Apr 28 Office hours, Final (Fri, May 2) Register of Good Deeds Errata to Bredon's Book

Announcements go here

In Small Scales, Everything's Linear

	$\longrightarrow$
$z$	$\mapsto$	$z^{2}$

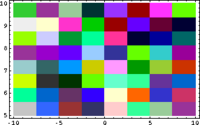
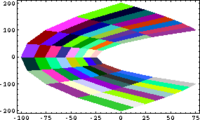
Code in Mathematica:

QuiltPlot[{f_,g_}, {x_, xmin_, xmax_, nx_}, {y_, ymin_, ymax_, ny_}] :=
Module[
  {dx, dy, grid, ix, iy},
  SeedRandom[1];
  dx=(xmax-xmin)/nx;
  dy=(ymax-ymin)/ny;
  grid = Table[
    {x -> xmin+ix*dx, y -> ymin+iy*dy},
    {ix, 0, nx}, {iy, 0, ny}
  ];
  grid = Map[({f, g} /. #)&, grid, {2}];
  Show[
    Graphics[Table[
      {
        RGBColor[Random[], Random[], Random[]],
        Polygon[{
          grid[[ix, iy]],
          grid[[ix+1, iy]],
          grid[[ix+1, iy+1]],
          grid[[ix, iy+1]]
        }]
      },
      {ix, nx}, {iy, ny}
    ]],
    Frame -> True
  ]
]

QuiltPlot[{x, y}, {x, -10, 10, 8}, {y, 5, 10, 8}]
QuiltPlot[{x^2-y^2, 2*x*y}, {x, -10, 10, 8}, {y, 5, 10, 8}]

See also 06-240/Linear Algebra - Why We Care.

Class Notes

Let $\$f:V\rightarrow W\$$ be a function defined in a neighborhood of the point $\$x\$$