06-1350/Class Notes for Thursday November 16: Difference between revisions

From Drorbn
Jump to navigationJump to search
No edit summary
 
No edit summary
 
(4 intermediate revisions by the same user not shown)
Line 1: Line 1:
{{06-1350/Navigation}}
{{06-1350/Navigation}}

{{In Preparation}}


==Today's Agenda==
==Today's Agenda==

===Formulas are a Chore (Bore?)===


* Sweeping clean a tree and <math>{\mathcal A}(\Gamma)\equiv{\mathcal A}(\uparrow_{b_1(\Gamma)})</math>.
* Sweeping clean a tree and <math>{\mathcal A}(\Gamma)\equiv{\mathcal A}(\uparrow_{b_1(\Gamma)})</math>.
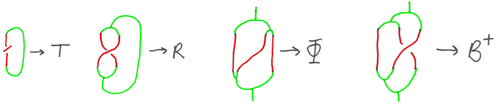
[[Image:06-1350-TRPhiB.png|center|500px]]
[[Image:06-1350-TRPhiB.png|center|500px]]

* <math>{\mathcal A}(\uparrow_n)</math> is a VS-algebra (see more at [[VS, TS and TG Algebras]]).
* <math>{\mathcal A}(\uparrow_n)</math> is a VS-algebra (see more at [[VS, TS and TG Algebras]]).

* In the coordinates above, write the <math>TR\Phi B</math> relations in various algebraic notations.
* In the coordinates above, write the <math>TR\Phi B</math> relations in various algebraic notations.
** R4: <math>(1230)^\star B^\pm\cdot(1213)^\star B^\pm\cdot(1023)^\star\Phi=(1123)^\star\Phi\cdot(1233)^\star B^\pm</math>.
** R4: <math>(1230)^\star B\cdot(1213)^\star B\cdot(1023)^\star\Phi=(1123)^\star\Phi\cdot(1233)^\star B</math> or <math>(B_{1a}B_{2a}\Phi_{1a}; B_{1b}B_{2b}; B_{1c}B_{2a}\Phi_{1b}; B_{2c}\Phi_{1c}) = (\Phi_{2a}B_{3a}; \Phi_{2a}B_{3b}; \Phi_{2b}B_{3c}; \Phi_{2c}B_{3c})</math>.
** R3: <math>(1230)^\star B\cdot(1213)^\star B\cdot(1023)^\star B = (1123)^\star B\cdot(1203)^\star B\cdot(1231)^\star B</math> or <math>(B_{1a}B_{2a}B_{3a}; B_{1b}B_{2b}; B_{1c}B_{2a}B_{3b}; B_{2c}B_{3c}) = (B_{4a}B_{5a}B_{6a}; B_{4a}B_{5b}B_{6b}; B_{4b}B_{6c}; B_{4c}B_{5c}B_{6a})</math>.
⚫
** <math>B^{\pm}</math> in terms of <math>\Phi</math> and <math>R</math> and <math>R</math> in terms of <math>T</math>.
** R2: <math>(123)^\star B^\pm\cdot(132)^\star B^\mp=1_3</math> or <math>(B^\pm_{1a}B^\mp_{2a}; B^\pm_{2b}B^\mp_{3c}; B^\pm_{1c}B^\mp_{2b}) = (1;1;1)</math>.
** R3, R2, R1
** R1: <math>(B^\pm_a; B^\pm_bB^\pm_c)=(1;T^{\pm 2})</math>.
* But for now, skip the writing of the following relations:
** Symmetry of <math>\Phi</math> and of <math>B^{\pm}</math>.
** Symmetry of <math>\Phi</math> and of <math>B^{\pm}</math>.
** <math>u</math>, <math>d</math> and <math>\#</math>
** <math>u</math>, <math>d</math> and <math>\#</math>
** Idempotence for <math>T</math>, <math>R</math>, <math>\Phi</math> and <math>B^{\pm}</math>.
** Idempotence for <math>T</math>, <math>R</math>, <math>\Phi</math> and <math>B^{\pm}</math>.
⚫
** <math>B^{\pm}</math> in terms of <math>\Phi</math> and <math>R</math> and <math>R</math> in terms of <math>T</math>.

===Exponentiation is a Miracle===

* Description of the problem.
* Beads and strands.
* The perturbative approach, linearization.
* The syzygies: relations between the errors.
* The Hochschild complex and homology.

Latest revision as of 09:29, 16 November 2006

06-1350/Navigation Panel  
# Week of... Links
1 Sep 11 About, Tue, Thu
2 Sep 18 Tue, Kurlin(P), Thu
3 Sep 25 Tue, Photo, Thu
4 Oct 2 HW1, Tue, Thu
5 Oct 9 Tue(P), Thu(P)
6 Oct 16 HW2, Tue(P), Thu(P)
7 Oct 23 Tue(P), Thu
8 Oct 30 HW3, Tue, Thu
9 Nov 6 Tue (), Thu
10 Nov 13 Tue, Thu
11 Nov 20 HW4(P), Thu
12 Nov 27 Thu
13 Dec 4 Syzygies in Asymptote, Final
Jan 8 Grades
Note. (P) means "contains a problem that Dror cares about".
06-1350-ClassPhoto.jpg
Add your name / see who's in!
On to 07-1352

Today's Agenda

Formulas are a Chore (Bore?)

  • Sweeping clean a tree and .
06-1350-TRPhiB.png
  • is a VS-algebra (see more at VS, TS and TG Algebras).
  • In the coordinates above, write the relations in various algebraic notations.
    • R4: or .
    • R3: or .
    • R2: or .
    • R1: .
  • But for now, skip the writing of the following relations:
    • Symmetry of and of .
    • , and
    • Idempotence for , , and .
    • in terms of and and in terms of .

Exponentiation is a Miracle

  • Description of the problem.
  • Beads and strands.
  • The perturbative approach, linearization.
  • The syzygies: relations between the errors.
  • The Hochschild complex and homology.
Retrieved from "https://drorbn.net/index.php?title=06-1350/Class_Notes_for_Thursday_November_16&oldid=2773"