12-240/Classnotes for Thursday October 18

DBN: Classes: 2012-13: 12-240 / Navigation Additions to this web site no longer count towards good deed points. # Week of... Notes and Links 1 Sep 10 About This Class, Tuesday, Thursday 2 Sep 17 HW1, Tuesday, Thursday, HW1 Solutions 3 Sep 24 HW2, Tuesday, Class Photo, Thursday 4 Oct 1 HW3, Tuesday, Thursday 5 Oct 8 HW4, Tuesday, Thursday 6 Oct 15 Tuesday, Thursday 7 Oct 22 HW5, Tuesday, Term Test was on Thursday. HW5 Solutions 8 Oct 29 Why LinAlg?, HW6, Tuesday, Thursday, Nov 4 is the last day to drop this class 9 Nov 5 Tuesday, Thursday 10 Nov 12 Monday-Tuesday is UofT November break, HW7, Thursday 11 Nov 19 HW8, Tuesday,Thursday 12 Nov 26 HW9, Tuesday , Thursday 13 Dec 3 Tuesday UofT Fall Semester ends Wednesday F Dec 10 The Final Exam (time, place, style, office hours times) Register of Good Deeds Add your name / see who's in!

Riddle Along

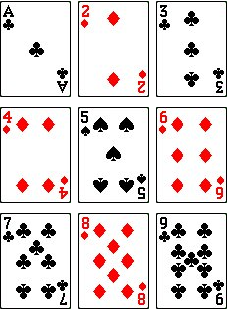
The game of 15 is played as follows. Two players alternate choosing cards numbered between 1 and 9, with repetitions forbidden, so that the game ends at most after 9 moves (or four and a half rounds). The first player to have within her/his cards a set of precisely 3 cards that add up to 15 wins.

Does this game has a winning strategy? What is it? Who wins, the first to move or the second? Why am I asking this question at this particular time?

See also a video and the transcript of that video.

Dror's notes above / Students' notes below

Theorems

1. If G generates, |G| Failed to parse (unknown function "\gre"): {\displaystyle \gre \!\,} n and G contains a basic, |G|=n then G is a basic

class note

• 

  page1

• 

  page2

• 

  page3