| #
|
Week of...
|
Notes and Links
|
| Additions to the MAT 1100 web site no longer count towards good deed points
|
| 1
|
Sep 12
|
About This Class, Tuesday - Non Commutative Gaussian Elimination, Thursday - NCGE completed, the category of groups, images and kernels.
|
| 2
|
Sep 19
|
I'll be in Strasbourg, class was be taught by Paul Selick. See my summary.
|
| 3
|
Sep 26
|
Class Photo, HW1, HW1 Submissions, HW 1 Solutions
|
| 4
|
Oct 3
|
The Simplicity of the Alternating Groups
|
| 5
|
Oct 10
|
HW2, HW 2 Solutions
|
| 6
|
Oct 17
|
Groups of Order 60 and 84
|
| 7
|
Oct 24
|
Extra office hours: Monday 10:30-12:30 (Dror), 5PM-7PM @ Huron 1028 (Stephen). Term Test on Tuesday. Summary for the midterm exam. Term Test - Sample Solutions
|
| 8
|
Oct 31
|
HW3, HW 3 Solutions
|
| 9
|
Nov 7
|
Monday-Tuesday is November Break, One Theorem, Two Corollaries, Four Weeks
|
| 10
|
Nov 14
|
HW4, HW 4 Solutions
|
| 11
|
Nov 21
|
|
| 12
|
Nov 28
|
HW5 and last week's schedule
|
| 13
|
Dec 5
|
Tuesday - the Jordan form; UofT Fall Semester ends Wednesday; our Final Exam took place on Friday
|
| Register of Good Deeds
|

Add your name / see who's in!
|
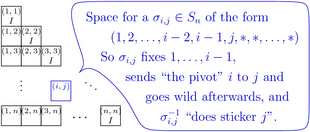
See Non Commutative Gaussian Elimination
|
|
The following is the proof for the simplicity of  . It is available individually in pdf format, can be found in the course notes, or on another user page.
. It is available individually in pdf format, can be found in the course notes, or on another user page.
Theorem: The alternating group  is simple for
is simple for  .
.
Note that for  this is trivial. For
this is trivial. For  we have that
we have that  which is an abelian group of prime-order, and hence simple.
which is an abelian group of prime-order, and hence simple.
We know that for  is not simple. Indeed, we have seen that there is a non-trivial homomorphism
is not simple. Indeed, we have seen that there is a non-trivial homomorphism  By restricting
By restricting  to
to  we still have a non-trivial homomorphism whose kernel is non-trivial. This kernel is a normal subgroup.
we still have a non-trivial homomorphism whose kernel is non-trivial. This kernel is a normal subgroup.
We will need the following Lemmas for our proof.
Lemma: Every element of  is a product of 3-cycles.
is a product of 3-cycles.
Proof
- Every
 is a product of an even number of 2-cycles. Without loss of generality, we will demonstrate this on the following "cycles. Indeed,
is a product of an even number of 2-cycles. Without loss of generality, we will demonstrate this on the following "cycles. Indeed,

Lemma: If  contains a 3-cycle, then
contains a 3-cycle, then  .
.
Proof
- Without loss of generality, we can consider
 We want to show that for all
We want to show that for all  we must have that
we must have that  . If
. If  then this is clear since
then this is clear since  is normal in
is normal in ; otherwise, take
; otherwise, take  with
with  . Since
. Since  we have that
we have that  So
So  contains all three cycles.
contains all three cycles.
Proof [Proof of Theorem]
- Let
 . By the previous two lemmas, it is sufficient to show that
. By the previous two lemmas, it is sufficient to show that  contains a three cycle.
contains a three cycle.
- "Case 1:"
 contains an element with cycle length at least 4.
contains an element with cycle length at least 4.
- Let
 . Now
. Now  is normal in
is normal in  so
so  . Similarly, multiplying by
. Similarly, multiplying by  will keep the element in
will keep the element in  , so
, so  and
and  contains a three cycle.
contains a three cycle.
- Case 2:
 contains an element with two cycles of length 3.
contains an element with two cycles of length 3.
- Let
 . Then by the same reasoning as before
. Then by the same reasoning as before  and can be computed as
and can be computed as  . We can now use Case 1 to deduce that
. We can now use Case 1 to deduce that  has a three cycle.
has a three cycle.
- Case 3:
 contains an element that is a three-cycle and a product of disjoint transpositions. Write
contains an element that is a three-cycle and a product of disjoint transpositions. Write  and note that
and note that 
- Then
 but the elements of
but the elements of  are disjoint with
are disjoint with  , and we conclude that
, and we conclude that  and
and  commute; that is,
commute; that is,  . Thus
. Thus  and
and  contains a three cycle.
contains a three cycle.
- Case 4: Finally, consider the case when the element of
 is a product of disjoint 2-cycles.
is a product of disjoint 2-cycles.
- Write
 . By previous rationale, we know that
. By previous rationale, we know that  and can be computed as
and can be computed as  We can view this as a sort of purification, in that we have simplified a product of disjoint 2-cycles of arbitrary length into the case of two disjoint 2-cycles. Applying this procedure again, we get
We can view this as a sort of purification, in that we have simplified a product of disjoint 2-cycles of arbitrary length into the case of two disjoint 2-cycles. Applying this procedure again, we get  and we again refer to Case 1 to conclude
and we again refer to Case 1 to conclude  contains a three cycle.
contains a three cycle.
Note that this last case is the only case in which we did not assume "5" was part of the hypothesis, but needed to use it. Hence we need  for this case to hold.
for this case to hold.









































