11-1100/Homework Assignment 3

In Preparation

The information below is preliminary and cannot be trusted! (v)

DBN: Classes: 2011-12: 11-1100/Navigation # Week of... Notes and Links Additions to the MAT 1100 web site no longer count towards good deed points 1 Sep 12 About This Class, Tuesday - Non Commutative Gaussian Elimination, Thursday - NCGE completed, the category of groups, images and kernels. 2 Sep 19 I'll be in Strasbourg, class was be taught by Paul Selick. See my summary. 3 Sep 26 Class Photo, HW1, HW1 Submissions, HW 1 Solutions 4 Oct 3 The Simplicity of the Alternating Groups 5 Oct 10 HW2, HW 2 Solutions 6 Oct 17 Groups of Order 60 and 84 7 Oct 24 Extra office hours: Monday 10:30-12:30 (Dror), 5PM-7PM @ Huron 1028 (Stephen). Term Test on Tuesday. Summary for the midterm exam. Term Test - Sample Solutions 8 Oct 31 HW3, HW 3 Solutions 9 Nov 7 Monday-Tuesday is November Break, One Theorem, Two Corollaries, Four Weeks 10 Nov 14 HW4, HW 4 Solutions 11 Nov 21 12 Nov 28 HW5 and last week's schedule 13 Dec 5 Tuesday - the Jordan form; UofT Fall Semester ends Wednesday; our Final Exam took place on Friday Register of Good Deeds Add your name / see who's in! 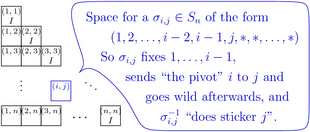See Non Commutative Gaussian Elimination

This assignment is due at class time on Tuesday, November 15, 2011.

Solve the following questions

Problem 1. (Selick) Show that any group of order 56 has a normal Sylow-$p$ subgroup, for some prime $p$ dividing 56.

Problem 2. (Qualifying exam, May 1997) Let $S_{5}$ act on $({\mathbb {Z} /5})^{5}$ by permuting the factors, and let $G$ be the semi-direct product of $S_{5}$ and $({\mathbb {Z} /5})^{5}$.

1. What is the order of $G$?
2. How many Sylow-5 subgroups does $G$ have? Write down one of them.

Problem 3. (Selick) Show that the group $Q$ of unit quaternions ($\{\pm 1,\pm i,\pm j,\pm k\}$, subject to $i^{2}=j^{2}=k^{2}=-1\in Z(Q)$ and $ij=k$) is not a semi-direct product of two of its proper subgroups.

Problem 4. (Qualifying exam, September 2008) Let $G$ be a finite group and $p$ be a prime. Show that if $H$ is a $p$-subgroup of $G$, then $(N_{G}(H):H)$ is congruent to $(G:H)$ mod $p$. You may wish to study the action of $H$ on $G/H$ by multiplication on the left.

Problem 5. (easy)

1. Prove that in any ring, $(-1)^{2}=1$.
2. Prove that even in a ring without a unit, $(-a)^{2}=a^{2}$.

(Feel free to do the second part first and then to substitute $a=1$).

Problem 6.

1. (Qualifying exam, April 2009) Prove that a finite integral domain is a field.
2. (Qualifying exam, September 2008) Prove that in a finite commutative ring, every prime ideal is maximal.

Problem 7. (Dummit and Foote) A ring $R$ is called a Boolean ring if $a^{2}=a$ for all $a\in R$.

1. Prove that every Boolean ring is commutative.
2. Prove that the only Boolean ring that is also an integral domain is ${\mathbb {Z} }/2$.

Problem 8. (bonus) Let $S$ be the ring of bounded sequences of real numbers with pointwise addition and multiplication, let $I$ be the ideal made of all sequences that are equal to $0$ except in at most finitely many places, and let $J$ be a maximal ideal in $S$ containing $I$.

1. Prove that $S/J\simeq {\mathbb {R} }$.
2. Denote by $\operatorname {Lim} _{J}$ the projection of $S$ to $S/J$ composed with the identification of the latter with ${\mathbb {R} }$, so that $\operatorname {Lim} _{J}:S\to {\mathbb {R} }$. Prove that for any scalar $c\in {\mathbb {R} }$ and any bounded sequences $(a_{n}),(b_{n})\in S$, we have that $\operatorname {Lim} _{J}(ca_{n})=c\cdot \operatorname {Lim} _{J}(a_{n})$, $\operatorname {Lim} _{J}(a_{n}+b_{n})=\operatorname {Lim} _{J}(a_{n})+\operatorname {Lim} _{J}(b_{n})$, and $\operatorname {Lim} _{J}(a_{n}b_{n})=(\operatorname {Lim} _{J}(a_{n}))(\operatorname {Lim} _{J}(b_{n}))$. (Easy, no bonuses for this part).
3. Prove that if $\lim a_{n}=\alpha$ in the ordinary sense of limits of sequences, then $\operatorname {Lim} _{J}a_{n}=\alpha$.
4. Is there a $J$ for which $\operatorname {Lim} _{J}$ is also translation invariant, namely such that $\operatorname {Lim} _{J}(a_{n})=\operatorname {Lim} _{J}(a_{n+1})$? (Again, easy).

(The sum total of all that is that using the axiom of choice you can construct things that are both too good to be true and not really useful anyway. Blame the axiom of choice, don't blame me.)