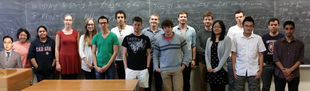14-1100/About This Class

DBN: Classes: 2014-15: 14-1100/Navigation Welcome to Math 1100! (additions to this web site no longer count towards good deed points) # Week of... Notes and Links 1 Sep 8 About This Class; Monday - Non Commutative Gaussian Elimination; Thursday - the category of groups, automorphisms and conjugations, images and kernels. 2 Sep 15 Monday - coset spaces, isomorphism theorems; Thursday - simple groups, Jordan-Holder decomposition series. 3 Sep 22 Monday - alternating groups, group actions, The Simplicity of the Alternating Groups, HW1, HW 1 Solutions, Class Photo; Thursday - group actions, Orbit-Stabilizer Thm, Class Equation. 4 Sep 29 Monday - Cauchy's Thm, Sylow 1; Thursday - Sylow 2. 5 Oct 6 Monday - Sylow 3, semi-direct products, braids; HW2; HW 2 Solutions; Thursday - braids, groups of order 12, Braids 6 Oct 13 No class Monday (Thanksgiving); Thursday - groups of order 12 cont'd. 7 Oct 20 Term Test; Term Test Solutions on Monday, HW3; HW 3 Solutions; Thursday - solvable groups, rings: defn's & examples. 8 Oct 27 Monday - functors, Cayley-Hamilton Thm, ideals, iso thm 1; Thursday - iso thms 2-4, integral domains, maximal ideals, One Theorem, Three Corollaries, Five Weeks 9 Nov 3 Monday - prime ideals, primes & irreducibles, UFD's, Euc.Domain$\Rightarrow$PID, Thursday - Noetherian rings, PID$\Rightarrow$UFD, Euclidean Algorithm, modules: defn & examples, HW4, HW 4 Solutions 10 Nov 10 Monday - R is a PID iff R has a D-H norm, R-modules, direct sums, every f.g. module is given by a presentation matrix, Thursday - row & column reductions plus, existence part of Thm 1 in 1t3c5w handout. 11 Nov 17 Monday-Tuesday is UofT's Fall Break, HW5, Thursday - 1t3c5w handout cont'd, JCF Tricks & Programs handout 12 Nov 24 Monday - JCF Tricks & Programs cont'd, tensor products, Thursday - tensor products cont'd 13 Dec 1 End-of-Course Schedule; Monday - tensor products finale, extension/reduction of scalars, uniqueness part of Thm 1 in 1t3c5w, localization & fields of fractions; Wednesday is a "makeup Monday"!; Notes for Studying for the Final Exam Glossary of terms F Dec 15 The Final Exam Register of Good Deeds Add your name / see who's in! See Non Commutative Gaussian Elimination

Crucial Information

Agenda: Groups, rings, modules.

Course Highlights: We'll solve the Rubik's cube! We'll understand many groups of small order! We'll understand "Modules over PIDs"! We'll know that the quintic can't be solved!

Instructor: Dror Bar-Natan, drorbn@math.toronto.edu, Bahen 6178, 416-946-5438. Office hours: by appointment.

Classes: Mondays 1-3 and Thursdays 1-2 in Bahen 6183.

Teaching Assistant: Louis-Philippe Thibault, lp.thibault@mail.utoronto.ca.

URL: https://drorbn.net/drorbn/index.php?title=14-1100.

Abstract

Taken from the Math Department Course Descriptions:

Basic notions of linear algebra: brief recollection. The language of Hom spaces and the corresponding canonical isomorphisms. Tensor product of vector spaces.

Group Theory: Isomorphism theorems, group actions, Jordan-Hölder theorem, Sylow theorems, direct and semidirect products, finitely generated abelian groups, simple groups, symmetric groups, linear groups, nilpotent and solvable groups, generators and relations.

Ring Theory: Rings, ideals, Euclidean domains, principal ideal domains, and unique factorization domains.

Modules: Modules and algebras over a ring, tensor products, modules over a principal ideal domain.

Text Book(s)

Lang's Algebra, Selick's lecture notes for this class, Dummit and Foote's Abstract Algebra, Hungerford's Abstract Algebra, Etingof's Groups Around Us.

Wiki

The class web site is a wiki, as in Wikipedia - meaning that anyone can and is welcome to edit almost anything and in particular, students can post notes, comments, pictures, whatever. Some rules, though -

• This wiki is a part of my (Dror's) academic web page. All postings on it must be class-related (or related to one of the other projects I'm involved with).
• You must login to edit. To get an account, email me your preferred login name, your real name and your email address if different from the address you are writing from.
• Criticism is fine, but no insults or foul language, please.
• I (Dror) will allow myself to exercise editorial control, when necessary.
• The titles of all pages related to this class should begin with "14-1100/" or with "14-1100-", just like the title of this page.
• For many 14-1100 pages, it is a good idea to put a line containing only the string {{14-1100/Navigation}} at the top of the page. This template inserts the class' "navigation panel" on the top right of the page.
• To edit the navigation panel itself, click on the word "Navigation" on the upper right of the panel. Use caution! Such edits affect many other pages! Note that due to page-caching, such edits take some time to propagate to the pages that include the navigation panel. To force immediate propagation to a given page, reload that page with the string "&action=purge" (meaning: "purge cached version") appended to the page's URL.
• Some further editing help is available at Help:Contents.

Marking Scheme

There will be one term test (25% of the total grade) and a final exam (50%), as well as about 5 homework assignments (25%).

The Term Test

A term test will take place in class on Monday October 20th, 1-3PM. If you missed the term test for a valid reason, the weight of your problem sets will increase to 35% and the weight of your final exam to 65%.

Homework

Assignments will be posted on the course web page and distributed in class (usually on Thursdays) approximately on the weeks shown in the class timeline. They will be due about two weeks later and they will be (at least partially) marked by the TA. In computing the homework grade, your single worst assignment will not count. I encourage you to discuss the assignments with other students or even browse the web, so long as you do at least some of the thinking on your own and you write up your own solutions.

Good Deeds

Students will be able to earn up to 25 "good deeds" points throughout the year for doing services to the class as a whole. There is no pre-set system for awarding these points, but the following will definitely count:

• Drawing a beautiful picture to illustrate a point discussed in class and posting it on this site.
• Taking class notes in nice handwriting, scanning them and posting them here.
• Typing up or formatting somebody else's class notes, correcting them or expanding them in any way.
• Writing an essay on expanding on anything mentioned in class and posting it here; correcting or expanding somebody else's article.
• Doing anything on our 14-1100/To do list.
• Any other service to the class as a whole.

Good deed points will count towards your final grade! If you got $n$ of those, they are solidly yours and the above formula for the final grade will only be applied to the remaining $100-n$ points. So if you got 25 good deed points (say) and your final grade is 80, I will report your grade as $25+80(100-25)/100=85$. Yet you can get an overall 100 even without doing a single good deed.

Important. For your good deeds to count, you must do them under your own name. So you must set up an account for yourself on this wiki and you must use it whenever you edit something. I will periodically check Recent changes to assign good deeds credits. Those credits will be made public (good deeds are public as a whole) towards the end of the course, at 14-1100/Register of Good Deeds.

Important. The good deed points are an extra, a bonus, a treat. Very few will get many, and you should not count of them as a substitute for doing class work.

Class Photo

To help me learn your names, I will take a class photo on Thursday of the third week of classes. I will post the picture on the class' web site and you will be required to send me an email and identify yourself in the picture or to identify yourself on the Class Photo page of this wiki.