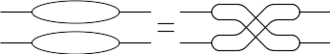The Envelope of The Alexander Polynomial

Paperlets Navigation Panel   Algebraic Knot Theory Algebraic Knot Theory - A Call for Action. The Kontsevich Integral for Knotted Trivalent Graphs. Knotted Trivalent Graphs are Finitely Presented. VS, TS and TG Algebras. The Alexander Polynomial of a Knotted Trivalent Graph. Some Questions About Trinions. Finite Type Invariants The Envelope of a Finite Type Invariant. The Envelope of The Alexander Polynomial. Some Quotients of A. Associators with Frozen Feet. The HOMFLY Braidor Algebra. Coloured Chord Diagrams. Khovanov Homology Mutation Invariance of Khovanov Homology. Khovanov Homology of Alternating Tangles. Other The Existence of the Exponential Function. The Many Faces of Alexander.

In Preparation

The information below is preliminary and cannot be trusted! (v)

The Internal Kernel of the Alexander Polynomial

All that there is here comes from 06-1350/Class Notes for Tuesday October 24. Many further relevant facts are in arXiv:q-alg/9602014 by José M. Figueroa-O'Farrill, Takashi Kimura, Arkady Vaintrob and in arXiv:math.QA/0204346 by Jens Lieberum.

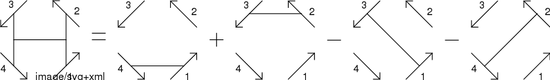
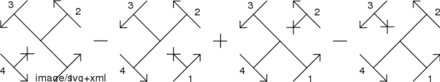
At the moment I know of just three relations in the internal kernel of the Alexander polynomial: the bubble relation, the H relation and the 4Y relation:

The Bubble Relation

The H Relation

The 4Y Relation

I have good reasons to suspect that there are further relations. But at the moment I don't know what they are, so below we will make do with what we have.

Alexander-Conway, Precisely

Let [math]\displaystyle{ C(z) }[/math] denote the Conway polynomial and [math]\displaystyle{ A(t) }[/math] denote the Alexander polynomial. By [Bar-Natan_Garoufalidis_96] we know that

[math]\displaystyle{ \frac{\hbar}{e^{\hbar/2}-e^{-\hbar/2}}C(e^{\hbar/2}-e^{-\hbar/2}) = \frac{\hbar}{e^{\hbar/2}-e^{-\hbar/2}}A(e^\hbar) }[/math]

is a canonical Vassiliev power series. Let [math]\displaystyle{ d }[/math] denote "half a bubble". The following theorem follows easily from the above canonicity statement and the fact that [math]\displaystyle{ W_C(d^{2n})=(-2\hbar^2)^n }[/math] (in shorter and less precise form, [math]\displaystyle{ \hbar=W_C(id/\sqrt2) }[/math]), where [math]\displaystyle{ W_C }[/math] is the weight system of the Alexander-Conway polynomial:

Theorem. Let [math]\displaystyle{ K }[/math] be a knot and let [math]\displaystyle{ Z(K) }[/math] be the Kontsevich integral of [math]\displaystyle{ K }[/math]. Then within the envelope of the Alexander-Conway polynomial,

[math]\displaystyle{ Z(K) = \frac{id/\sqrt2}{e^{id/2\sqrt2}-e^{-id/2\sqrt2}} C(e^{id/2\sqrt2}-e^{-id/2\sqrt2}) = \frac{id/\sqrt2}{e^{id/2\sqrt2}-e^{-id/2\sqrt2}} A(e^{id/\sqrt2}) }[/math].

References

[Bar-Natan_Garoufalidis_96] ^  Dror Bar-Natan and Stavros Garoufalidis, On the Melvin-Morton-Rozansky Conjecture, Inventiones Mathematicae 125 (1996) 103-133.