Fields 2009 Finite Type Invariants Proposal

This is a part of a proposal for a 2009 Knot Theory Program at the Fields Institute.

What is a Universal Finite Type Invariant?

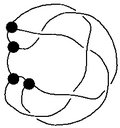
Given a graph $\Gamma$ ("the skeleton"), let ${\mathcal {K}}(\Gamma )$ denote the set of all "knottings" of $\Gamma$ - the set of all embeddings of $\Gamma$ into ${\mathbb {R} }^{3}$ considered modulo isotopy. So if $\Gamma$ is a circle, ${\mathcal {K}}(\Gamma )$ is an ordinary knot. If it is a union of circles, ${\mathcal {K}}(\Gamma )$ is a link, and if it is, say, a tetrahedron, ${\mathcal {K}}(\Gamma )$ will contain, for example, the knotted graph shown on the right.

A universal finite type invariant (using a rather broad definition) is a family of invariants $\{Z_{\Gamma }:{\mathcal {K}}(\Gamma )\to {\mathcal {A}}(\Gamma )\}$, one for each skeleton $\Gamma$, valued in some specific family of linear spaces ${\mathcal {A}}(\Gamma )$ one for each $\Gamma$. The spaces ${\mathcal {A}}(\Gamma )$ are themselves defined in terms of graphs along with some linear algebra, but since we don't need the details here, we won't show them. A certain "universality" property is expected to hold, but again, we don't need it right now so we won't discuss it.