10-1100/Homework Assignment 2

DBN: Classes: 2010-11: 10-1100/Navigation Additions to the MAT 1100 web site no longer count towards good deed points # Week of... Notes and Links 1 Sep 13 About This Class, Tuesday - Non Commutative Gaussian Elimination, Thursday 2 Sep 20 Tuesday - Homomorphisms and Normal Groups, Thursday - Isomorphism Theorems 3 Sep 27 Class Photo, HW1, HW1 solution 4 Oct 4 5 Oct 11 HW2, HW2 solution 6 Oct 18 7 Oct 25 Term Test 8 Nov 1 HW3, HW3 solution 9 Nov 8 Monday-Tuesday is Fall Break, One Theorem, Two Corollaries, Four Weeks 10 Nov 15 HW4, HW4 solution 11 Nov 22 12 Nov 29 HW5, HW5 solution 13 Dec 6 Boxing Day Handout, see also December 2010 Schedule. Some Class Notes F Dec 13 Final exam, Tuesday December 14 10-1, Bahen 6183 Register of Good Deeds Add your name / see who's in! 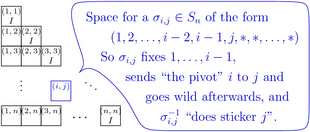See Non Commutative Gaussian Elimination

In Preparation

The information below is preliminary and cannot be trusted! (v)

This assignment is due at class time on Thursday, October 21, 2010.

Solve the following questions

1. (Selick)
   1. What it the least integer $n$ for which the symmetric group Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle S_n} contains an element of order 18?
   2. What is the maximal order of an element in $S_{52}$? (That is, of a shuffling of a deck of cards?)
2. (Selick) Let $H$ be a subgroup of index 2 in a group $G$. Show that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H} is normal in $G$.
3. Let $\sigma \in S_{20}$ be a permutation whose cycle decomposition consists of one 5-cycle, two 3-cycles, and one 2-cycle. What is the order of the centralizer $C_{S_{20}}(\sigma )$ of $\sigma$?
4. (Selick) Let Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G} be a group of odd order. Show that $x$ is not conjugate to $x^{-1}$ unless $x=e$.
5. (Dummit and Foote) Show that if $G/Z(G)$ is cyclic then $G$ is Abelian.
6. (Lang) Prove that if the group of automorphisms of a group Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G} is cyclic, then $G$ is Abelian.
7. (Lang)
   1. Let $G$ be a group and let $H$ be a subgroup of finite index. Prove that there is a normal subgroup Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle N} of $G$, contained in $H$, so that $(G:N)$ is also finite. (Hint: Let $(G:H)=n$ and find a morphism $G\to S_{n}$ whose kernel is contained in Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H} .)
   2. Let $G$ be a group and $H_{1}$ and $H_{2}$ be subgroups of $G$. Suppose Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (G:H_1)<\infty} and $(G:H_{2})<\infty$. Show that $(G:H_{1}\cap H_{2})<\infty$