14-1100/Homework Assignment 3

DBN: Classes: 2014-15: 14-1100/Navigation Welcome to Math 1100! (additions to this web site no longer count towards good deed points) # Week of... Notes and Links 1 Sep 8 About This Class; Monday - Non Commutative Gaussian Elimination; Thursday - the category of groups, automorphisms and conjugations, images and kernels. 2 Sep 15 Monday - coset spaces, isomorphism theorems; Thursday - simple groups, Jordan-Holder decomposition series. 3 Sep 22 Monday - alternating groups, group actions, The Simplicity of the Alternating Groups, HW1, HW 1 Solutions, Class Photo; Thursday - group actions, Orbit-Stabilizer Thm, Class Equation. 4 Sep 29 Monday - Cauchy's Thm, Sylow 1; Thursday - Sylow 2. 5 Oct 6 Monday - Sylow 3, semi-direct products, braids; HW2; HW 2 Solutions; Thursday - braids, groups of order 12, Braids 6 Oct 13 No class Monday (Thanksgiving); Thursday - groups of order 12 cont'd. 7 Oct 20 Term Test; Term Test Solutions on Monday, HW3; HW 3 Solutions; Thursday - solvable groups, rings: defn's & examples. 8 Oct 27 Monday - functors, Cayley-Hamilton Thm, ideals, iso thm 1; Thursday - iso thms 2-4, integral domains, maximal ideals, One Theorem, Three Corollaries, Five Weeks 9 Nov 3 Monday - prime ideals, primes & irreducibles, UFD's, Euc.Domain$\Rightarrow$PID, Thursday - Noetherian rings, PID$\Rightarrow$UFD, Euclidean Algorithm, modules: defn & examples, HW4, HW 4 Solutions 10 Nov 10 Monday - R is a PID iff R has a D-H norm, R-modules, direct sums, every f.g. module is given by a presentation matrix, Thursday - row & column reductions plus, existence part of Thm 1 in 1t3c5w handout. 11 Nov 17 Monday-Tuesday is UofT's Fall Break, HW5, Thursday - 1t3c5w handout cont'd, JCF Tricks & Programs handout 12 Nov 24 Monday - JCF Tricks & Programs cont'd, tensor products, Thursday - tensor products cont'd 13 Dec 1 End-of-Course Schedule; Monday - tensor products finale, extension/reduction of scalars, uniqueness part of Thm 1 in 1t3c5w, localization & fields of fractions; Wednesday is a "makeup Monday"!; Notes for Studying for the Final Exam Glossary of terms F Dec 15 The Final Exam Register of Good Deeds Add your name / see who's in! 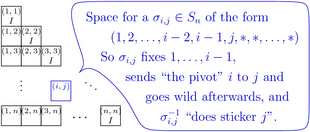See Non Commutative Gaussian Elimination

In Preparation

The information below is preliminary and cannot be trusted! (v)

This assignment is due at class time on Thursday, November 6, 2011.

Solve the following questions

Problem 1. (easy)

1. Prove that in any ring, $(-1)^{2}=1$.
2. Prove that even in a ring without a unit, $(-a)^{2}=a^{2}$.

(Feel free to do the second part first and then to substitute $a=1$).

Problem 2.

1. (Qualifying exam, April 2009) Prove that a finite integral domain is a field.
2. (Qualifying exam, September 2008) Prove that in a finite commutative ring, every prime ideal is maximal.

Problem 3. (Dummit and Foote) A ring $R$ is called a Boolean ring if $a^{2}=a$ for all $a\in R$.

1. Prove that every Boolean ring is commutative.
2. Prove that the only Boolean ring that is also an integral domain is ${\mathbb {Z} }/2$.

Problem 4. (bonus) Let $S$ be the ring of bounded sequences of real numbers with pointwise addition and multiplication, let $I$ be the ideal made of all sequences that are equal to $0$ except in at most finitely many places, and let $J$ be a maximal ideal in $S$ containing $I$.

1. Prove that $S/J\simeq {\mathbb {R} }$.
2. Denote by $\operatorname {Lim} _{J}$ the projection of $S$ to $S/J$ composed with the identification of the latter with ${\mathbb {R} }$, so that $\operatorname {Lim} _{J}:S\to {\mathbb {R} }$. Prove that for any scalar $c\in {\mathbb {R} }$ and any bounded sequences $(a_{n}),(b_{n})\in S$, we have that $\operatorname {Lim} _{J}(ca_{n})=c\cdot \operatorname {Lim} _{J}(a_{n})$, $\operatorname {Lim} _{J}(a_{n}+b_{n})=\operatorname {Lim} _{J}(a_{n})+\operatorname {Lim} _{J}(b_{n})$, and $\operatorname {Lim} _{J}(a_{n}b_{n})=(\operatorname {Lim} _{J}(a_{n}))(\operatorname {Lim} _{J}(b_{n}))$. (Easy, no bonuses for this part).
3. Prove that if $\lim a_{n}=\alpha$ in the ordinary sense of limits of sequences, then $\operatorname {Lim} _{J}a_{n}=\alpha$.
4. Is there a $J$ for which $\operatorname {Lim} _{J}$ is also translation invariant, namely such that $\operatorname {Lim} _{J}(a_{n})=\operatorname {Lim} _{J}(a_{n+1})$? (Again, easy).

Warning. The right order for solving these questions is not necessarily the order in which they are presented.

Opinion. The sum total of all that is that using the axiom of choice you can construct things that are both too good to be true and not really useful anyway. Blame the axiom of choice, don't blame me.