14-1100/Homework Assignment 2

DBN: Classes: 2014-15: 14-1100/Navigation Welcome to Math 1100! (additions to this web site no longer count towards good deed points) # Week of... Notes and Links 1 Sep 8 About This Class; Monday - Non Commutative Gaussian Elimination; Thursday - the category of groups, automorphisms and conjugations, images and kernels. 2 Sep 15 Monday - coset spaces, isomorphism theorems; Thursday - simple groups, Jordan-Holder decomposition series. 3 Sep 22 Monday - alternating groups, group actions, The Simplicity of the Alternating Groups, HW1, HW 1 Solutions, Class Photo; Thursday - group actions, Orbit-Stabilizer Thm, Class Equation. 4 Sep 29 Monday - Cauchy's Thm, Sylow 1; Thursday - Sylow 2. 5 Oct 6 Monday - Sylow 3, semi-direct products, braids; HW2; HW 2 Solutions; Thursday - braids, groups of order 12, Braids 6 Oct 13 No class Monday (Thanksgiving); Thursday - groups of order 12 cont'd. 7 Oct 20 Term Test; Term Test Solutions on Monday, HW3; HW 3 Solutions; Thursday - solvable groups, rings: defn's & examples. 8 Oct 27 Monday - functors, Cayley-Hamilton Thm, ideals, iso thm 1; Thursday - iso thms 2-4, integral domains, maximal ideals, One Theorem, Three Corollaries, Five Weeks 9 Nov 3 Monday - prime ideals, primes & irreducibles, UFD's, Euc.Domain$\Rightarrow$PID, Thursday - Noetherian rings, PID$\Rightarrow$UFD, Euclidean Algorithm, modules: defn & examples, HW4, HW 4 Solutions 10 Nov 10 Monday - R is a PID iff R has a D-H norm, R-modules, direct sums, every f.g. module is given by a presentation matrix, Thursday - row & column reductions plus, existence part of Thm 1 in 1t3c5w handout. 11 Nov 17 Monday-Tuesday is UofT's Fall Break, HW5, Thursday - 1t3c5w handout cont'd, JCF Tricks & Programs handout 12 Nov 24 Monday - JCF Tricks & Programs cont'd, tensor products, Thursday - tensor products cont'd 13 Dec 1 End-of-Course Schedule; Monday - tensor products finale, extension/reduction of scalars, uniqueness part of Thm 1 in 1t3c5w, localization & fields of fractions; Wednesday is a "makeup Monday"!; Notes for Studying for the Final Exam Glossary of terms F Dec 15 The Final Exam Register of Good Deeds Add your name / see who's in! 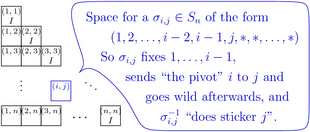See Non Commutative Gaussian Elimination

In Preparation

The information below is preliminary and cannot be trusted! (v)

This assignment is due at class time on Thursday, October 20, 2010.

Solve the following questions

1. (Selick)
   1. What it the least integer $n$ for which the symmetric group $S_{n}$ contains an element of order 18?
   2. What is the maximal order of an element in $S_{26}$? (That is, of a shuffling of the red cards within a deck of cards?)
2. (Selick) Let $H$ be a subgroup of index 2 in a group $G$. Show that $H$ is normal in $G$.
3. Let $\sigma \in S_{20}$ be a permutation whose cycle decomposition consists of one 5-cycle, two 3-cycles, and one 2-cycle. What is the order of the centralizer $C_{S_{20}}(\sigma )$ of $\sigma$?
4. (Selick) Let $G$ be a group of odd order. Show that $x$ is not conjugate to $x^{-1}$ unless $x=e$.
5. (Dummit and Foote) Show that if $G/Z(G)$ is cyclic then $G$ is Abelian.
6. (Lang) Prove that if the group of automorphisms of a group $G$ is cyclic, then $G$ is Abelian.
7. (Lang)
   1. Let $G$ be a group and let $H$ be a subgroup of finite index. Prove that there is a normal subgroup $N$ of $G$, contained in $H$, so that $(G:N)$ is also finite. (Hint: Let $(G:H)=n$ and find a morphism $G\to S_{n}$ whose kernel is contained in $H$.)
   2. Let $G$ be a group and $H_{1}$ and $H_{2}$ be subgroups of $G$. Suppose $(G:H_{1})<\infty$ and $(G:H_{2})<\infty$. Show that $(G:H_{1}\cap H_{2})<\infty$

Problem 1. (Selick) Show that any group of order 56 has a normal Sylow-$p$ subgroup, for some prime $p$ dividing 56.

Problem 2. (Qualifying exam, May 1997) Let $S_{5}$ act on $({\mathbb {Z} /5})^{5}$ by permuting the factors, and let $G$ be the semi-direct product of $S_{5}$ and $({\mathbb {Z} /5})^{5}$.

1. What is the order of $G$?
2. How many Sylow-5 subgroups does $G$ have? Write down one of them.

Problem 3. (Selick) Show that the group $Q$ of unit quaternions ($\{\pm 1,\pm i,\pm j,\pm k\}$, subject to $i^{2}=j^{2}=k^{2}=-1\in Z(Q)$ and $ij=k$) is not a semi-direct product of two of its proper subgroups.

Problem 4. (Qualifying exam, September 2008) Let $G$ be a finite group and $p$ be a prime. Show that if $H$ is a $p$-subgroup of $G$, then $(N_{G}(H):H)$ is congruent to $(G:H)$ mod $p$. You may wish to study the action of $H$ on $G/H$ by multiplication on the left.