10-1100/Homework Assignment 4

DBN: Classes: 2010-11: 10-1100/Navigation Additions to the MAT 1100 web site no longer count towards good deed points # Week of... Notes and Links 1 Sep 13 About This Class, Tuesday - Non Commutative Gaussian Elimination, Thursday 2 Sep 20 Tuesday - Homomorphisms and Normal Groups, Thursday - Isomorphism Theorems 3 Sep 27 Class Photo, HW1, HW1 solution 4 Oct 4 5 Oct 11 HW2, HW2 solution 6 Oct 18 7 Oct 25 Term Test 8 Nov 1 HW3, HW3 solution 9 Nov 8 Monday-Tuesday is Fall Break, One Theorem, Two Corollaries, Four Weeks 10 Nov 15 HW4, HW4 solution 11 Nov 22 12 Nov 29 HW5, HW5 solution 13 Dec 6 Boxing Day Handout, see also December 2010 Schedule. Some Class Notes F Dec 13 Final exam, Tuesday December 14 10-1, Bahen 6183 Register of Good Deeds Add your name / see who's in! 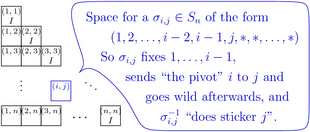See Non Commutative Gaussian Elimination

This assignment is due at class time on Tuesday, November 30, 2010.

Solve the following questions

Problem 1. Prove that a ring [math]\displaystyle{ R }[/math] is a PID iff it is a UFD in which [math]\displaystyle{ \gcd(a,b)\in\langle a, b\rangle }[/math] for every non-zero [math]\displaystyle{ a,b\in R }[/math].

Problem 2. (Selick) In a ring [math]\displaystyle{ R }[/math], and element [math]\displaystyle{ x }[/math] is called "nilpotent" if for some positive [math]\displaystyle{ n }[/math], [math]\displaystyle{ x^n=0 }[/math]. Let [math]\displaystyle{ \eta(R) }[/math] be the set of all nilpotent elements of [math]\displaystyle{ R }[/math].

1. Prove that if [math]\displaystyle{ R }[/math] is commutative then [math]\displaystyle{ \eta(R) }[/math] is an ideal.
2. Give an example of a non-commutative ring [math]\displaystyle{ R }[/math] in which [math]\displaystyle{ \eta(R) }[/math] is not an ideal.

Problem 3. (comprehensive exam, 2009) Let [math]\displaystyle{ A }[/math] be a commutative ring. Show that a polynomial [math]\displaystyle{ f\in A[x] }[/math] is invertible in [math]\displaystyle{ A[x] }[/math] iff its constant term is invertible in [math]\displaystyle{ A }[/math] and the rest of its coefficients are nilpotent.

Problem 4. (Lang) Show that the ring [math]\displaystyle{ {\mathbb Z}[i]=\{a+ib\colon a,b\in{\mathbb Z}\}\subset{\mathbb C} }[/math] is a PID and hence a UFD. What are the units of that ring?

Problem 5. (Dummit and Foote) In [math]\displaystyle{ {\mathbb Z}[i] }[/math], find the greatest common divisor of [math]\displaystyle{ 85 }[/math] and [math]\displaystyle{ 1+13i }[/math], and express it as a linear combination of these two elements.

---

Problem 6. (Withdrawn, do not submit) Show that the quotient ring [math]\displaystyle{ {\mathbb Q}[x,y]/\langle x^2+y^2-1\rangle }[/math] is not a UFD.