User:Shawkm/06-1350-HW4

The Generators

Our generators are $T$, $R$, $\Phi$ and $B^{\pm }$:

Picture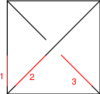
Generator	$T$	$R$	$\Phi$	$B^{+}$	$B^{-}$
Perturbation	$t$	$r$	$\varphi$	$b^{+}$	$b^{-}$

The Relations

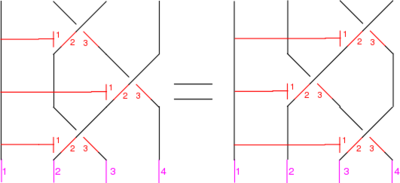
The Reidemeister Move R3

The picture (with three sides of the shielding removed) is

In formulas, this is

$(1230)^{\star }B^{+}(1213)^{\star }B^{+}(1023)^{\star }B^{+}=(1123)^{\star }B^{+}(1203)^{\star }B^{+}(1231)^{\star }B^{+}$.

Linearized and written in functional form, this becomes

$\rho _{3}(x_{1},x_{2},x_{3},x_{4})=$	$b^{+}(x_{1},x_{2},x_{3})+b^{+}(x_{1}+x_{3},x_{2},x_{4})+b^{+}(x_{1},x_{3},x_{4})$
	$-b^{+}(x_{1}+x_{2},x_{3},x_{4})-b^{+}(x_{1},x_{2},x_{4})-b^{+}(x_{1}+x_{4},x_{2},x_{3}).$

The Syzygies

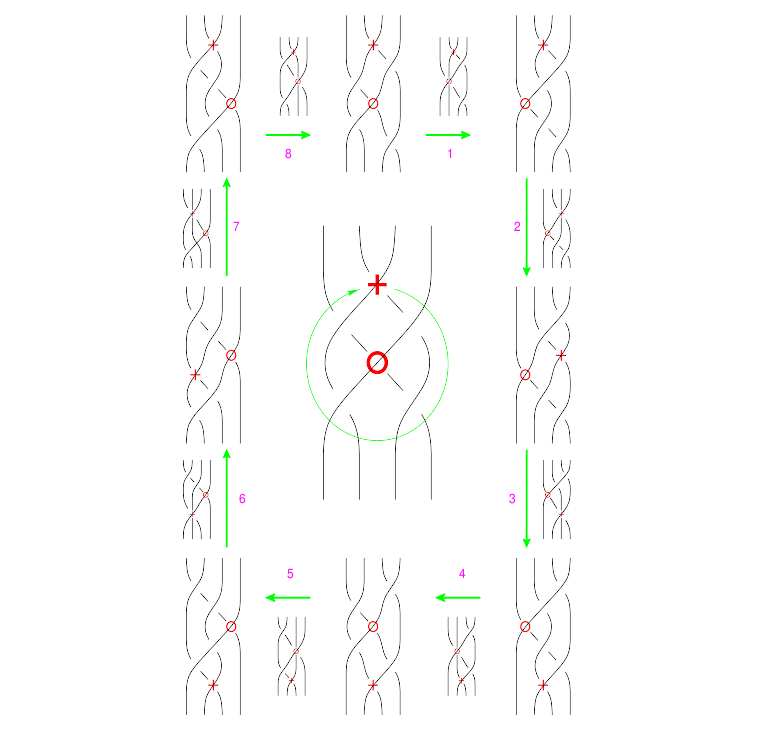
The "B around B" Syzygy

The picture, with all shielding removed, is

(Drawn with Inkscape) (note that lower quality pictures are also acceptable)

The functional form of this syzygy is

$BB(x_{1},x_{2},x_{3},x_{4},x_{5})=$	$\rho _{3}(x_{1},x_{2},x_{3},x_{5})+\rho _{3}(x_{1}+x_{5},x_{2},x_{3},x_{4})-\rho _{3}(x_{1}+x_{2},x_{3},x_{4},x_{5})$
	$-\rho _{3}(x_{1},x_{2},x_{4},x_{5})-\rho _{3}(x_{1}+x_{4},x_{2},x_{3},x_{5})-\rho _{3}(x_{1},x_{2},x_{3},x_{4})$
	$+\rho _{3}(x_{1},x_{3},x_{4},x_{5})+\rho _{3}(x_{1}+x_{3},x_{2},x_{4},x_{5}).$

A Mathematica Verification

The following simulated Mathematica session proves that for our single relation and single syzygy, $d^{2}=0$. Copy paste it into a live Mathematica session to see that it's right!

In[1]:=

d1 = { rho3[x1_, x2_, x3_, x4_] :> bp[x1, x2, x3] + bp[x1 + x3, x2, x4] + bp[x1, x3, x4] - bp[x1 + x2, x3, x4] - bp[x1, x2, x4] - bp[x1 + x4, x2, x3] }; d2 = { BAroundB[x1_, x2_, x3_, x4_, x5_] :> rho3[x1, x2, x3, x5] + rho3[x1 + x5, x2, x3, x4] - rho3[x1 + x2, x3, x4, x5] - rho3[x1, x2, x4, x5] - rho3[x1 + x4, x2, x3, x5] - rho3[x1, x2, x3, x4] + rho3[x1, x3, x4, x5] + rho3[x1 + x3, x2, x4, x5] };

In[3]:=	BAroundB[x1, x2, x3, x4, x5] /. d2
Out[3]=	- rho3[x1, x2, x3, x4] + rho3[x1, x2, x3, x5] - rho3[x1, x2, x4, x5] + rho3[x1, x3, x4, x5] - rho3[x1 + x2, x3, x4, x5] + rho3[x1 + x3, x2, x4, x5] - rho3[x1 + x4, x2, x3, x5] + rho3[x1 + x5, x2, x3, x4]

In[4]:=	BAroundB[x1, x2, x3, x4, x5] /. d2 /. d1
Out[4]=	0

Here Goes Something New(?)

The first thing to notice is that the relation $\rho _{3}$ holds for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b^+ <\math> and <math> b^- <\math> so we have another version: {| align=center |- |<math>\rho_3(x_1, x_2, x_3, x_4) = } |$b^{-}(x_{1},x_{2},x_{3})+b^{-}(x_{1}+x_{3},x_{2},x_{4})+b^{-}(x_{1},x_{3},x_{4})$ |- | |$-b^{-}(x_{1}+x_{2},x_{3},x_{4})-b^{-}(x_{1},x_{2},x_{4})-b^{-}(x_{1}+x_{4},x_{2},x_{3}).$ |}

This is probably a completely trivial remark, and it is also trivial to see that mathematica will deal with this in the same way as for Failed to parse (unknown function "\math"): {\displaystyle b^+ <\math> so I won't verify that <math> d^2 =0 <\math>.}