WClips/Navigation

Back to WKO.
Next Meeting. Wednesday March 7, 2012, 12-2, at Bahen 4010. We'll complete the discussion of the expansion for w-knots and move on to Section 3.5, "Jacobi Diagrams, Trees and Wheels".

Announcements. small circle, wide circle, UofT, LDT Blog (also here).

Resources. How to use this site, Dror's notebook, blackboard shots.

The wClips

http://katlas.math.toronto.edu/drorbn/dbnvp/dbnvp.png

Date	Links
11/01/12	120111-1: Introduction.  120111-2: Section 2.1 - v-Braids.
18/01/12	120118-1: An introduction to this web site.  120118-2: Section 2.2 - w-Braids by generators and relations and as flying rings.  120118-3: Section 2.2 - w-Braids - other drawing conventions, "wens".
25/01/12	120125-1: Section 2.2.3 - basis conjugating automorphisms of $F_{n}$.  120125-2: A very quick introduction to finite type invariants in the "u" case.
01/02/12	120201: Section 2.3 - finite type invariants of v- and w-braids, arrow diagrams, 6T, TC and 4T relations, expansions / universal finite type invariants.
08/02/12	120208: Review of u,v, and w braids and of Section 2.3.
08/02/15	120215: Section 2.5 - mostly compatibilities of $Z^{w}$, also injectivity and uniqueness of $Z^{w}$.
08/02/22	120222: Section 2.5.5, $\alpha :{\mathcal {A}}^{u}\to {\mathcal {A}}^{v}$, and Section 3.1 (partially), the definition of v- and w-knots.
08/02/29	120229: Sections 3.1-3.4: v-Knots and w-Knots: Definitions, framings, finite type invariants, dimensions, and the expansion in the w case.
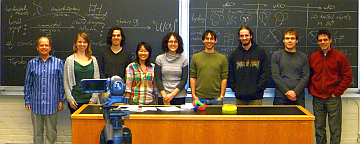
Group photo on January 11, 2012