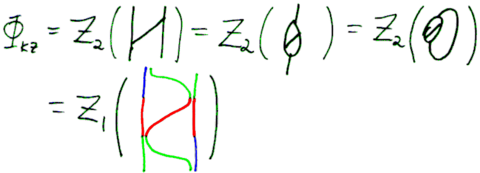The Kontsevich Integral for Knotted Trivalent Graphs: Difference between revisions
No edit summary |
|||
| (3 intermediate revisions by the same user not shown) | |||
| Line 23: | Line 23: | ||
==Step 2 - The Renormalized Kontsevich Integral== |
==Step 2 - The Renormalized Kontsevich Integral== |
||
[[Image:07-1352 Renormalizing the Kontsevich Integral.png| |
[[Image:07-1352 Renormalizing the Kontsevich Integral.png|480px|center]] |
||
After choosing a scale <math>\mu</math> and using ideas from renormalization theory as practiced by quantum field theorists, we define the "renormalized Kontsevich integral" <math>Z_2</math> of a knotted trivalent graph or a slice thereof as summarized by the picture above. |
After choosing a scale <math>\mu</math> and an infinitesimal <math>\epsilon</math> and using ideas from renormalization theory as practiced by quantum field theorists, we define the "renormalized Kontsevich integral" <math>Z_2</math> of a knotted trivalent graph or a slice thereof as summarized by the picture above. |
||
===The Good=== |
===The Good=== |
||
| Line 33: | Line 33: | ||
* It is invariant under rigid motions of critical points and trivalent vertices. |
* It is invariant under rigid motions of critical points and trivalent vertices. |
||
* It has sensible behaviour under vertical connect sum, delete and unzip operations. |
* It has sensible behaviour under vertical connect sum, delete and unzip operations. |
||
* It has a sensible behaviour under the changing of the scale <math>\mu</math> |
* It has a sensible behaviour under the changing of the scale <math>\mu</math>, as follows from: |
||
'''Claim.''' Let <math>w_1,\ldots,w_n</math> be distinct complex numbers and let <math>\beta</math> be another complex number. Let <math>B</math> be the (<math>n</math>-strand) braid "rescaling braid" which is the image of the map <math>[0,1]\to[0,1]\times{\mathbb C}^n</math> defined by <math>t\mapsto(t, e^{\beta t}w_1,\ldots,e^{\beta t}w_n)</math>. Finally, in <math>{\mathcal A}(\uparrow_n)</math> let <math>c</math> be the "sum of all horizontal chords"; <math>c=\sum_{1\leq i<j\leq n}t^{ij}</math>. Then up to normalization factors which we are getting right with probability <math>\frac{1}{2^k}</math> for some small <math>k\in{\mathbb N}</math>, |
|||
{{Equation*|<math>Z(B)=\exp\frac{\beta c}{2\pi i}\in{\mathcal A}(\uparrow_n)</math>.}} |
|||
===The Bad=== |
===The Bad=== |
||
| Line 41: | Line 45: | ||
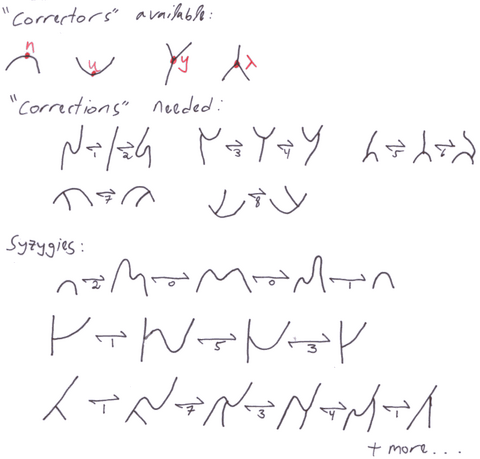
==Step 3 - The Corrected Kontsevich Integral== |
==Step 3 - The Corrected Kontsevich Integral== |
||
[[Image:07-1352 Correctors Corrections Syzygies.png|480px|center]] |
|||
| ⚫ | By correcting caps and cups with factors <math>n</math> and <math>u</math>, and the two types of trivalent vertices with factors <math>y</math> and <math>\lambda</math>, we define the "corrected Kontsevich integral" <math>Z=Z_3</math> of a knotted trivalent graph or a slice thereof. It has all the good properties we can wish for. |
||
| ⚫ | By correcting caps and cups with factors <math>n</math> and <math>u</math>, and the two types of trivalent vertices with factors <math>y</math> and <math>\lambda</math>, we define the "corrected Kontsevich integral" <math>Z=Z_3</math> of a knotted trivalent graph or a slice thereof. It has all the good properties we can wish for. '''The details still need to be worked out here!''' |
||
==Aside - The Relationship with Drinfel'd's KZ Associator== |
==Aside - The Relationship with Drinfel'd's KZ Associator== |
||
The Drinfel'd KZ associator is reproduced already by <math>Z_2</math> |
The Drinfel'd KZ associator <math>\Phi_{KZ}</math> is reproduced already by <math>Z_2</math>: |
||
[[Image:07-1352 Normalizing PhiKZ.png|480px|center]] |
|||
Latest revision as of 15:22, 6 March 2007
|
The information below is preliminary and cannot be trusted! (v)
Abstract
We construct a (very) well-behaved invariant of knotted trivalent graphs using only the Kontsevich integral, in three steps.
Step 1 - The Naive Kontsevich Integral
We define the "naive Kontsevich integral" of a knotted trivalent graph or a slice thereof as in the "standard" picture above, except generalized to graphs in the obvious manner.
The Good
- It has a factorization property.
- For the "braid-like" factors, it has invariance under horizontal deformations.
- It is morally a universal finite type invariant.
The Bad
- It is divergent.
Step 2 - The Renormalized Kontsevich Integral
After choosing a scale and an infinitesimal and using ideas from renormalization theory as practiced by quantum field theorists, we define the "renormalized Kontsevich integral" of a knotted trivalent graph or a slice thereof as summarized by the picture above.
The Good
- It retains all the good properties of the naive Kontsevich integral.
- It is convergent.
- It is invariant under rigid motions of critical points and trivalent vertices.
- It has sensible behaviour under vertical connect sum, delete and unzip operations.
- It has a sensible behaviour under the changing of the scale , as follows from:
Claim. Let be distinct complex numbers and let be another complex number. Let be the (-strand) braid "rescaling braid" which is the image of the map defined by . Finally, in let be the "sum of all horizontal chords"; . Then up to normalization factors which we are getting right with probability for some small ,
The Bad
- It is not invariant under non-rigid motions of vertices and critical points.
Step 3 - The Corrected Kontsevich Integral
By correcting caps and cups with factors and , and the two types of trivalent vertices with factors and , we define the "corrected Kontsevich integral" of a knotted trivalent graph or a slice thereof. It has all the good properties we can wish for. The details still need to be worked out here!
Aside - The Relationship with Drinfel'd's KZ Associator
The Drinfel'd KZ associator is reproduced already by :









![{\displaystyle [0,1]\to [0,1]\times {\mathbb {C} }^{n}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b9371dd13f8e28e88a417d0bfcaaa871fb7f84c8)