Mutation Invariance of Khovanov Homology: Difference between revisions
No edit summary |
|||
| (42 intermediate revisions by 6 users not shown) | |||
| Line 1: | Line 1: | ||
{{Paperlets Navigation}} |
|||
| ⚫ | |||
[[Image:TheInsideStory.png|250px|right]] |
|||
[[Image:ReEmbeddingAndMutation.png|right|250px]] |
|||
| ⚫ | |||
==History And Summary== |
==History And Summary== |
||
I have presented an almost-proof of invariance of Khovanov homology under knot mutation in a conference in Snowbird, Utah in June 2005 (see the handouts from that talk [http://www.math.toronto.edu/~drorbn/Talks/Utah-0506/index.html here]). At the time it was clear to me that the very end of the proof |
I have presented an almost-proof of invariance of Khovanov homology under knot mutation in a conference in Snowbird, Utah in June 2005 (see the handouts from that talk [http://www.math.toronto.edu/~drorbn/Talks/Utah-0506/index.html here]). At the time it was clear to me that the very end of the proof was a bit fishy, and an example by Jake Rasmussen made it look even fishier. |
||
Yet the proof seemed to have all the right ingredients in it (for example, it was restricted to the case of knots; indeed, mutation invariance of Khovanov homology for links is known to fail). So I was quite sure the missing bit will be completed quickly. A short time later I (thought I) was able to complete the proof, and for a few months I believed my proof was right, though I did not write it up so I was never confident. |
Yet the proof seemed to have all the right ingredients in it (for example, it was restricted to the case of knots; indeed, mutation invariance of Khovanov homology for links is known to fail). So I was quite sure the missing bit will be completed quickly. A short time later I (thought I) was able to complete the proof, and for a few months I believed my proof was right, though I did not write it up so I was never confident. |
||
| Line 10: | Line 14: | ||
Yet not all is lost: |
Yet not all is lost: |
||
* The proof remains "almost true" and still seems to have (at least |
* The proof remains "almost true" and still seems to have (at least many of) the right ingredients. Thus somebody may well be able to complete it one day. |
||
* The parts of the proof that still hold suggest ways to narrow down the search for counterexamples for mutation invariance. Thus with the proper software there is some real hope that a counterexample will be found. |
* The parts of the proof that still hold suggest ways to narrow down the search for counterexamples for mutation invariance. Thus with the proper software there is some real hope that a counterexample will be found. |
||
* In an effort to save the proof I realized that mutation invariance is implied by a much broader and more conceptual suggested property of Khovanov homology, the property of "re- |
* In an effort to save the proof I realized that mutation invariance is implied by a much broader and more conceptual suggested property of Khovanov homology, the property of "re-embedding universality". The difficulty in proving re-embedding universality is the same as the difficulty in proving mutation invariance, but the benefit, if proven, seems greater. I hope people will put some effort into understanding re-embedding universality. |
||
==What's Mutation?== |
==What's Mutation?== |
||
| Line 20: | Line 24: | ||
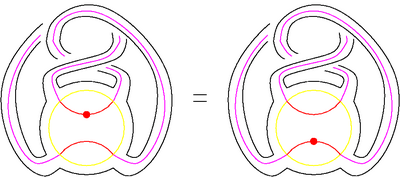
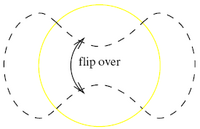
A ''mutation'' is the process of marking a circle on a knot or a link diagram which intersects it at precisely four points, and flipping its inside (the ''inside tangle''), yet not its outside (the ''outside tangle''), around a horizontal axis as illustrated on the right. (Other types of mutation are topologically equivalent to this one). Mutation does not change the overall connectivity of the knot/link in question. Thus a knot stays a knot and a link stays a link. |
A ''mutation'' is the process of marking a circle on a knot or a link diagram which intersects it at precisely four points, and flipping its inside (the ''inside tangle''), yet not its outside (the ''outside tangle''), around a horizontal axis as illustrated on the right. (Other types of mutation are topologically equivalent to this one). Mutation does not change the overall connectivity of the knot/link in question. Thus a knot stays a knot and a link stays a link. |
||
It is well known that the Jones polynomial |
It is well known that the Jones polynomial, as well as the Alexander, HOMFLYPT, and coloured Jones polynomials, are invariant under knot mutation. There are easy examples to show that Khovanov homology is not invariant under link mutation, but it is still unknown whether or not Khovanov homology is invariant under knot mutation. |
||
==The Almost Proof== |
==The Almost Proof== |
||
| Line 40: | Line 44: | ||
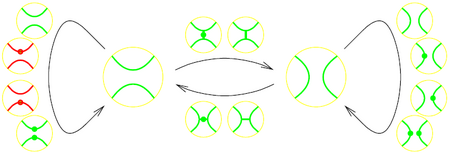
[[Image:TheInsideStory.png|thumb|450px|center|The "inside" category has two objects and 12 morphisms]] |
[[Image:TheInsideStory.png|thumb|450px|center|The "inside" category has two objects and 12 morphisms]] |
||
The construction of <math>\operatorname{Kh}(T)</math> and the process of "delooping" are clearly equivariant under horizontal (or vertical, for that matter) flips and so <math>\operatorname{Kh}(T_2)</math> is obtained from <math>\operatorname{Kh}(T_1)</math> simply by flipping all objects and morphisms in sight. But these all fall within the category in the picture above, and all two of the objects and 10 of the 12 morphisms are clearly invariant under flips. The remaining two morphisms are drawn in red in the above picture. The only difference between <math>\operatorname{Kh}(T_1)</math> and <math>\operatorname{Kh}(T_2)</math> is that every |
The construction of <math>\operatorname{Kh}(T)</math> and the process of "delooping" are clearly equivariant under horizontal (or vertical, for that matter) flips and so <math>\operatorname{Kh}(T_2)</math> is obtained from <math>\operatorname{Kh}(T_1)</math> simply by flipping all objects and morphisms in sight. But these all fall within the category in the picture above, and all two of the objects and 10 of the 12 morphisms are clearly invariant under flips. The remaining two morphisms are drawn in red in the above picture. The only difference between <math>\operatorname{Kh}(T_1)</math> and <math>\operatorname{Kh}(T_2)</math> is that every occurrence of a "red" morphism in <math>\operatorname{Kh}(T_1)</math> becomes the "other red" in <math>\operatorname{Kh}(T_2)</math>. |
||
===The Outside Story=== |
===The Outside Story=== |
||
| Line 47: | Line 51: | ||
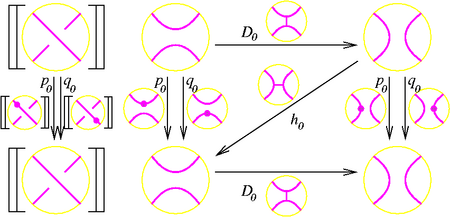
[[Image:TheOutsideStory.png|thumb|450px|center|A "dot" can be moved across a crossing]] |
[[Image:TheOutsideStory.png|thumb|450px|center|A "dot" can be moved across a crossing]] |
||
This picture shows (by displaying an explicit homotopy) that the two endomorphisms <math> |
This picture shows (by displaying an explicit homotopy) that the two endomorphisms <math>p_0</math> and <math>q_0</math> of the complex of a single crossing, obtained by a horizontal composition with a dotted curtain either on the upper left or on the lower right are homotopic. This can be iterated to "drag" dots across crossings and thus, as the connectivity of strands in <math>S</math> is as in '''Figure 1''', the "dot" in any one "red" morphism in <math>\operatorname{Kh}(T_1)</math> can be pulled around the outside so as to turn it into the other "red" morphism. |
||
(Notice: this is where the given connectivity for the outside is used, and it is at this point that our almost proof fails entirely for links). |
|||
| ⚫ | |||
| ⚫ | |||
===The Inside Meets the Outside=== |
===The Inside Meets the Outside=== |
||
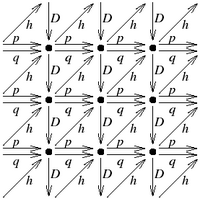
[[Image:TheDoubleComplexForMutation.png|thumb|200px|right|The double complex for mutation invariance.]] |
[[Image:TheDoubleComplexForMutation.png|thumb|200px|right|The double complex for mutation invariance.]] |
||
In summary, <math>\operatorname{Kh}(K_1)</math> and <math>\operatorname{Kh}(K_2)</math> are the homologies (or just the homotopy classes) of two double complexes that share a vertical differential <math>D</math> but may have different horizontal differentials, <math>p</math> and <math>q</math>, and those two horizontal differentials are homotopic relative to the vertical differential using a homotopy <math>h</math>. |
|||
Note that <math>p</math> and <math>q</math> here are not quite the same as <math>p_0</math> and <math>q_0</math> of the previous section - <math>p_0</math> and <math>q_0</math> were just single "red" parts of the <math>p</math>'s and <math>q</math>'s here, and dots were just being "transported" across a single crossing. <math>p</math> and <math>q</math> may differ by having ''many'' red parts, and the dots on these red parts may need transportation across ''many'' crossings. So <math>h</math> is a (possibly) complex assembly of many versions of <math>h_0</math> "installed" at (possibly) many locations on the outside tangle and within (possibly) many entries in the double complex. |
|||
==It's Failure== |
|||
One would hope that with such a close relationship between the complexes for <math>\operatorname{Kh}(K_1)</math> and <math>\operatorname{Kh}(K_2)</math> it should be easy to prove that the two homologies are equal. |
|||
| ⚫ | |||
==Failure of the "Almost Proof"== |
|||
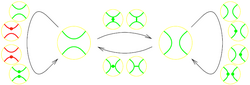
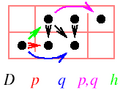
[[Image:RasmussensExampleDoubleComplex.png|thumb|left|120px|Rasmussen's example]] |
|||
Unfortunately it is simply not true that homotopic horizontal differentials in a double complex lead to equal overall homology. A nice counterexample was given already in Utah by Jake Rasmussen, and is reproduced on the left here (dots stand for vectors and the maps are presented by colour-coded arrows). |
|||
It is not difficult to show that if in addition <math>h</math> commutes with <math>p</math> and <math>q</math> then <math>\exp(h)</math> is an isomorphism between the two double complexes. For a while I believed I could show that in our case indeed <math>h</math> commutes with <math>p</math> and <math>q</math> and the problem is resolved. But in December 2005 Stephan Wehrli asked for the details, and it turned out I didn't have them (no counterexample yet, either). At this time neither I nor he know how to revive the proof. |
|||
| ⚫ | |||
We will say that a knot invariant has "re-embedding universality" if whenever a knot is drawn on a surface and the surface is embedded in space, one can recover the invariant of the "pushforward" knot in space from the invariant of that knot on the surface and from information about the embedding of the surface in space. |
|||
After saying the same thing again in a few more words, we'll state the obvious fact that the Jones polynomial has re-embedding universality and ask if Khovanov homology has that too. If it does, mutation invariance holds (for knots) for Khovanov homology. That would be a lovely corollary of re-embedding universality. Yet IMHO, re-embedding universality is such a lovely abstract property of the Jones polynomial that resolving whether or not it holds for Khovanov homology is much more significant than deciding the issue of mutation invariance. |
|||
===Re-Embedding Universality in More words=== |
|||
Suppose a knot (or a link) <math>K</math> is drawn on a surface <math>\Sigma</math> (i.e., embedded in <math>\Sigma\times I</math>, via a map <math>\gamma:S\to\Sigma\times I</math>, where <math>S</math> is a circle or a union of circles), and suppose that in turn, <math>\Sigma</math> is embedded in <math>{\mathbb R}^3</math> via <math>\sigma:\Sigma\to{\mathbb R}^3</math>. Extend <math>\sigma</math> to a homeomorphism of <math>\Sigma\times I</math> with a tubular neighbourhood of <math>\sigma(\Sigma)</math> and abuse the notation a bit by denoting the resulting extension again by <math>\sigma</math>. We can now regard <math>K</math> also as a knot in <math>{\mathbb R}^3</math> which perhaps is better denoted <math>\sigma\circ\gamma</math>. |
|||
Suppose <math>I</math> is some knot/link invariant which is defined for knots drawn in the plane (i.e., knots in <math>{\mathbb R}^3</math>) as well as for knots/links drawn on other surfaces. |
|||
We will say that <math>I</math> has "re-embedding universality" if <math>I(\gamma)</math> and <math>\sigma</math> together determine <math>I(\sigma\circ\gamma)</math>. This is a natural "good behaviour" property for <math>I</math> which somehow says that there is no loss of information in computing <math>I</math> for knots that are embedded "in steps". |
|||
===The Jones Polynomial has Re-Embedding Universality=== |
|||
There's nothing to say here, really. Consider the Jones polynomial for surface knots using Kauffman bracket skein modules (so that it is valued in <math>{\mathbb Z}[q,q^{-1}]</math>-linear combinations of "smoothings" on <math>\Sigma</math>. Once <math>\sigma</math> is given, each smoothing on <math>\Sigma</math> becomes a knot in <math>{\mathbb R}^3</math> which has its own Jones polynomial, and the appropriate linear combination of these Jones polynomials is the Jones polynomial of <math>\sigma\circ\gamma</math>; this is merely the fact that in resolving crossings to smoothings, one may first resolve the crossings "on <math>\Sigma</math>" and then the crossings induced from the projection of <math>\Sigma</math> to the plane. |
|||
In fairness, a minor complication does arise - the Jones polynomial is defined for oriented knots/links, while smoothings are unoriented. But orientation issues only amount to signs and overall powers of <math>q</math>. So you may either ignore those, or do the right thing, work a bit harder, and find a way to incorporate a bit of extra orientation information into the definition of <math>J</math> for surface knots. Most likely it will be enough to declare that the "enhanced Jones" of <math>\gamma:S\to\Sigma\times I</math> is the "old Jones" of <math>\gamma</math> along with <math>\gamma_\star H_1(S)\subset H_1(\Sigma)</math>. I will ignore this issue below. |
|||
===Khovanov Homology and Re-Embedding Universality=== |
|||
Does Khovanov homology enjoy re-embedding universality? |
|||
The complete locality of the constructions in {{ref|Bar-Natan}} allows them to be easily generalized to knots on surfaces. In short, Khovanov homology for knots on <math>\Sigma</math> takes values in formal complexes in the category <math>\operatorname{Cob}(\Sigma)</math> whose objects are smoothings in <math>\Sigma</math> and whose morphisms are cobordisms in <math>\Sigma\times I</math> between such smoothings. For a given knot <math>K</math>, call the resulting complex <math>\operatorname{Kh}(K)</math>. |
|||
Given an embedding <math>\sigma</math> of <math>\Sigma</math> in <math>{\mathbb R}^3</math> there is a natural functor <math>\operatorname{Kh}_\sigma</math> taking <math>\operatorname{Cob}(\Sigma)</math> to the category <math>\operatorname{Kob}</math> of complexes of smoothings in the plane (with ordinary cobordisms for the differentials). Namely, map every smoothing <math>s</math> on <math>\Sigma</math> to the ordinary Khovanov homology complex of <math>\sigma_\star s</math>. Likewise, the image via <math>\sigma</math> of every morphism in <math>\operatorname{Cob}(\Sigma)</math> can be interpreted as a movie of smoothings and hence as a morphism between their Khovanov complexes. |
|||
Thus things seem to look good for re-embedding universality for Khovanov homology. Given <math>K</math> on <math>\Sigma</math>, compute its Khovanov homology <math>\operatorname{Kh}(K)</math> within <math>\Sigma</math> and get a complex in <math>\operatorname{Cob}(\Sigma)</math>. To that complex you can apply the above mentioned functor <math>\operatorname{Kh}_\sigma</math> and get a double complex in <math>\operatorname{Kob}</math>, whose collapse into a single complex by summing the diagonals ought to be <math>\operatorname{Kh}(\sigma_\star K)</math>. |
|||
Unfortunately, two problems arise: |
|||
# (Noted by Stephan Wehrli) The "movies to morphisms" map is only well defined up to signs. Thus it is reasonable to expect that <math>\operatorname{Kh}_\sigma</math> will only be unambiguous (and hence re-embedding universality will only hold) provided certain parity/genus/orientation conditions are met. I am not sure what these parity/genus/orientation conditions should be; but surely their determination can be taken as a further incentive for a better understanding of the signs that occur in the "movies to morphisms" map. Note that this problem may be a blessing in disguise - as Wehrli had noted (see below), if re-embedding universality was to hold without any parity/genus/orientation conditions, it would prove mutation invariance for certain links for which it is known to be false. |
|||
# <math>\operatorname{Kh}(K)</math> is only determined up to homotopy equivalence, and thus the application of <math>\operatorname{Kh}_\sigma</math> yields a double complex whose "columns" are only specified up to homotopy. The "collapse" of such a double complex is not well defined up to homotopy for reasons similar to the failure of our proof of mutation invariance, as mentioned [[#Failure of the "Almost Proof"|above]]. |
|||
I don't know if anything can be saved and at the end, I have no opinion as for whether or not Khovanov homology enjoys re-embedding invariance. I'd expect that the parity/genus/orientation issues of the first problem above will be resolved without too much difficulty. The homotopy equivalence issues of the second problem may be harder and deeper. |
|||
===Khovanov Homology, Re-Embedding Universality and Mutation Invariance=== |
|||
'''Exercise 1.''' Show that re-embedding universality for Khovanov homology implies mutation invariance of Khovanov homology for knots. |
|||
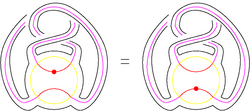
'''Hint.''' Consider the following figure: |
|||
[[Image:ReEmbeddingAndMutation.png|thumb|center|400px|'''Figure 2.''' On a surface, sliding red dots is a non-issue.]] |
|||
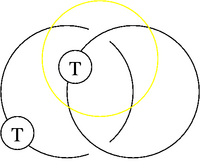
[[Image:ReEmbeddingUniversalityFailure.png|thumb|right|200px|A "T" here is an insertion of a trefoil tangle.]] |
|||
'''Exercise 2.''' (S. Wehrli) Show that naive re-embedding universality cannot hold in the situation described by the figure on the right, or else mutation invariance will hold for a link for which it is known to fail. |
|||
Note though that the surface <math>\Sigma</math> in this figure is of genus 1, while in the previous figure <math>\Sigma</math> is of genus 0. |
|||
===Alas=== |
|||
Alas, as of July 2006 it seems that re-embedding universality does not hold for Khovanov homology. Indeed, Khovanov homology is not invariant under the "cabled mutation" of {{ref|Dunfield_Garoufalidis_Shumakovitch_Thistlethwaite}} (see especially pages 8-9). But it seems that re-embedding universality for Khovanov homology implies invariance under cabled mutation and hence the former cannot hold. Some further details and discussion may appear here in the future. |
|||
==A Partial Success by Stephan Wehrli== |
|||
In mid 2007 Stephan Wehrli was able to complete argument of the "first attempt" above, though only over <math>{\mathbb Z}/2{\mathbb Z}</math>. See his upcoming paper on the subject, and meanwhile, see the slides of a talk he gave about the subject in [http://www.math.kyoto-u.ac.jp/~nakajima/07_Link%20homology%20and%20categorification/schedule.html Kyoto] (his is the last link on that page). |
|||
==References== |
==References== |
||
{{note|Bar-Natan}} Dror Bar-Natan, [http://www.math.toronto.edu/~drorbn/papers/Cobordism/ Khovanov's Homology for Tangles and Cobordisms], Geometry and Topology '''9-33''' (2005) 1443-1499. |
{{note|Bar-Natan}} Dror Bar-Natan, ''[http://www.math.toronto.edu/~drorbn/papers/Cobordism/ Khovanov's Homology for Tangles and Cobordisms]'', Geometry and Topology '''9-33''' (2005) 1443-1499. |
||
{{note|Dunfield_Garoufalidis_Shumakovitch_Thistlethwaite}} Nathan Dunfield, Stavros Garoufalidis, Alexander Shumakovitch and Morwen Thistlethwaite, ''Behavior of Knot Invariants under Genus 2 Mutation'', {{arXiv|math.GT/0607258}}. |
|||
Latest revision as of 13:50, 26 June 2007
|
Abstract. The purpose of this note is to sketch my "almost proof" of mutation invariance for Khovanov homology of knots and to discuss "re-embedding universality" for Khovanov homology.
History And Summary
I have presented an almost-proof of invariance of Khovanov homology under knot mutation in a conference in Snowbird, Utah in June 2005 (see the handouts from that talk here). At the time it was clear to me that the very end of the proof was a bit fishy, and an example by Jake Rasmussen made it look even fishier.
Yet the proof seemed to have all the right ingredients in it (for example, it was restricted to the case of knots; indeed, mutation invariance of Khovanov homology for links is known to fail). So I was quite sure the missing bit will be completed quickly. A short time later I (thought I) was able to complete the proof, and for a few months I believed my proof was right, though I did not write it up so I was never confident.
Then in October 2005 I explained my proof over the phone to Stephan Wehrli in Zurich, and in December 2005 he contacted me again with a gap in the proof. I still don't know how to resolve the difficulty and I am no longer convinced that Khovanov homology is invariant under knot mutation.
Yet not all is lost:
- The proof remains "almost true" and still seems to have (at least many of) the right ingredients. Thus somebody may well be able to complete it one day.
- The parts of the proof that still hold suggest ways to narrow down the search for counterexamples for mutation invariance. Thus with the proper software there is some real hope that a counterexample will be found.
- In an effort to save the proof I realized that mutation invariance is implied by a much broader and more conceptual suggested property of Khovanov homology, the property of "re-embedding universality". The difficulty in proving re-embedding universality is the same as the difficulty in proving mutation invariance, but the benefit, if proven, seems greater. I hope people will put some effort into understanding re-embedding universality.
What's Mutation?
A mutation is the process of marking a circle on a knot or a link diagram which intersects it at precisely four points, and flipping its inside (the inside tangle), yet not its outside (the outside tangle), around a horizontal axis as illustrated on the right. (Other types of mutation are topologically equivalent to this one). Mutation does not change the overall connectivity of the knot/link in question. Thus a knot stays a knot and a link stays a link.
It is well known that the Jones polynomial, as well as the Alexander, HOMFLYPT, and coloured Jones polynomials, are invariant under knot mutation. There are easy examples to show that Khovanov homology is not invariant under link mutation, but it is still unknown whether or not Khovanov homology is invariant under knot mutation.
The Almost Proof
Let us present an almost proof of invariance of Khovanov homology under knot mutation.
The Setup
Assume that the connectivity of the inside and outside tangles are as in Figure 1. With minor effort, all other possible connectivity possibilities for knot mutation can be treated in similar ways. (In fact, the almost proof below works just as well for link mutations in which "there is no exchange of genetic material" between the two link components involved). Let be the knot in question "before" the mutation, and let be the "after" knot. Likewise let and be the inside tangle before and after mutation (so is the horizontal flip of ) and let be the (fixed) outside tangle.
Using the constructions of [Bar-Natan] we have that "the overall is the tensor product of the inside and the outside": and . Thus let us start by comparing with .
For simplicity we work in the category of "dotted cobordisms" as in Section 11.2 of [Bar-Natan].
The Inside Story
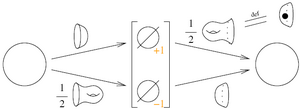
It is well known (though not well documented, and I have only myself to blame), that in a circle is isomorphic to two empty sets (with some grading shifts; a proof is on the right).
After this isomorphism is used repeatedly on , the objects in the complex that remains have no loops, and there are only two possibilities for such objects - the "horizontal smoothing" and the "vertical smoothing". Furthermore, there are only 12 possible cobordisms whose tops and bottoms are horizontal or vertical smoothings. Thus can be seen as taking values in a (very) finite category consisting of 2 objects and 12 morphisms ("arrows"), as summarized in the following picture:
The construction of and the process of "delooping" are clearly equivariant under horizontal (or vertical, for that matter) flips and so is obtained from simply by flipping all objects and morphisms in sight. But these all fall within the category in the picture above, and all two of the objects and 10 of the 12 morphisms are clearly invariant under flips. The remaining two morphisms are drawn in red in the above picture. The only difference between and is that every occurrence of a "red" morphism in becomes the "other red" in .
The Outside Story
We claim that relative to the outside differential, the two "red" morphisms of the "inside" invariant are homotopic. The key point is in the picture below:
This picture shows (by displaying an explicit homotopy) that the two endomorphisms and of the complex of a single crossing, obtained by a horizontal composition with a dotted curtain either on the upper left or on the lower right are homotopic. This can be iterated to "drag" dots across crossings and thus, as the connectivity of strands in is as in Figure 1, the "dot" in any one "red" morphism in can be pulled around the outside so as to turn it into the other "red" morphism.
(Notice: this is where the given connectivity for the outside is used, and it is at this point that our almost proof fails entirely for links).
(To be precise, the "outside" image shows that . But there is an even number of crossings on the outside strand that our dot needs to travel, so overall, signs work out right).
The Inside Meets the Outside
In summary, and are the homologies (or just the homotopy classes) of two double complexes that share a vertical differential but may have different horizontal differentials, and , and those two horizontal differentials are homotopic relative to the vertical differential using a homotopy .
Note that and here are not quite the same as and of the previous section - and were just single "red" parts of the 's and 's here, and dots were just being "transported" across a single crossing. and may differ by having many red parts, and the dots on these red parts may need transportation across many crossings. So is a (possibly) complex assembly of many versions of "installed" at (possibly) many locations on the outside tangle and within (possibly) many entries in the double complex.
One would hope that with such a close relationship between the complexes for and it should be easy to prove that the two homologies are equal.
Failure of the "Almost Proof"
Unfortunately it is simply not true that homotopic horizontal differentials in a double complex lead to equal overall homology. A nice counterexample was given already in Utah by Jake Rasmussen, and is reproduced on the left here (dots stand for vectors and the maps are presented by colour-coded arrows).
It is not difficult to show that if in addition commutes with and then is an isomorphism between the two double complexes. For a while I believed I could show that in our case indeed commutes with and and the problem is resolved. But in December 2005 Stephan Wehrli asked for the details, and it turned out I didn't have them (no counterexample yet, either). At this time neither I nor he know how to revive the proof.
A Second Attempt - "Re-Embedding Universality"
We will say that a knot invariant has "re-embedding universality" if whenever a knot is drawn on a surface and the surface is embedded in space, one can recover the invariant of the "pushforward" knot in space from the invariant of that knot on the surface and from information about the embedding of the surface in space.
After saying the same thing again in a few more words, we'll state the obvious fact that the Jones polynomial has re-embedding universality and ask if Khovanov homology has that too. If it does, mutation invariance holds (for knots) for Khovanov homology. That would be a lovely corollary of re-embedding universality. Yet IMHO, re-embedding universality is such a lovely abstract property of the Jones polynomial that resolving whether or not it holds for Khovanov homology is much more significant than deciding the issue of mutation invariance.
Re-Embedding Universality in More words
Suppose a knot (or a link) is drawn on a surface (i.e., embedded in , via a map , where is a circle or a union of circles), and suppose that in turn, is embedded in via . Extend to a homeomorphism of with a tubular neighbourhood of and abuse the notation a bit by denoting the resulting extension again by . We can now regard also as a knot in which perhaps is better denoted .
Suppose is some knot/link invariant which is defined for knots drawn in the plane (i.e., knots in ) as well as for knots/links drawn on other surfaces.
We will say that has "re-embedding universality" if and together determine . This is a natural "good behaviour" property for which somehow says that there is no loss of information in computing for knots that are embedded "in steps".
The Jones Polynomial has Re-Embedding Universality
There's nothing to say here, really. Consider the Jones polynomial for surface knots using Kauffman bracket skein modules (so that it is valued in -linear combinations of "smoothings" on . Once is given, each smoothing on becomes a knot in which has its own Jones polynomial, and the appropriate linear combination of these Jones polynomials is the Jones polynomial of ; this is merely the fact that in resolving crossings to smoothings, one may first resolve the crossings "on " and then the crossings induced from the projection of to the plane.
In fairness, a minor complication does arise - the Jones polynomial is defined for oriented knots/links, while smoothings are unoriented. But orientation issues only amount to signs and overall powers of . So you may either ignore those, or do the right thing, work a bit harder, and find a way to incorporate a bit of extra orientation information into the definition of for surface knots. Most likely it will be enough to declare that the "enhanced Jones" of is the "old Jones" of along with . I will ignore this issue below.
Khovanov Homology and Re-Embedding Universality
Does Khovanov homology enjoy re-embedding universality?
The complete locality of the constructions in [Bar-Natan] allows them to be easily generalized to knots on surfaces. In short, Khovanov homology for knots on takes values in formal complexes in the category whose objects are smoothings in and whose morphisms are cobordisms in between such smoothings. For a given knot , call the resulting complex .
Given an embedding of in there is a natural functor taking to the category of complexes of smoothings in the plane (with ordinary cobordisms for the differentials). Namely, map every smoothing on to the ordinary Khovanov homology complex of . Likewise, the image via of every morphism in can be interpreted as a movie of smoothings and hence as a morphism between their Khovanov complexes.
Thus things seem to look good for re-embedding universality for Khovanov homology. Given on , compute its Khovanov homology within and get a complex in . To that complex you can apply the above mentioned functor and get a double complex in , whose collapse into a single complex by summing the diagonals ought to be .
Unfortunately, two problems arise:
- (Noted by Stephan Wehrli) The "movies to morphisms" map is only well defined up to signs. Thus it is reasonable to expect that will only be unambiguous (and hence re-embedding universality will only hold) provided certain parity/genus/orientation conditions are met. I am not sure what these parity/genus/orientation conditions should be; but surely their determination can be taken as a further incentive for a better understanding of the signs that occur in the "movies to morphisms" map. Note that this problem may be a blessing in disguise - as Wehrli had noted (see below), if re-embedding universality was to hold without any parity/genus/orientation conditions, it would prove mutation invariance for certain links for which it is known to be false.
- is only determined up to homotopy equivalence, and thus the application of yields a double complex whose "columns" are only specified up to homotopy. The "collapse" of such a double complex is not well defined up to homotopy for reasons similar to the failure of our proof of mutation invariance, as mentioned above.
I don't know if anything can be saved and at the end, I have no opinion as for whether or not Khovanov homology enjoys re-embedding invariance. I'd expect that the parity/genus/orientation issues of the first problem above will be resolved without too much difficulty. The homotopy equivalence issues of the second problem may be harder and deeper.
Khovanov Homology, Re-Embedding Universality and Mutation Invariance
Exercise 1. Show that re-embedding universality for Khovanov homology implies mutation invariance of Khovanov homology for knots.
Hint. Consider the following figure:
Exercise 2. (S. Wehrli) Show that naive re-embedding universality cannot hold in the situation described by the figure on the right, or else mutation invariance will hold for a link for which it is known to fail.
Note though that the surface in this figure is of genus 1, while in the previous figure is of genus 0.
Alas
Alas, as of July 2006 it seems that re-embedding universality does not hold for Khovanov homology. Indeed, Khovanov homology is not invariant under the "cabled mutation" of [Dunfield_Garoufalidis_Shumakovitch_Thistlethwaite] (see especially pages 8-9). But it seems that re-embedding universality for Khovanov homology implies invariance under cabled mutation and hence the former cannot hold. Some further details and discussion may appear here in the future.
A Partial Success by Stephan Wehrli
In mid 2007 Stephan Wehrli was able to complete argument of the "first attempt" above, though only over . See his upcoming paper on the subject, and meanwhile, see the slides of a talk he gave about the subject in Kyoto (his is the last link on that page).
References
[Bar-Natan] ^ Dror Bar-Natan, Khovanov's Homology for Tangles and Cobordisms, Geometry and Topology 9-33 (2005) 1443-1499.
[Dunfield_Garoufalidis_Shumakovitch_Thistlethwaite] ^ Nathan Dunfield, Stavros Garoufalidis, Alexander Shumakovitch and Morwen Thistlethwaite, Behavior of Knot Invariants under Genus 2 Mutation, arXiv:math.GT/0607258.


































![{\displaystyle {\mathbb {Z} }[q,q^{-1}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/769507ccf3974f6ba7926efff86a9b4e52ef4e33)