Notes for AKT-140120/0:22:11

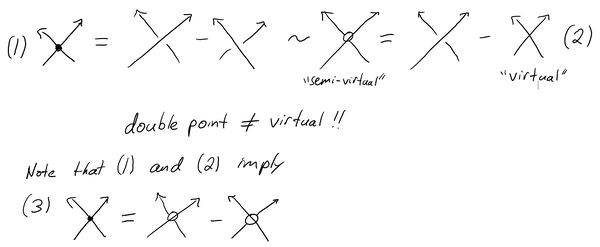
Much like the concept of a type n knot invariant described in this lecture, there is such a thing as a type n invariant of "virtual knots". Virtual knots can be defined (among other ways) as equivalence classes of Gauss diagrams under a certain set of generalized Reidemeister moves. Refer to the note at time 31:27 of the video found at http://drorbn.net/dbnvp/AKT-140127.php, and Goussarev, Polyak, and Viro's paper (https://arxiv.org/abs/math/9810073) for more information on Gauss diagrams and the specific set of moves. Equivalently, one can think of virtual knots as quadrivalent planar graphs, where each vertex can either be the usual over/undercrossing pair as in regular knots, or a so-called "virtual crossing", at which the lines simply cross each other with no extra information. Analogous to the double point in the regular knot situation is the "semi-virtual crossing", given by an analogous relation (illustrated below).

An invariant $v: \mathcal{VK} \rightarrow A$ (where $\mathcal{VK}$ is the space of virtual knots and $A$ is some abelian group) of virtual knots is said to be of type $n$ if it vanishes on virtual knots with $n+1$ semi-virtual crossings. By virtue of equation (3) above, it can be shown that type $n$ virtual knot invariants, when restricted to honest knots, are knot invariants of type at least n. Namely, a type n virtual knot invariant evaluated on $n+1$ double points is a sum of evaluations of $v$ on $n+1$ semi-virtual crossings (by equation 3), and is therefore equal to 0.