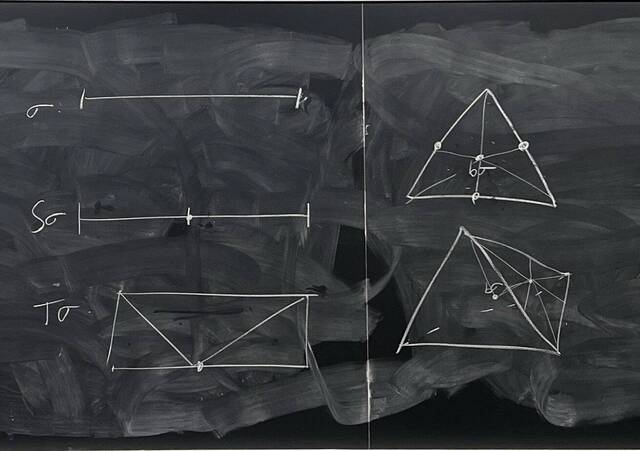
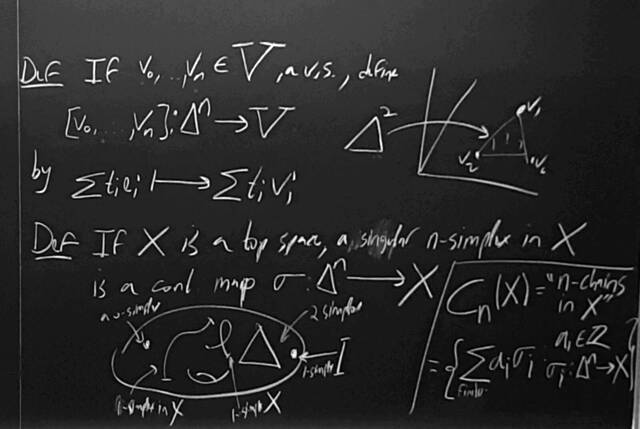
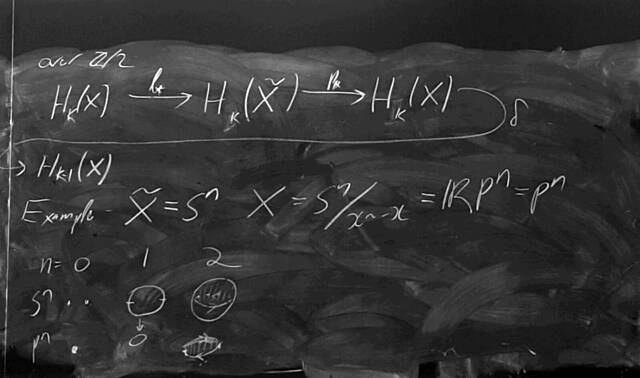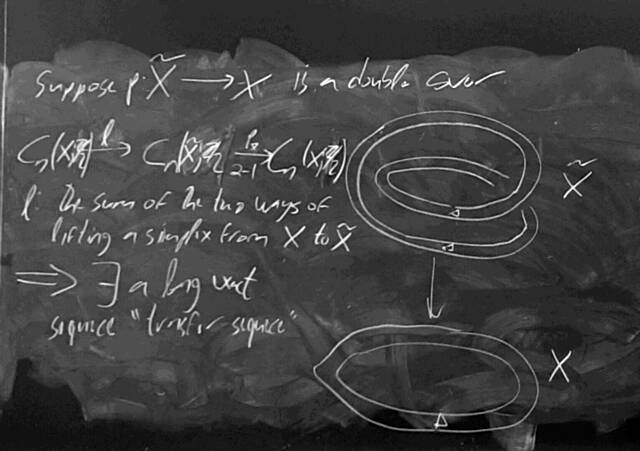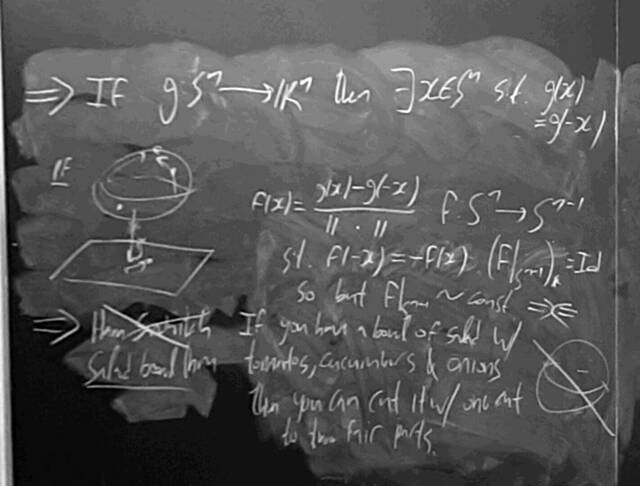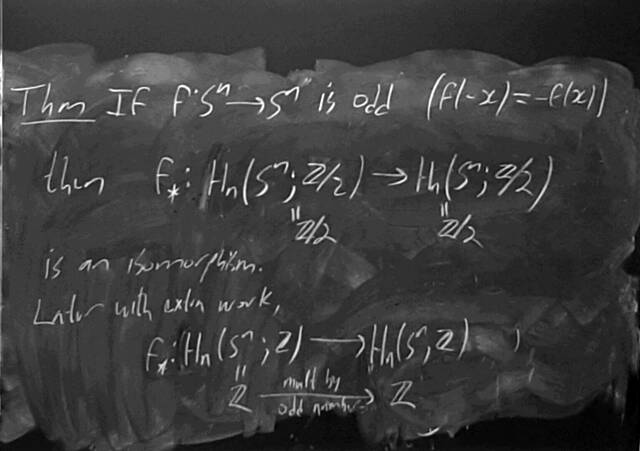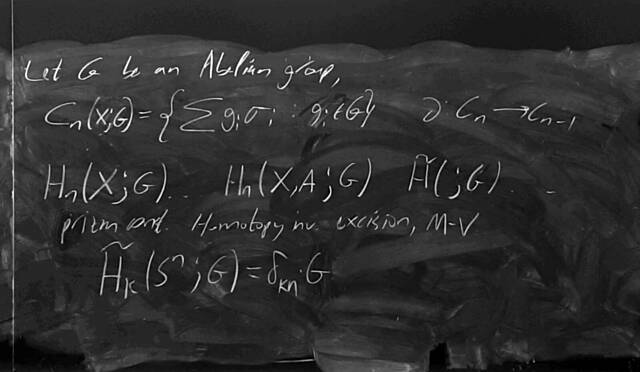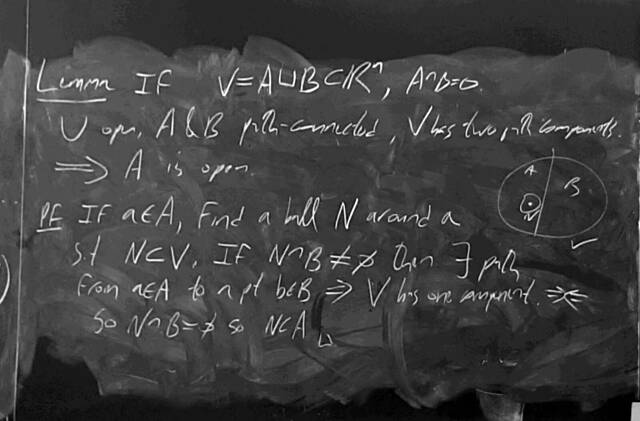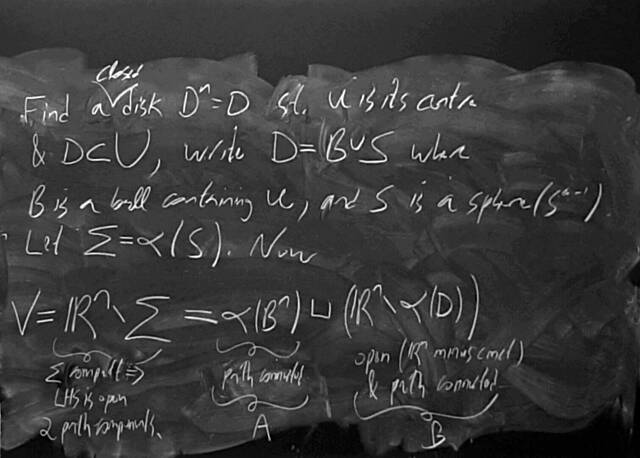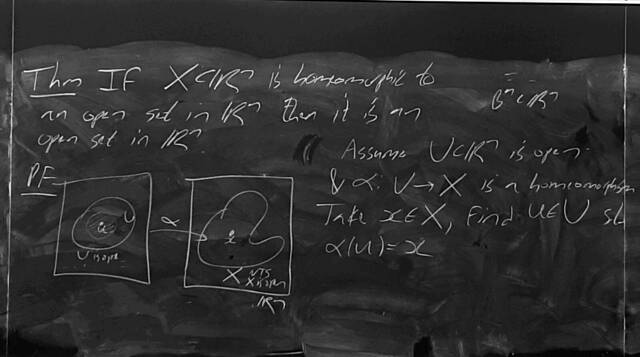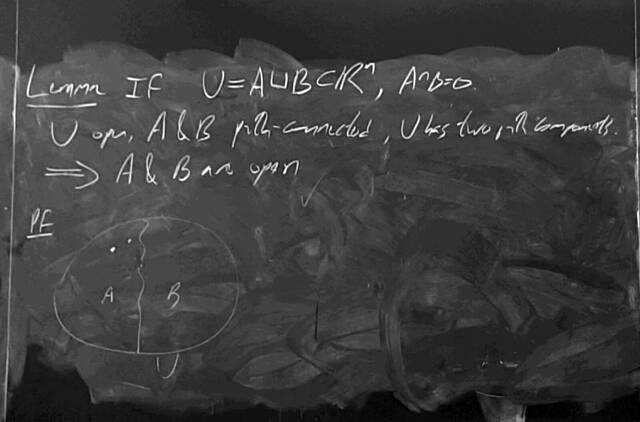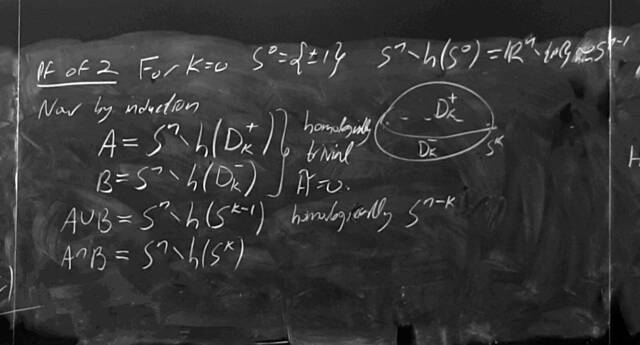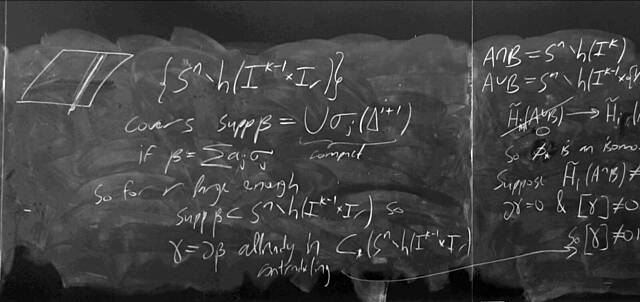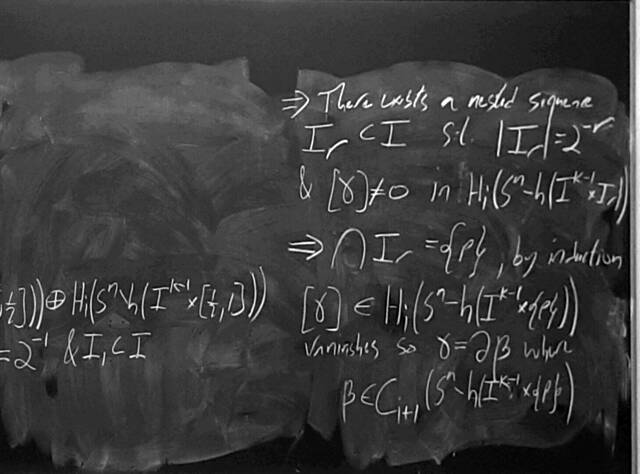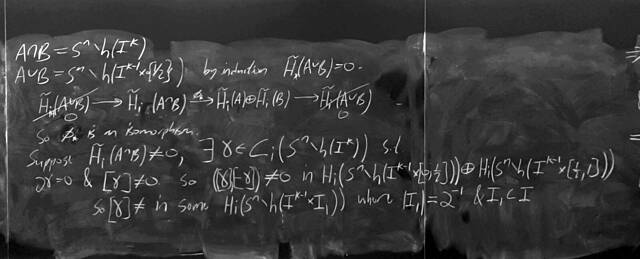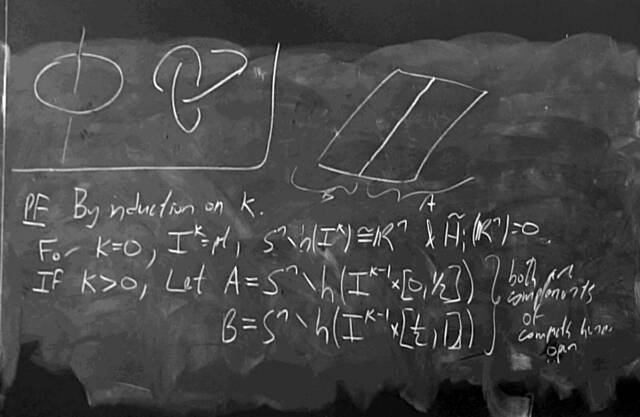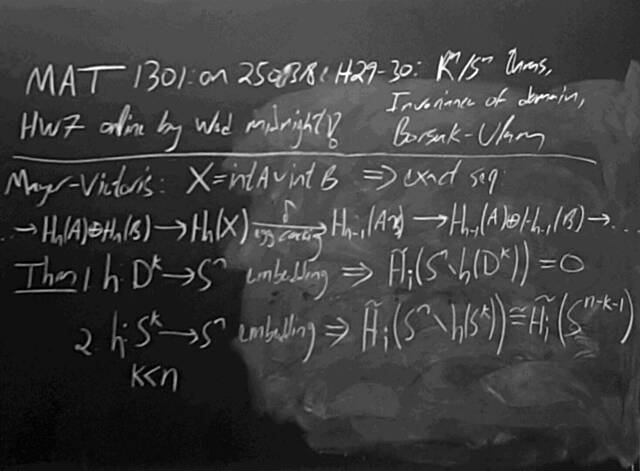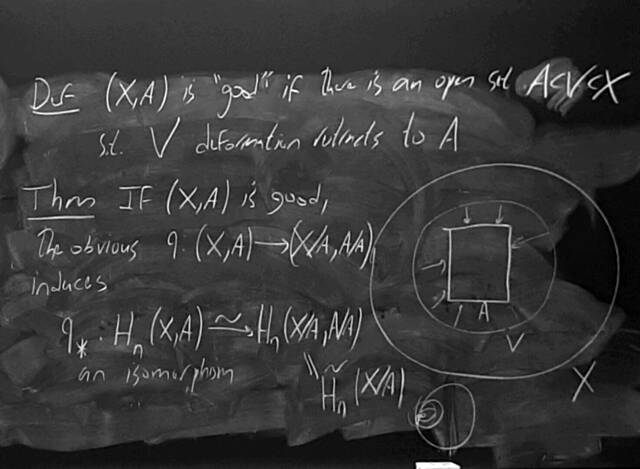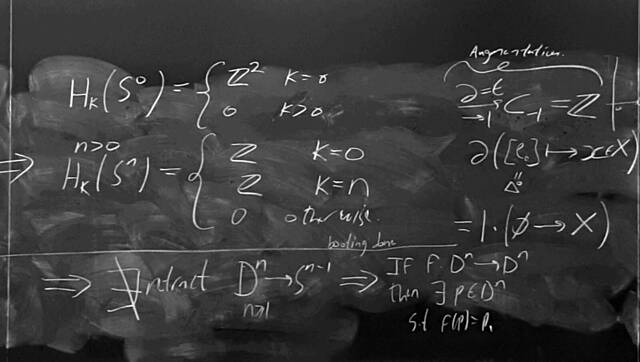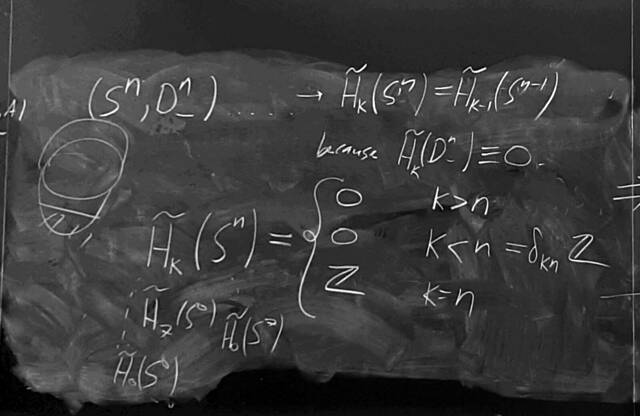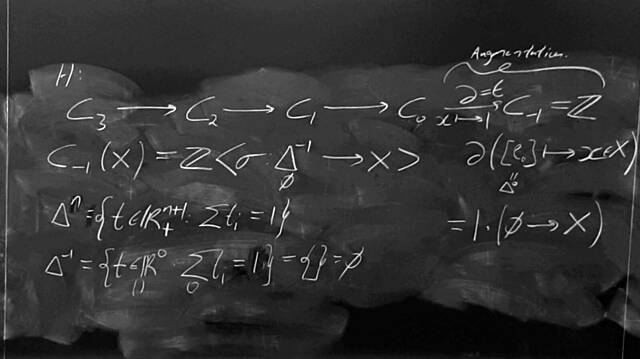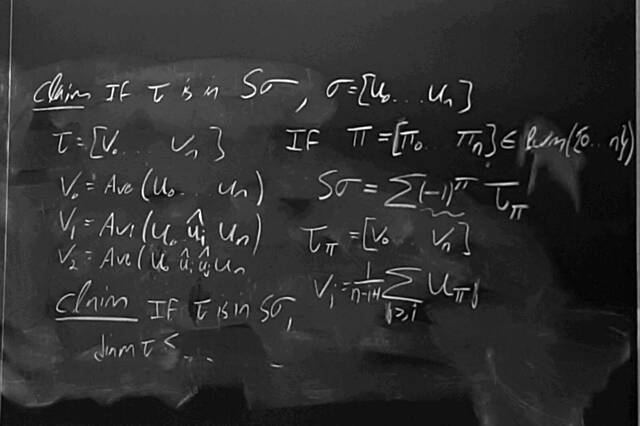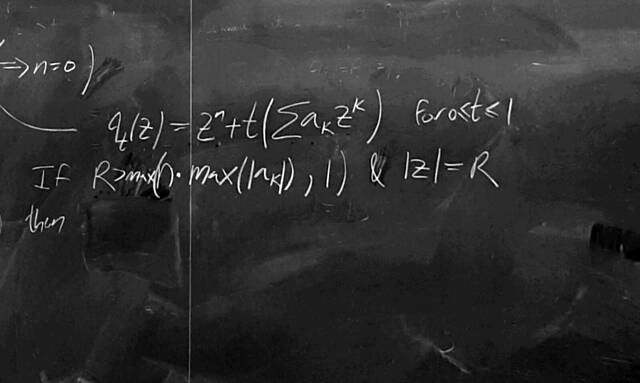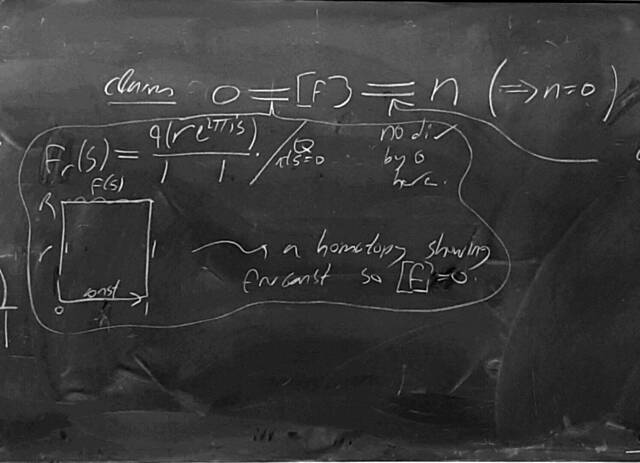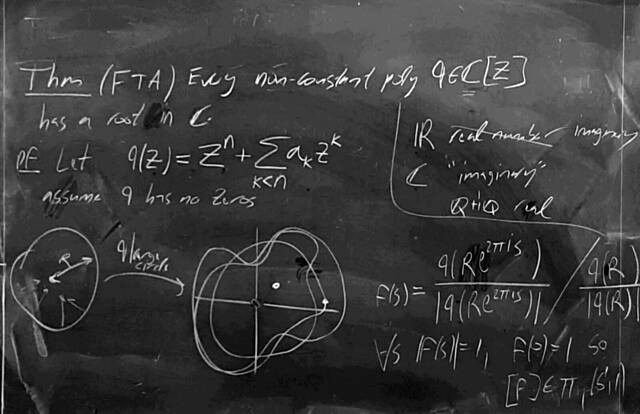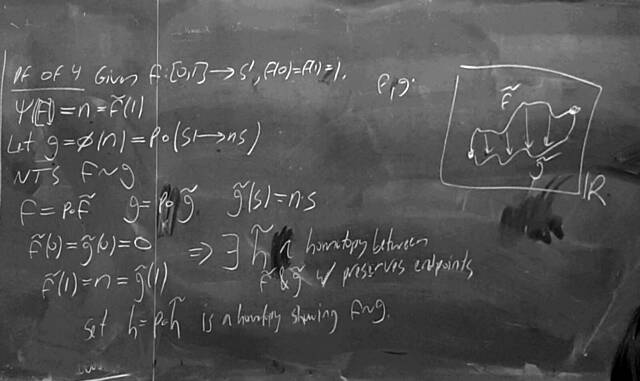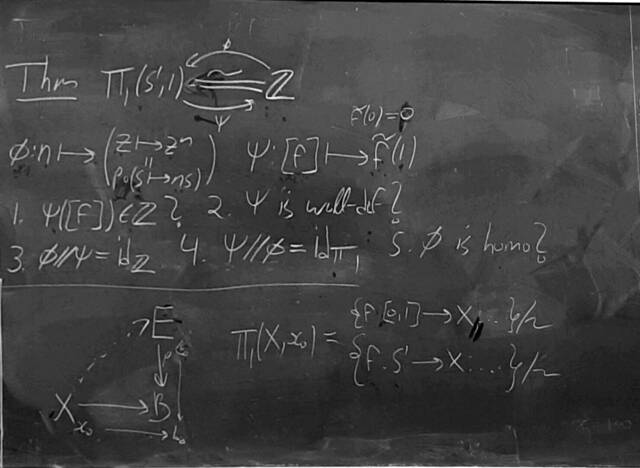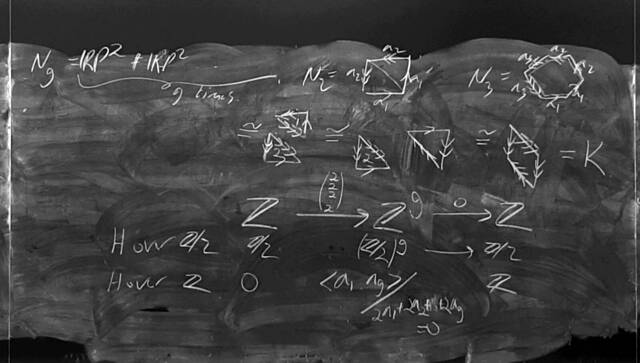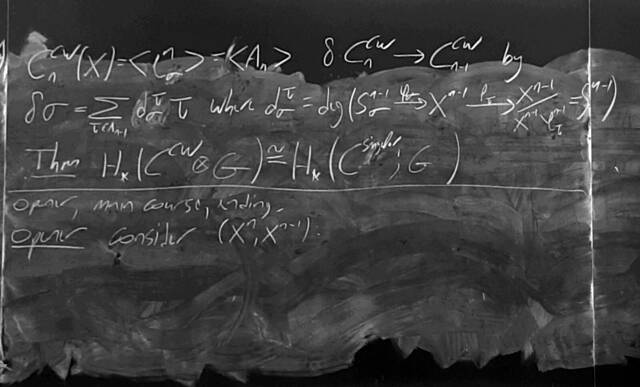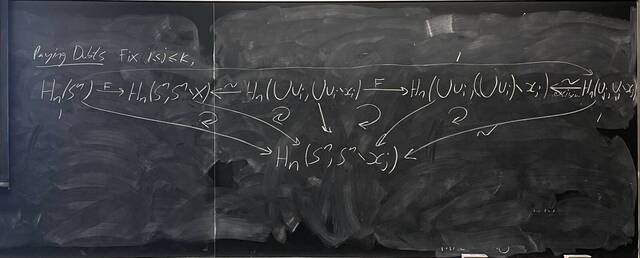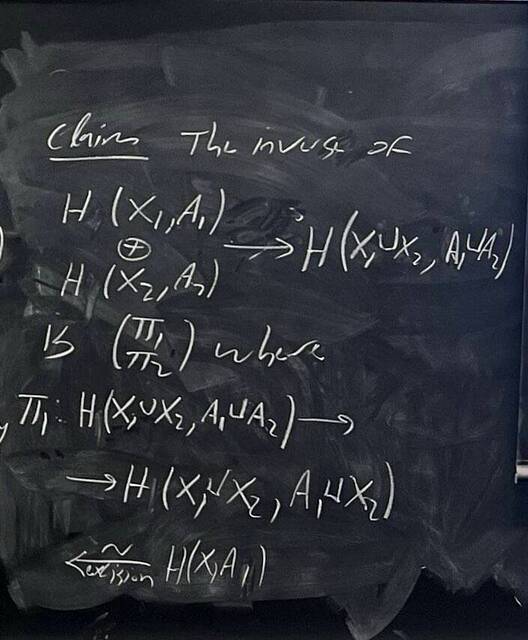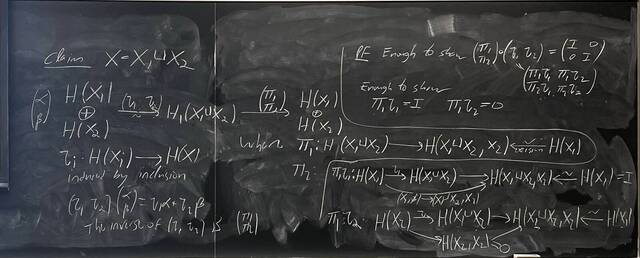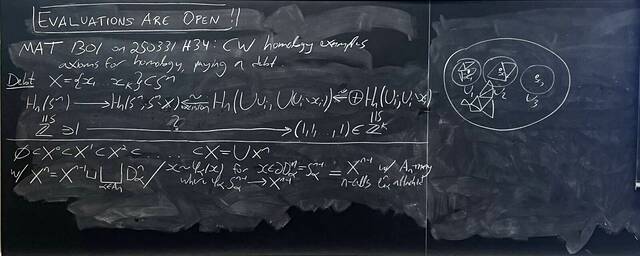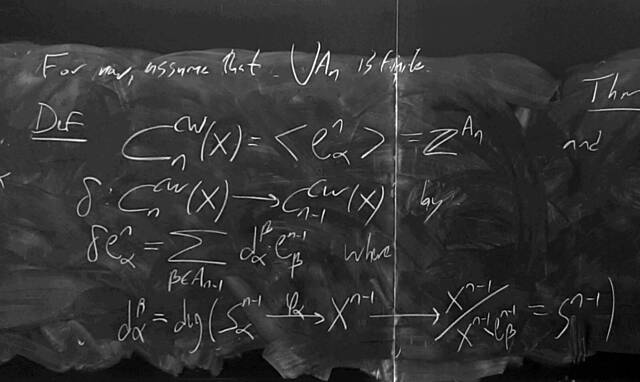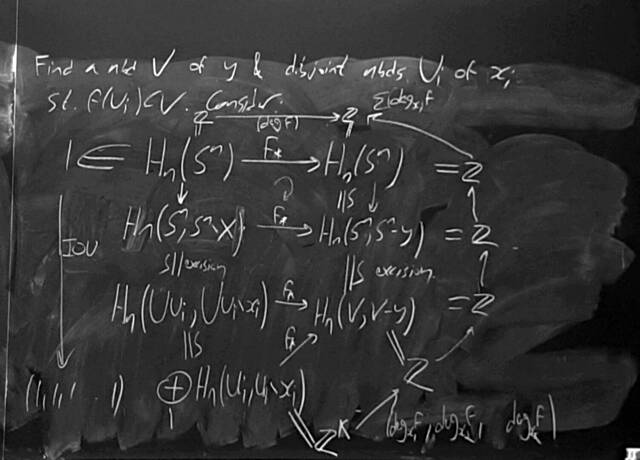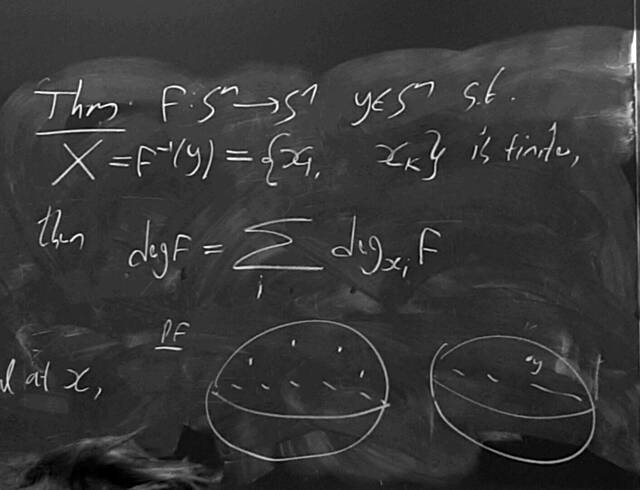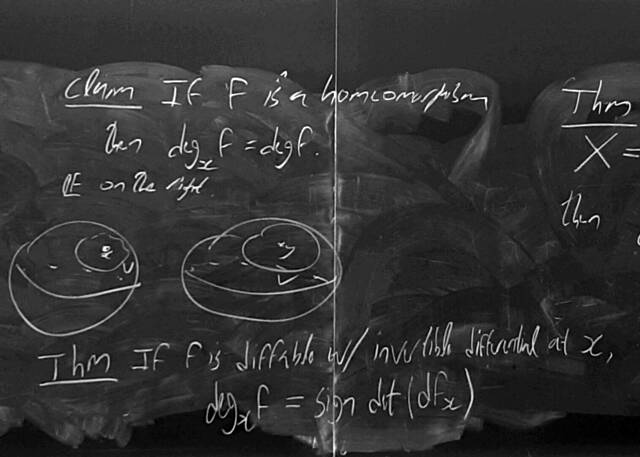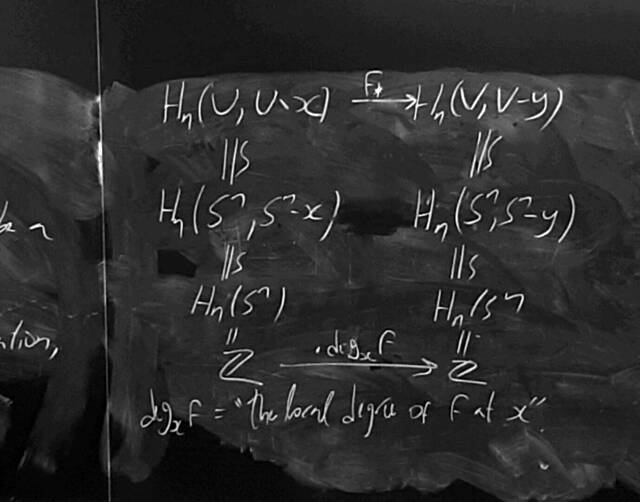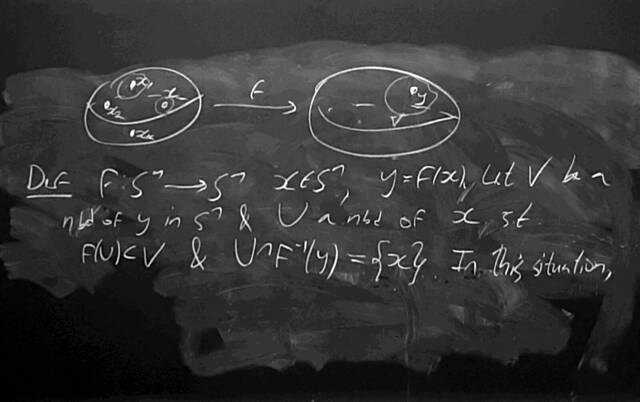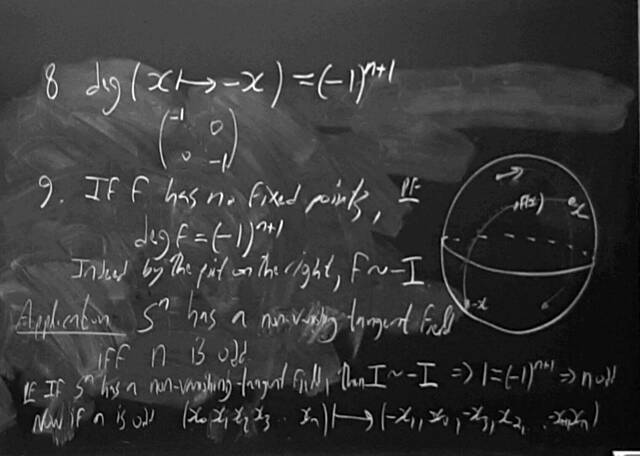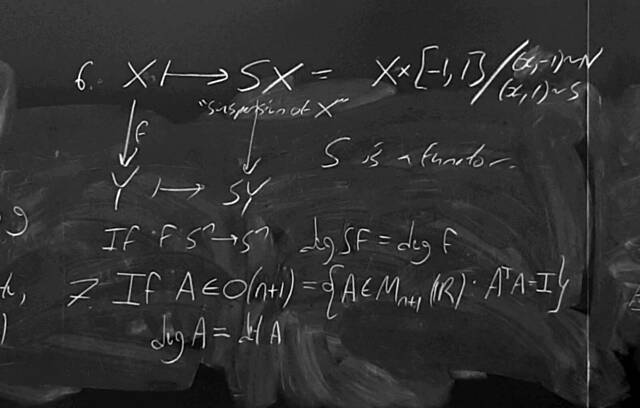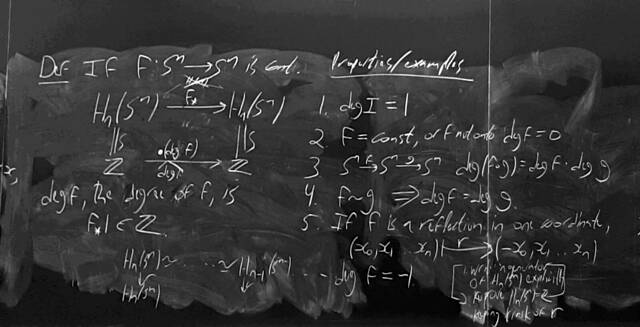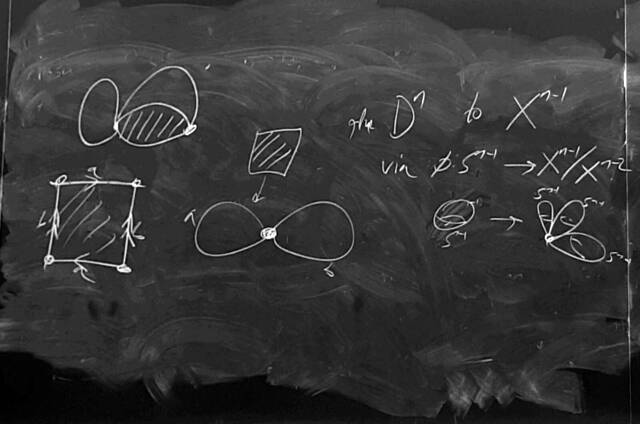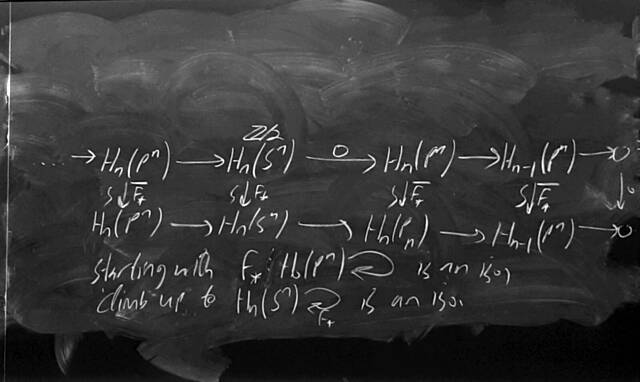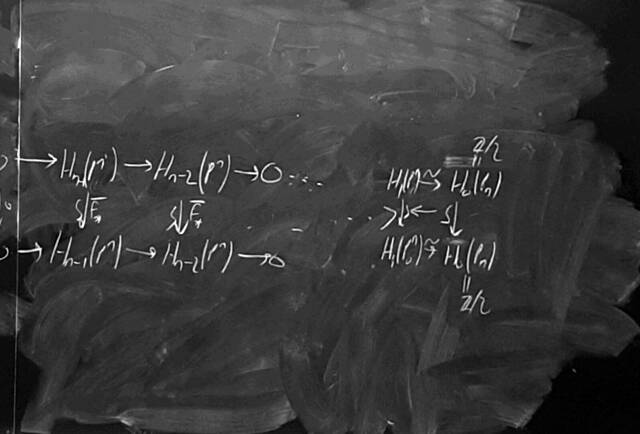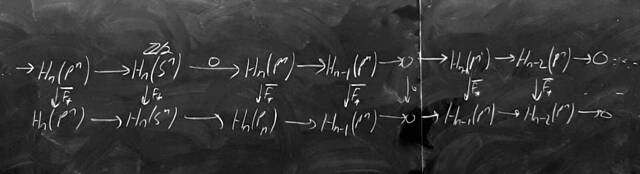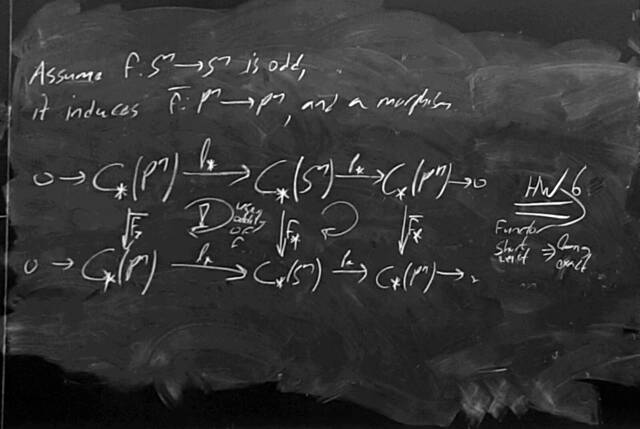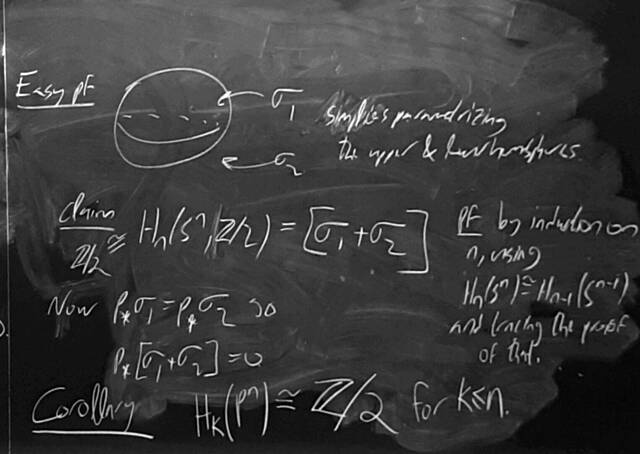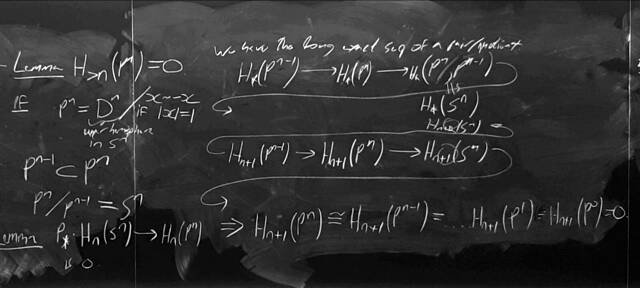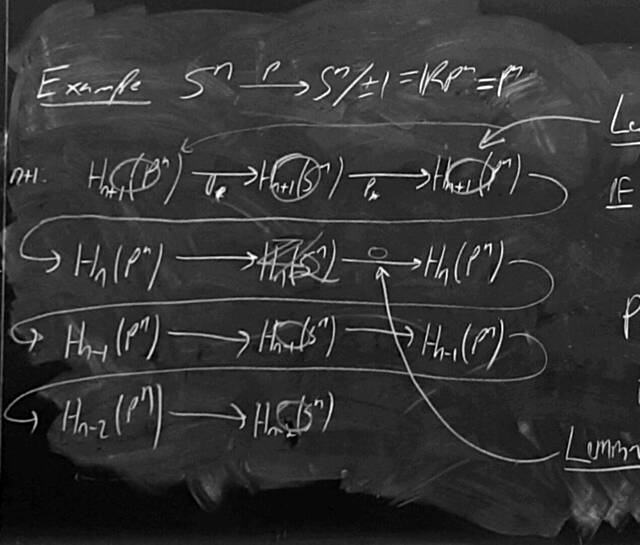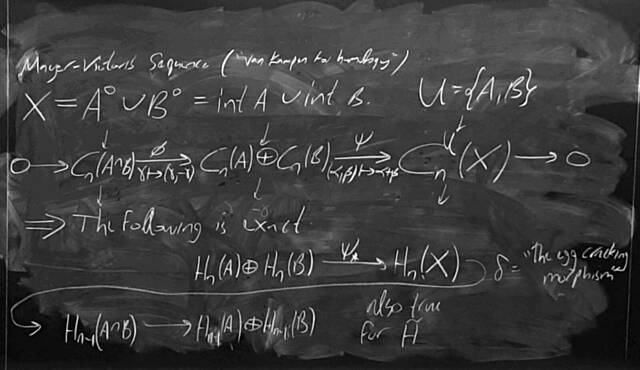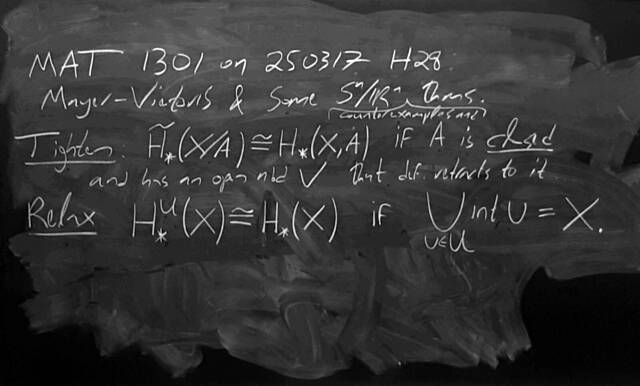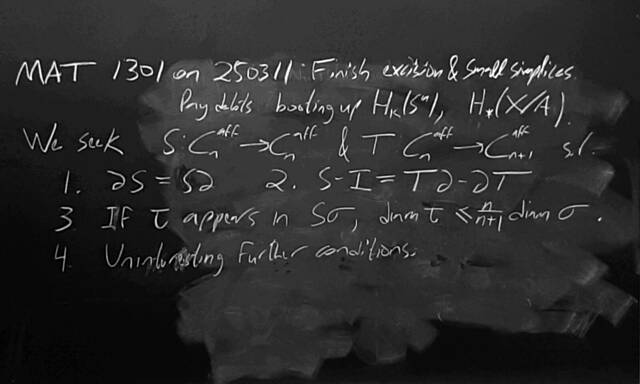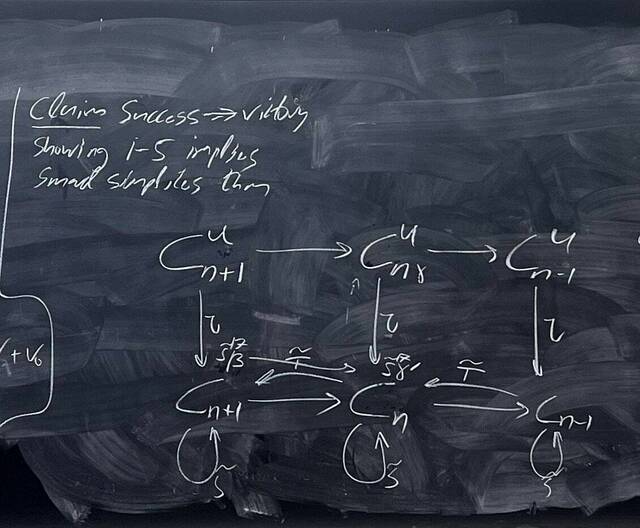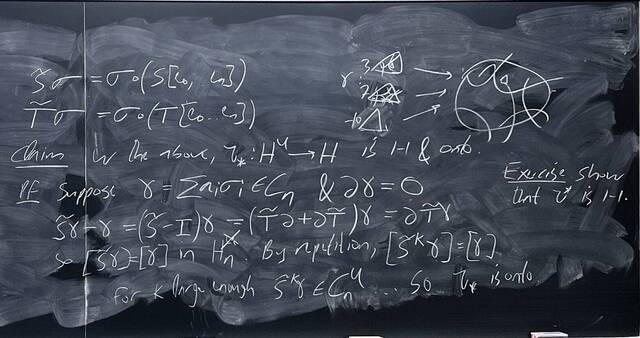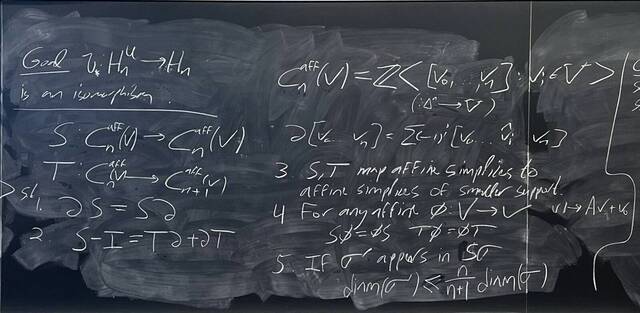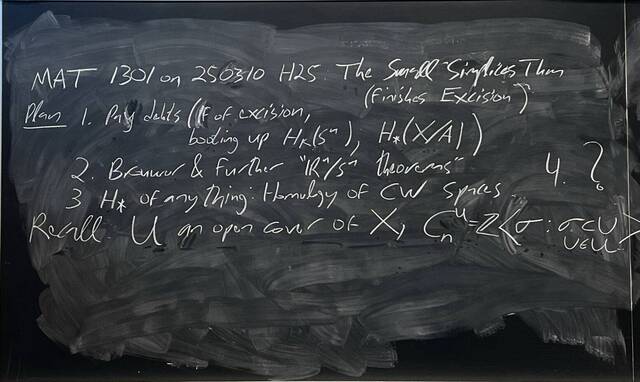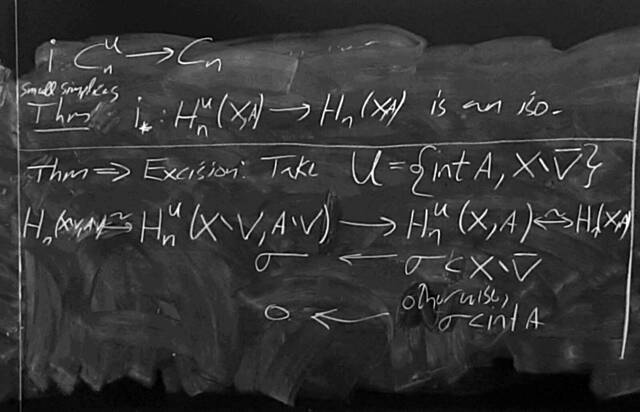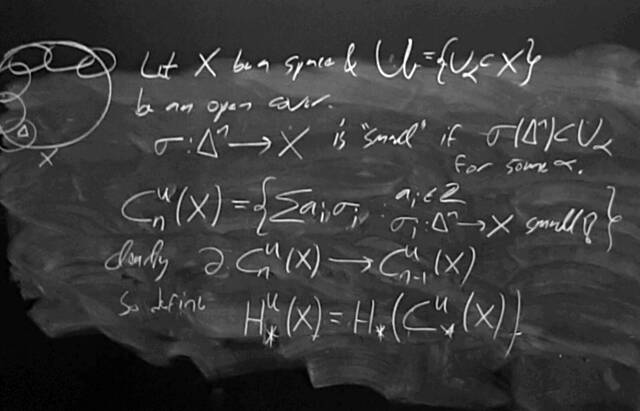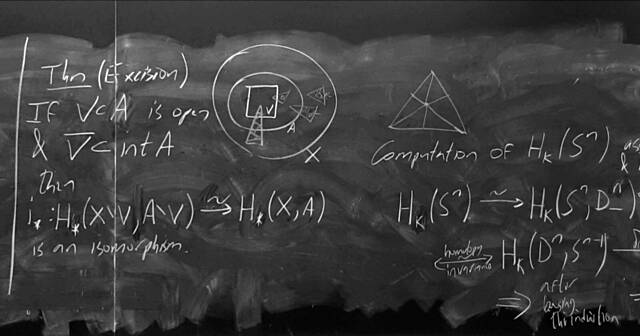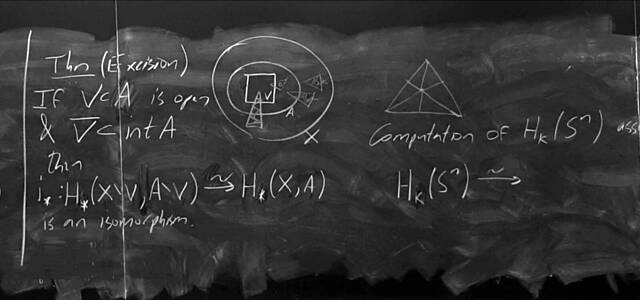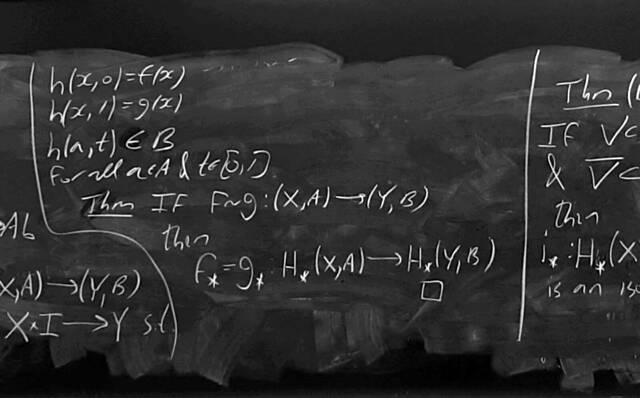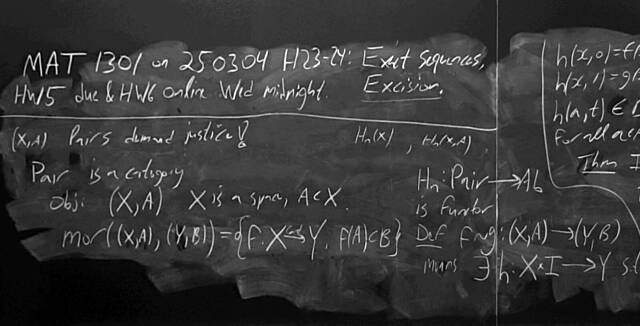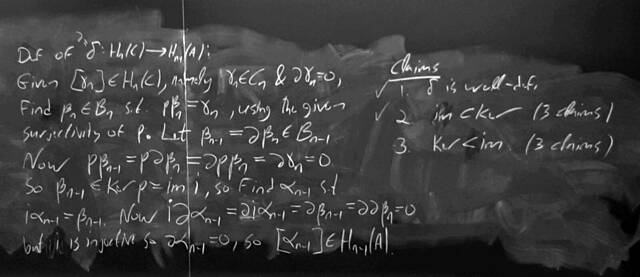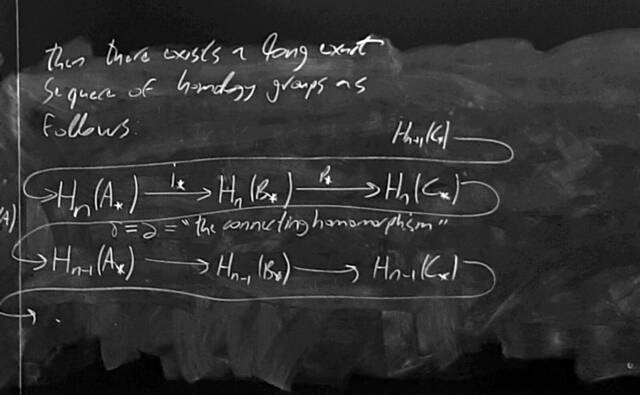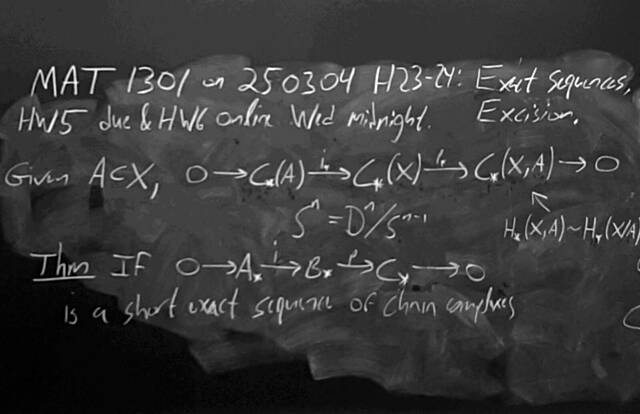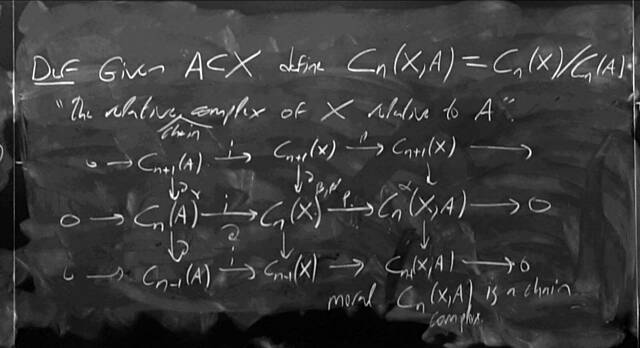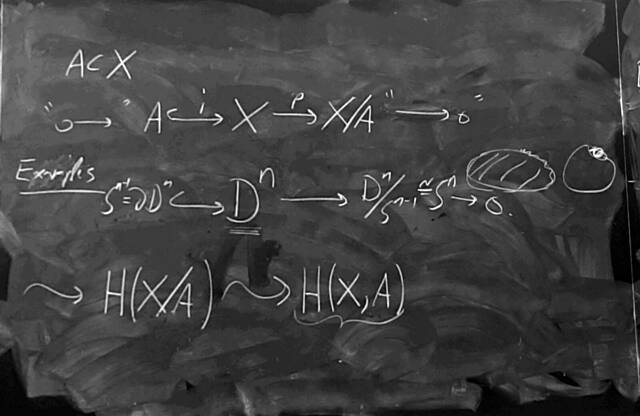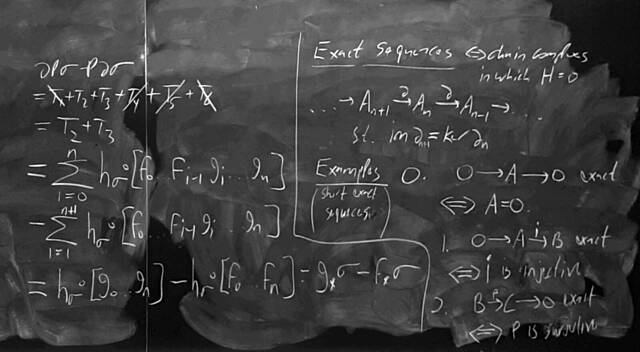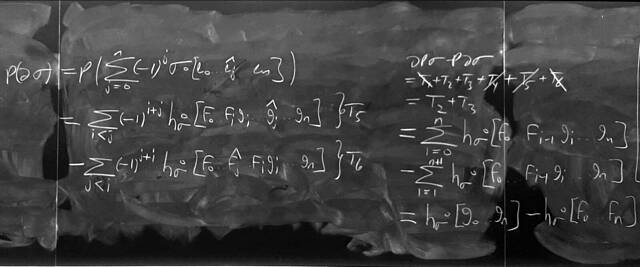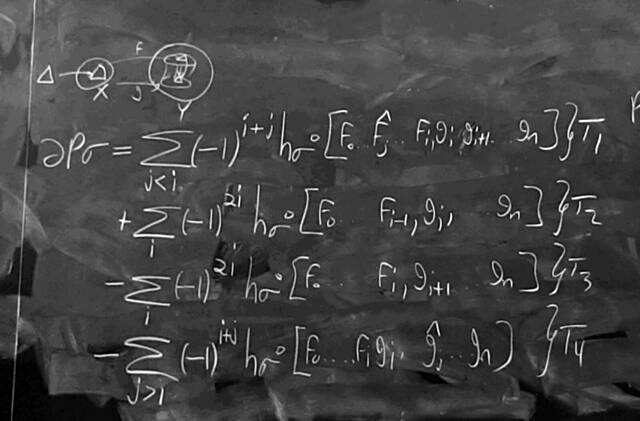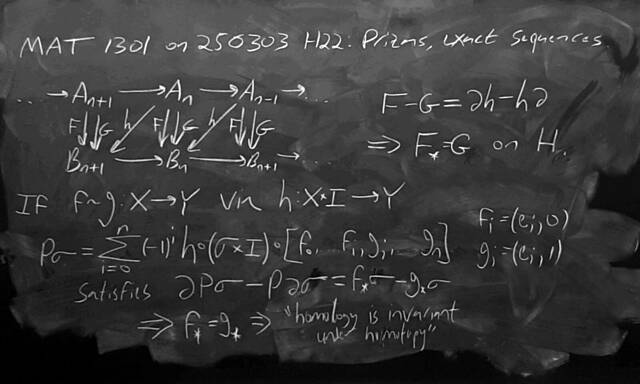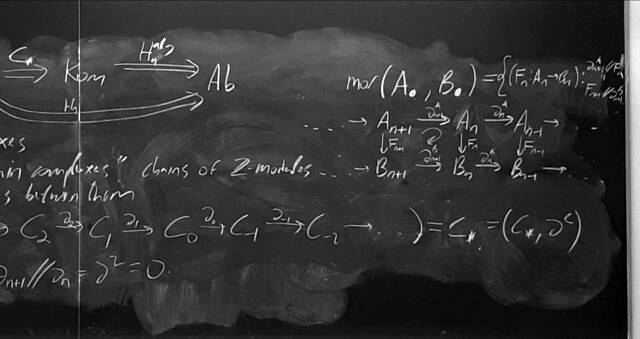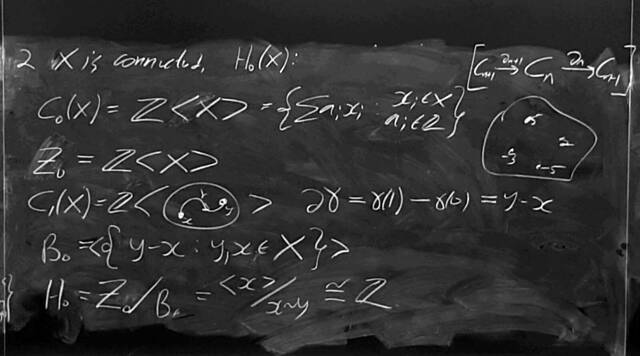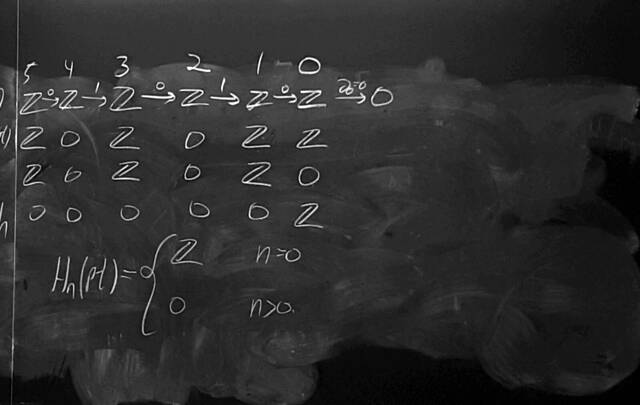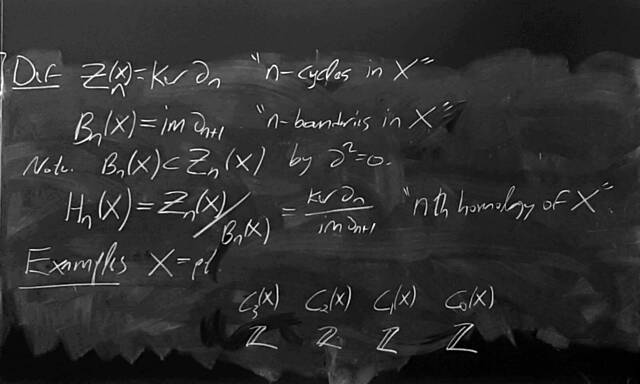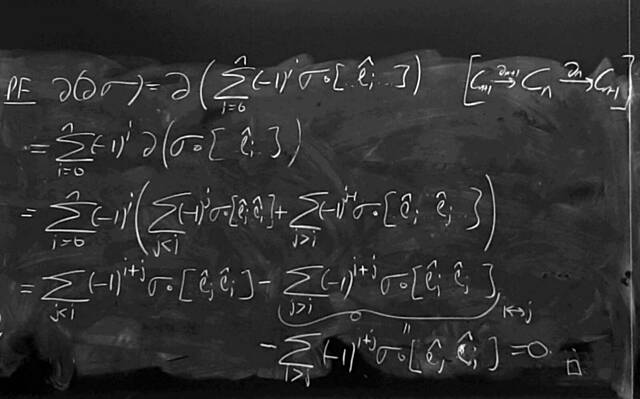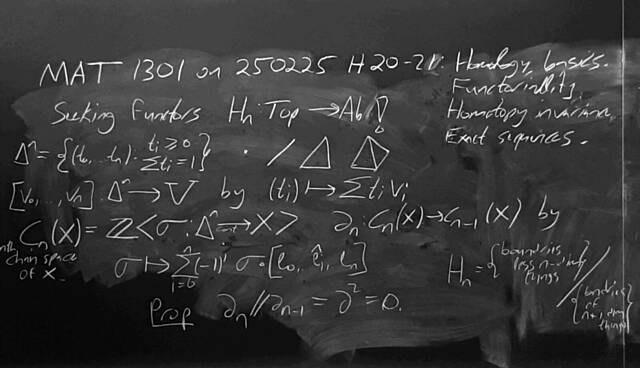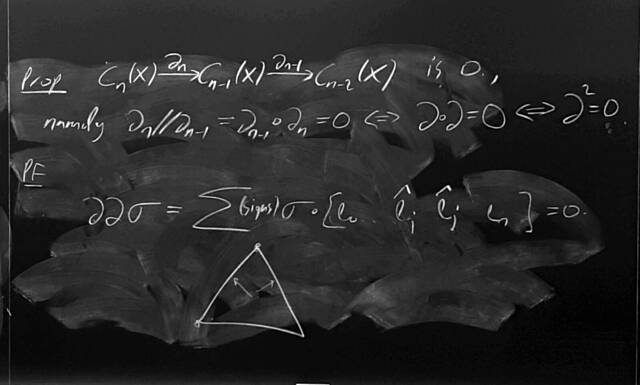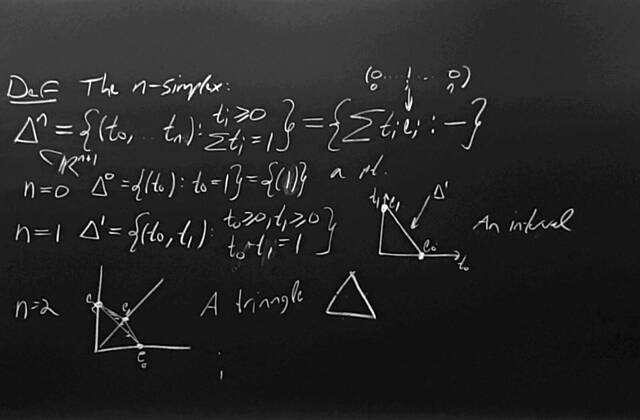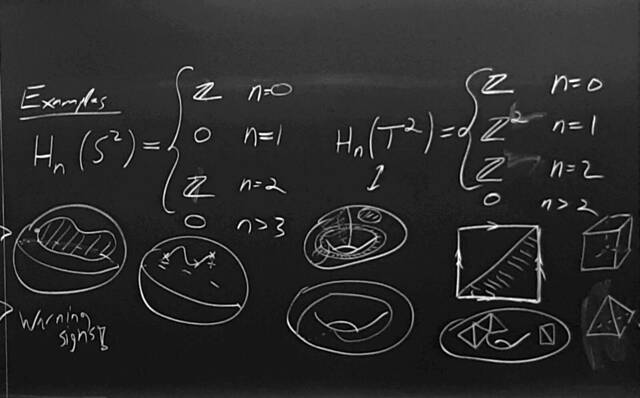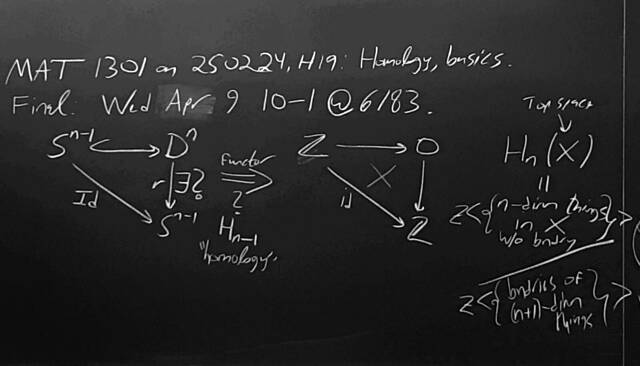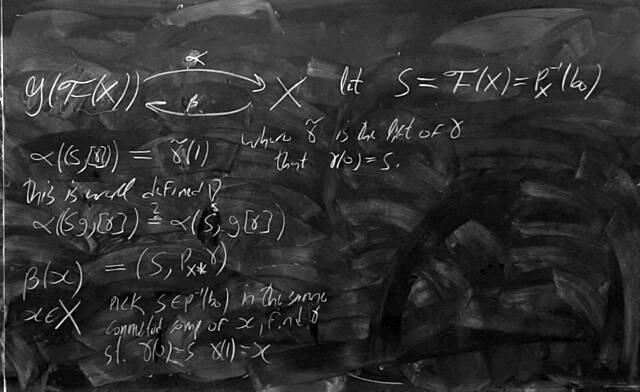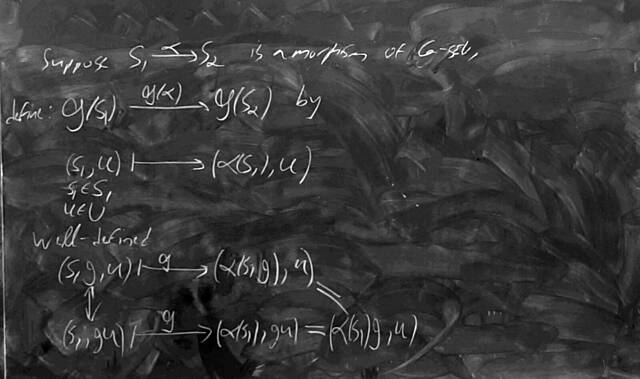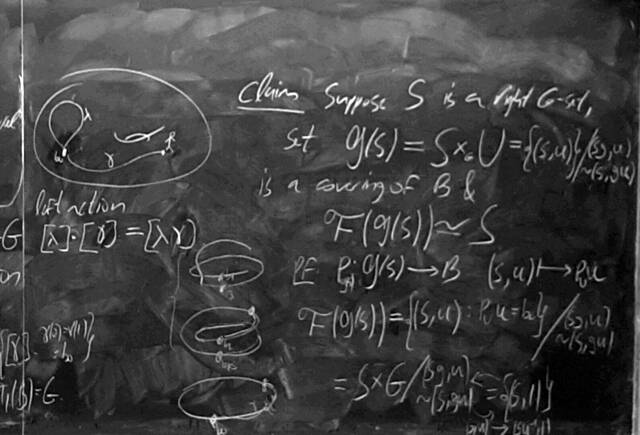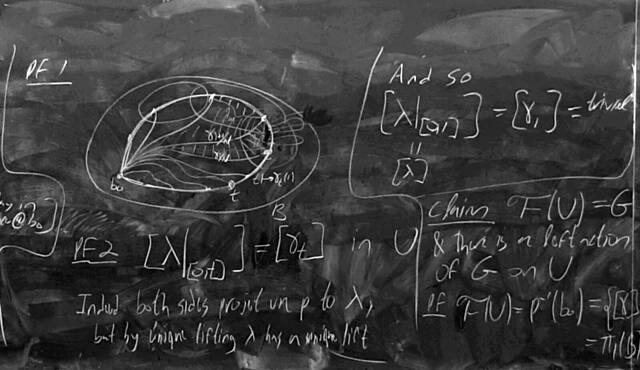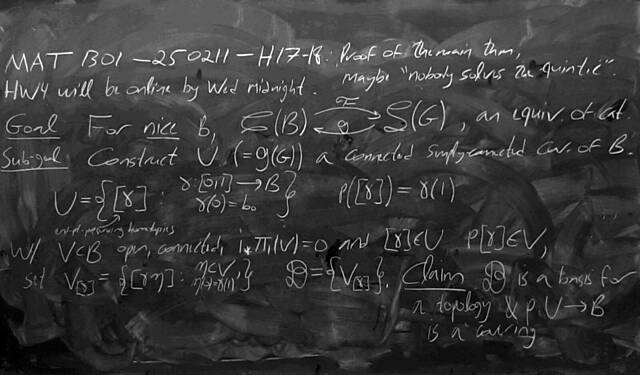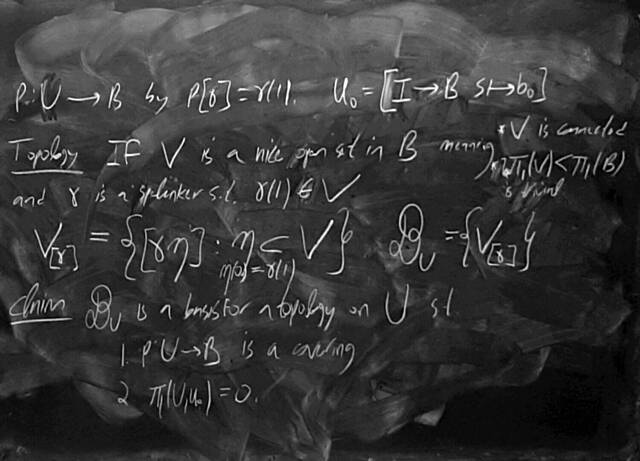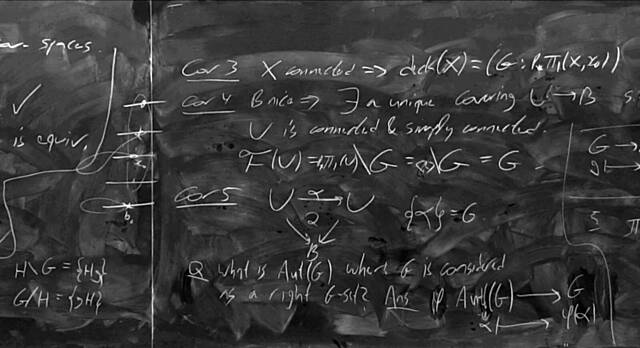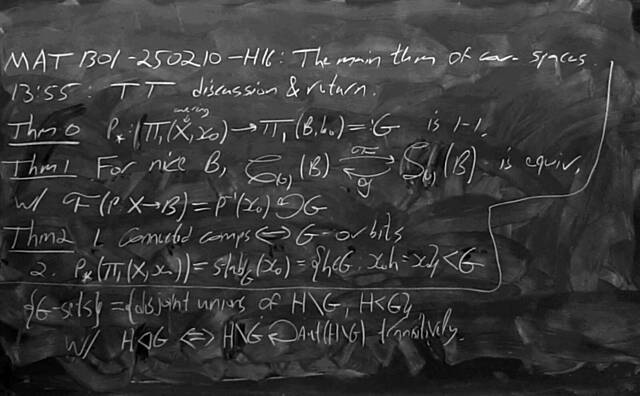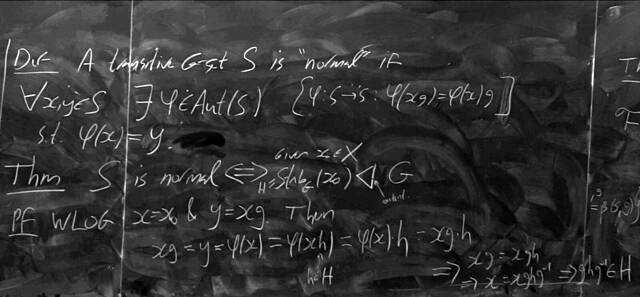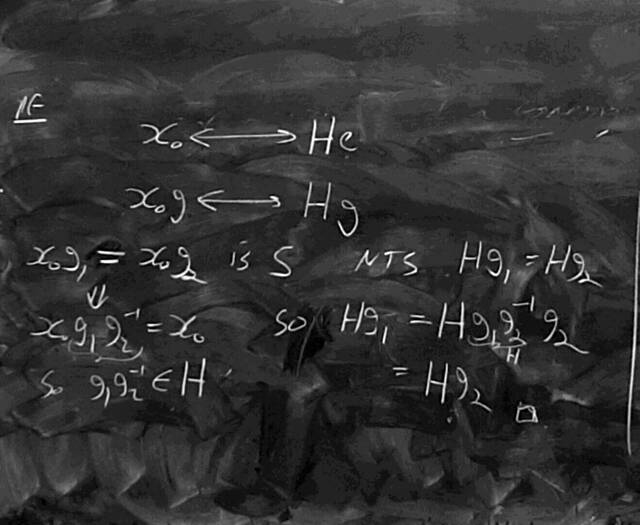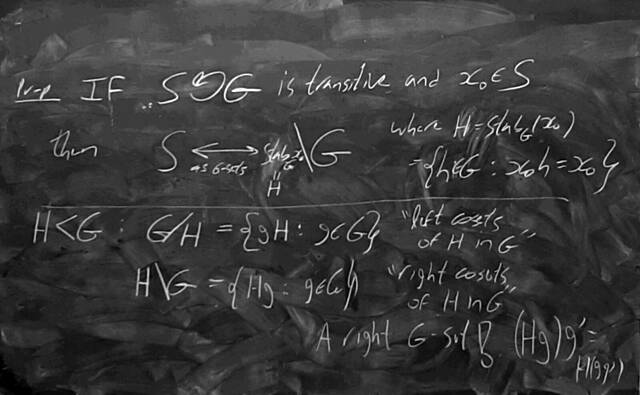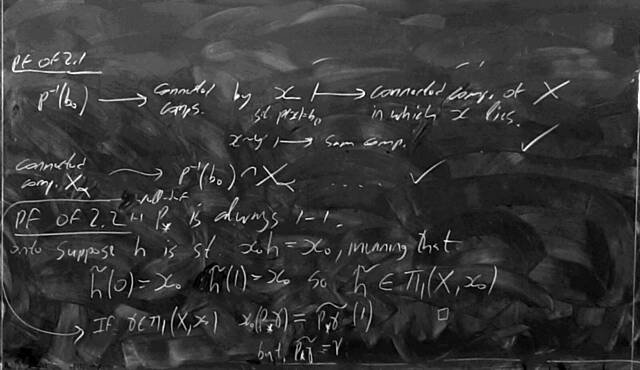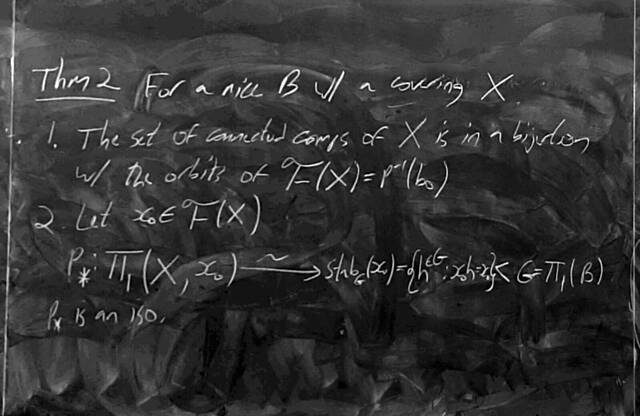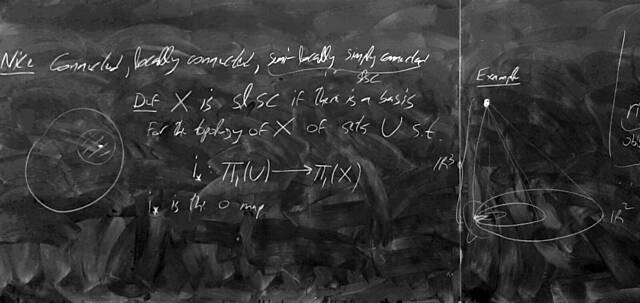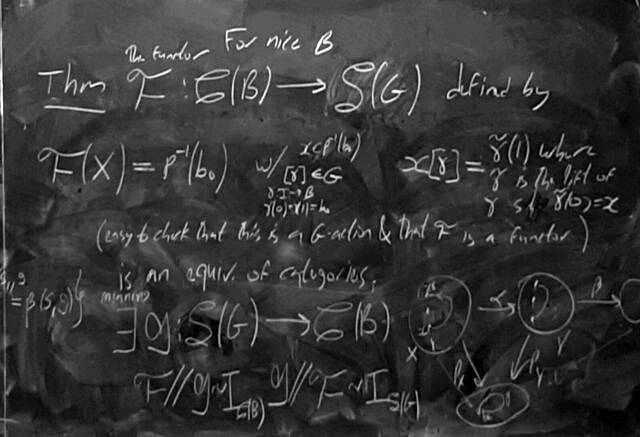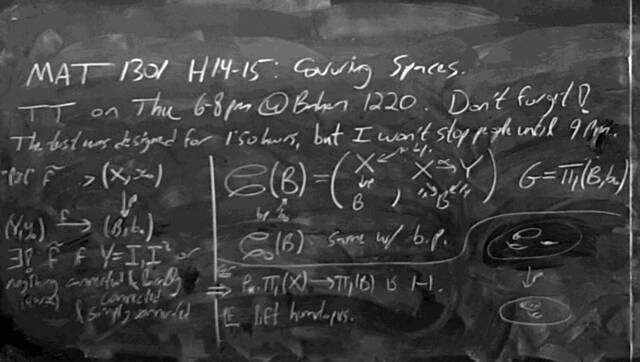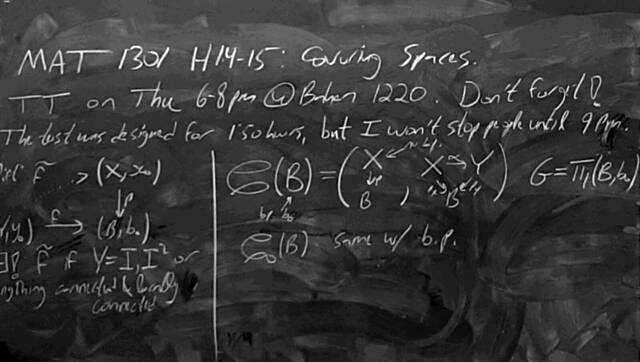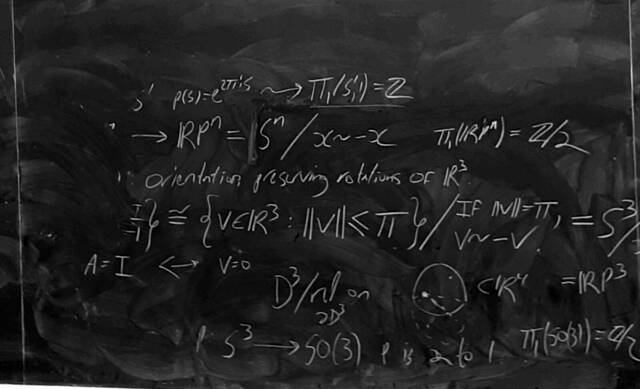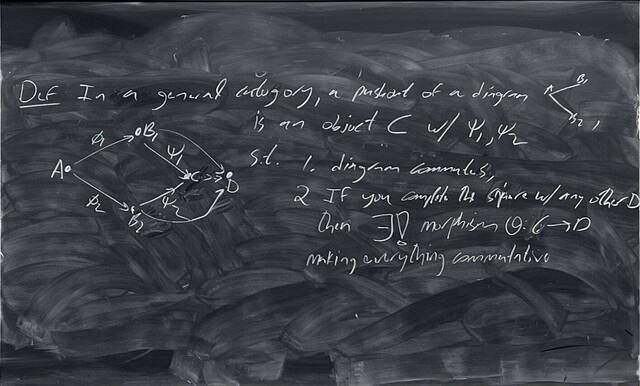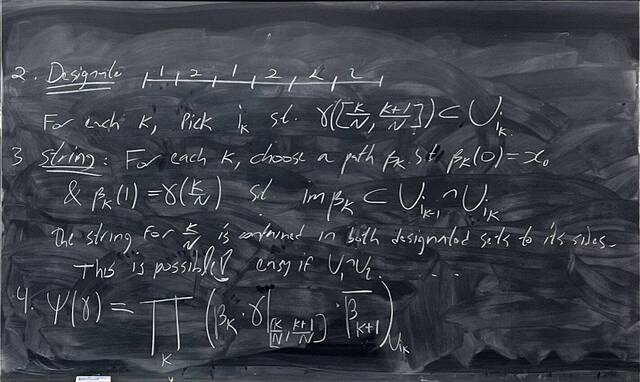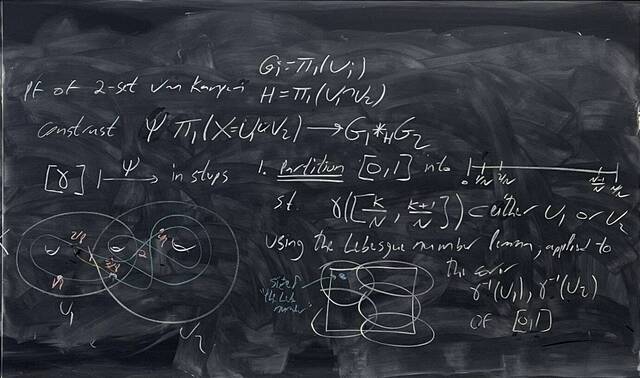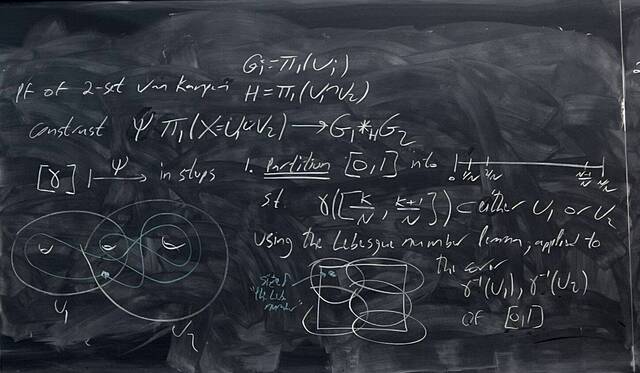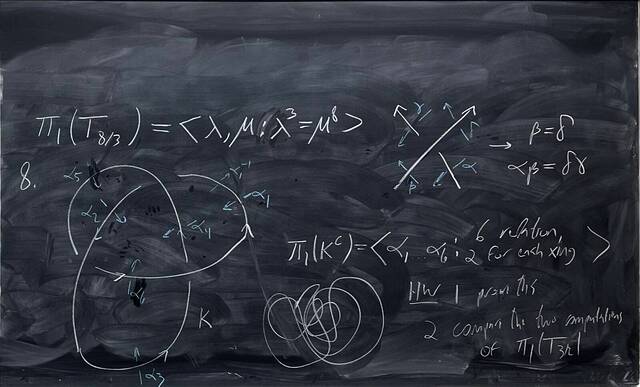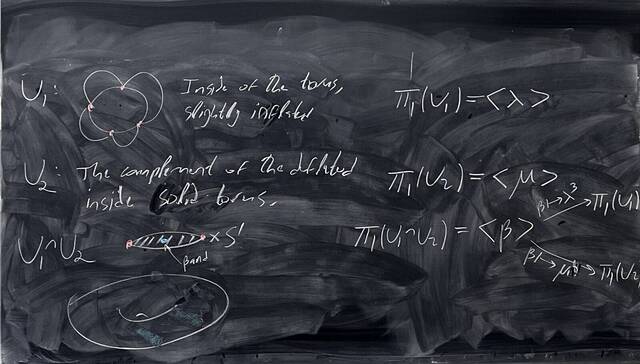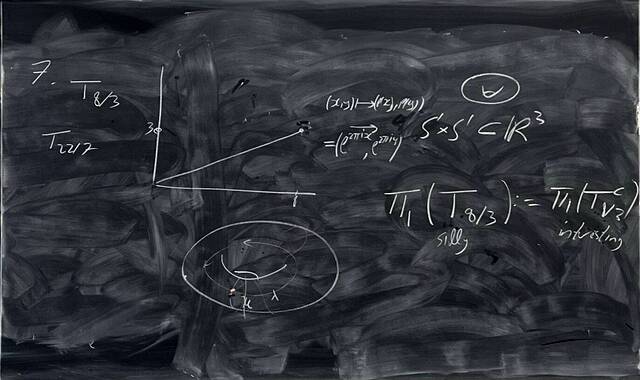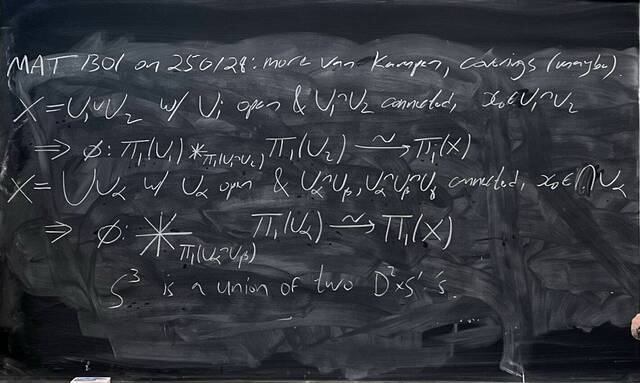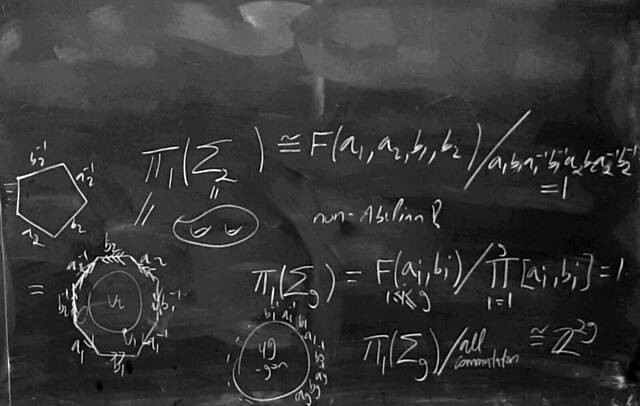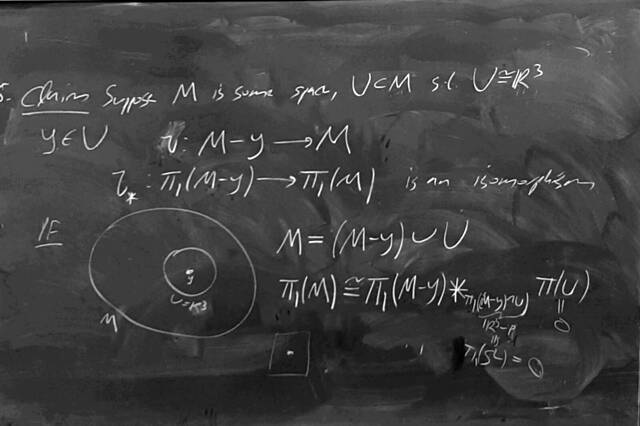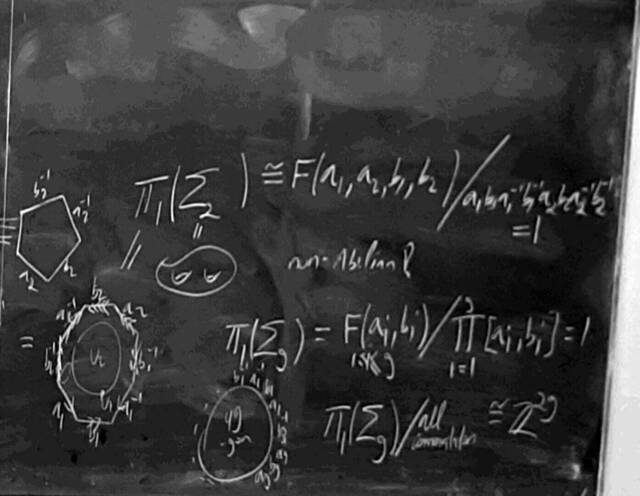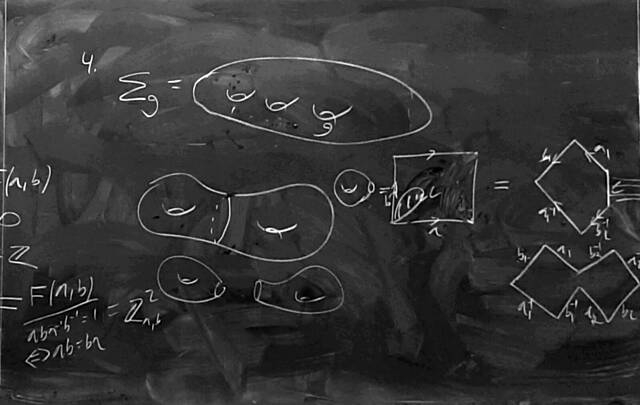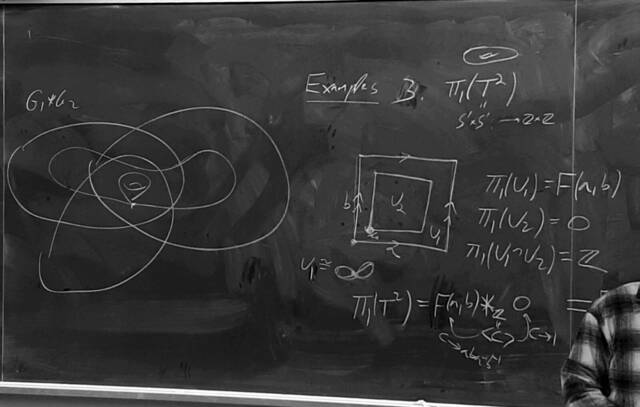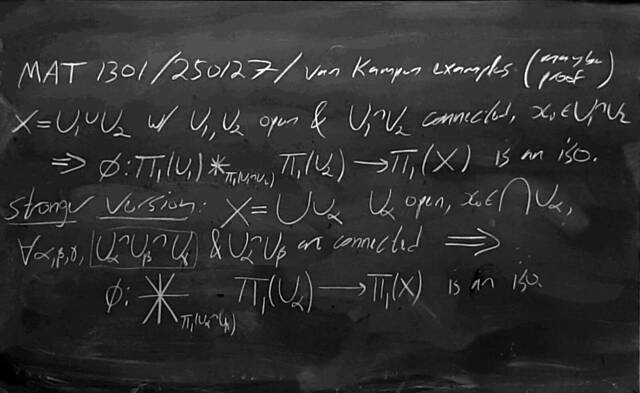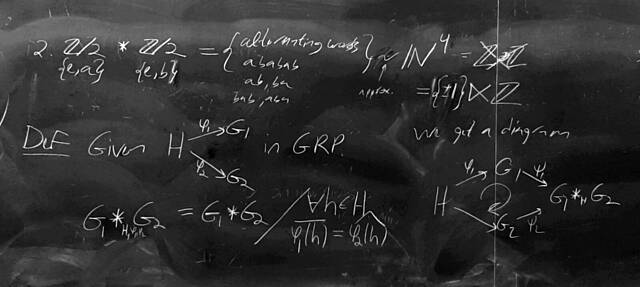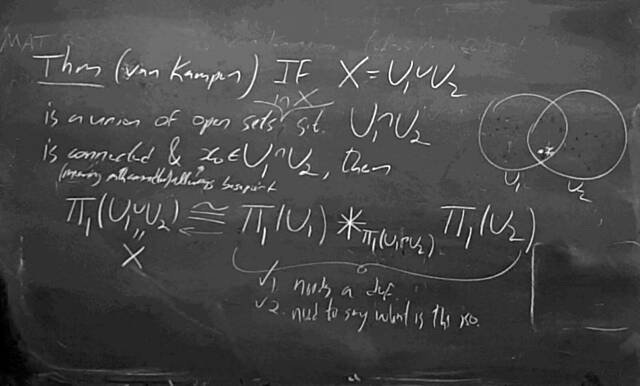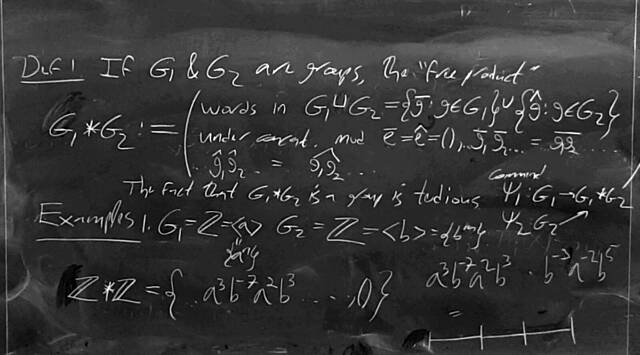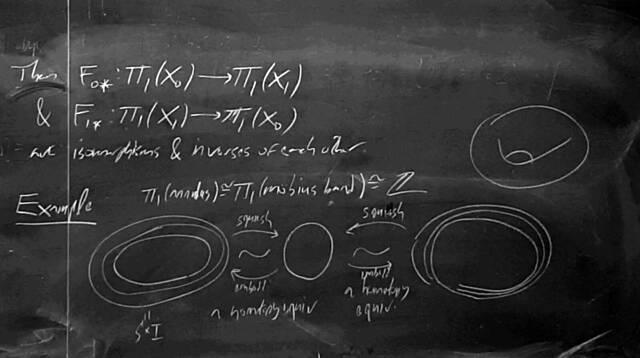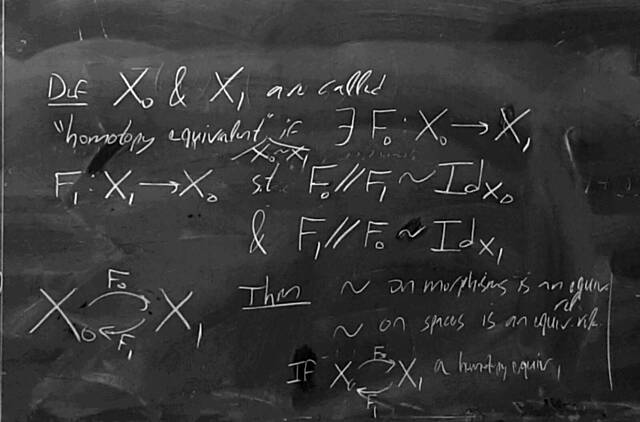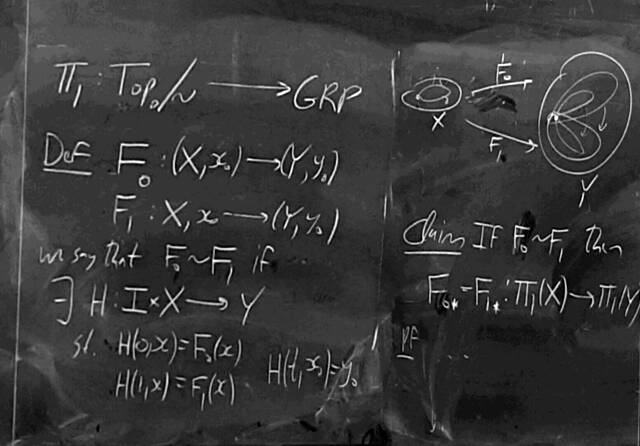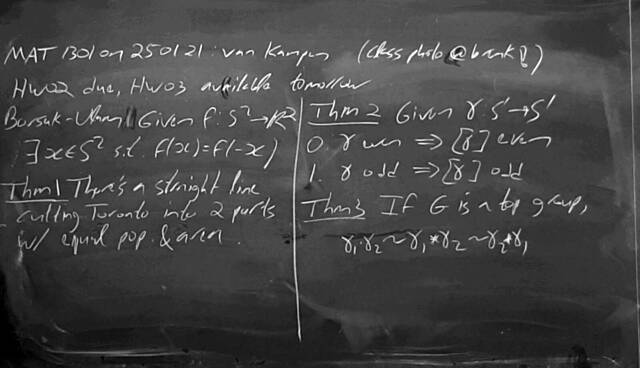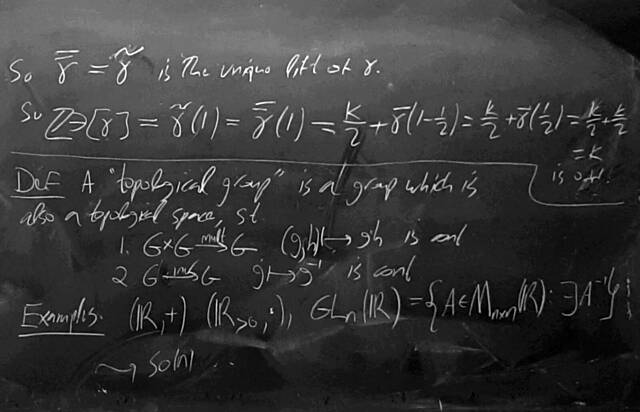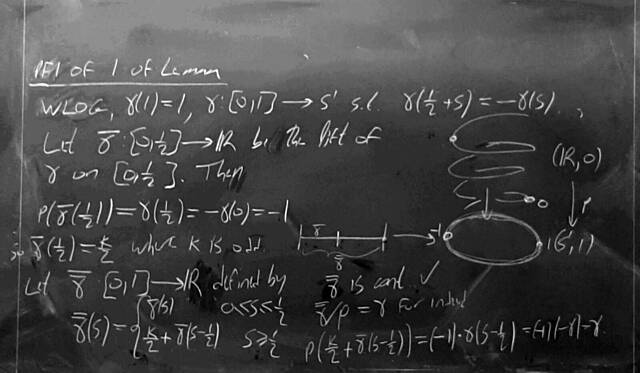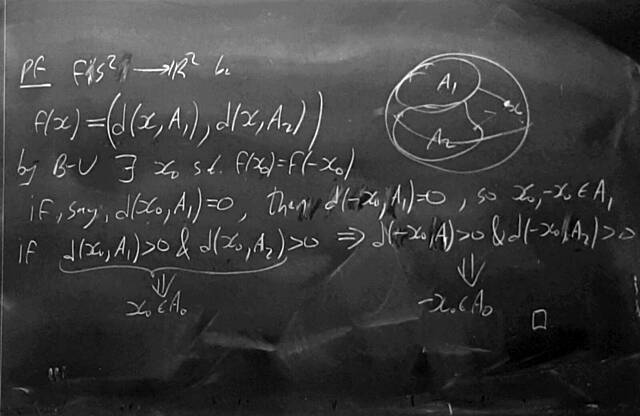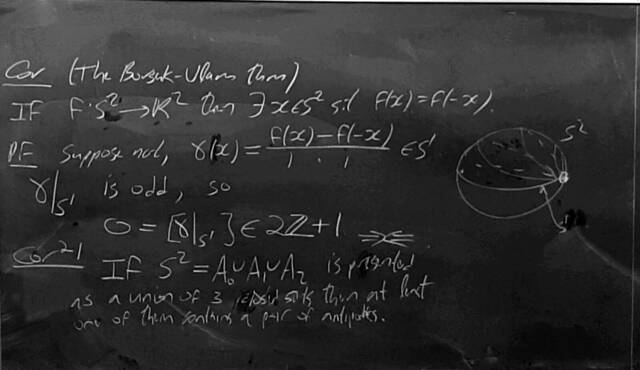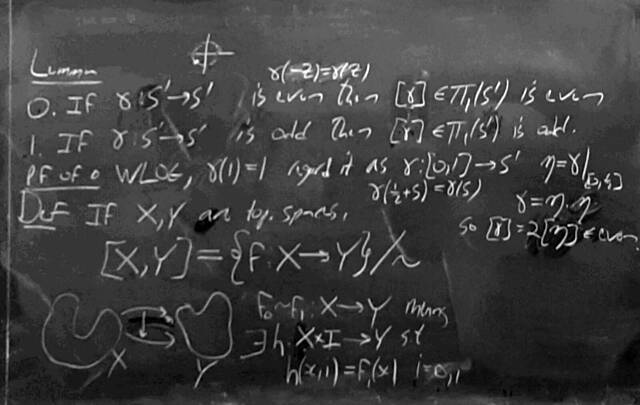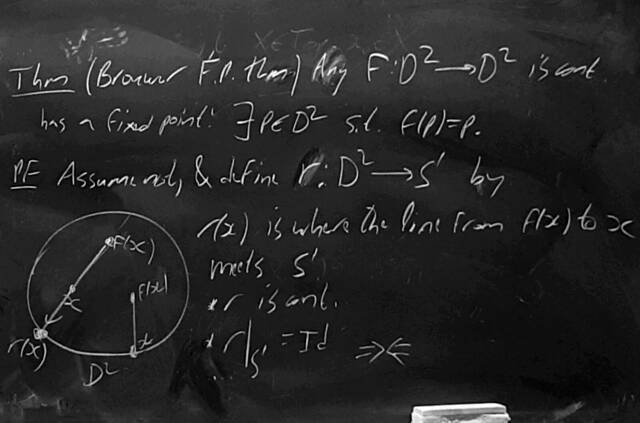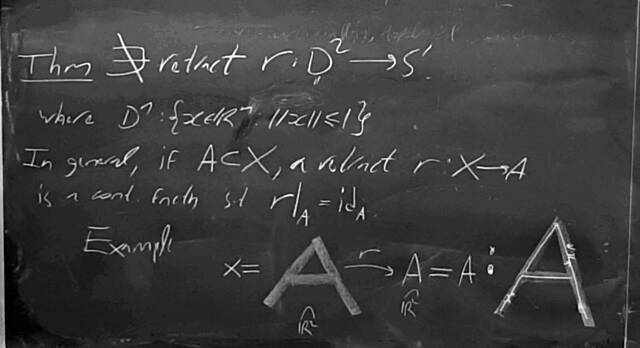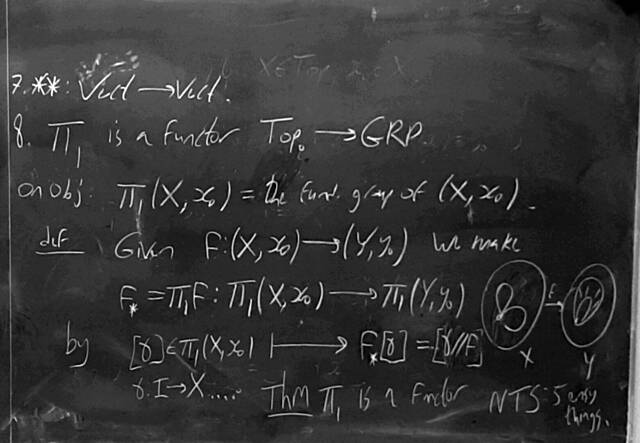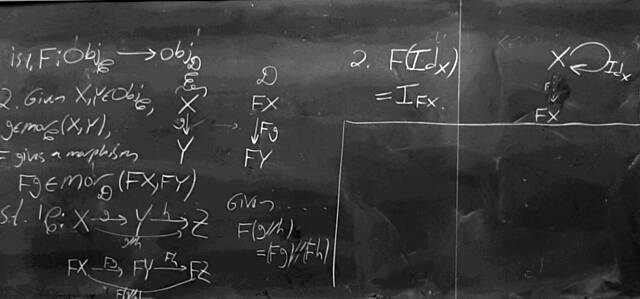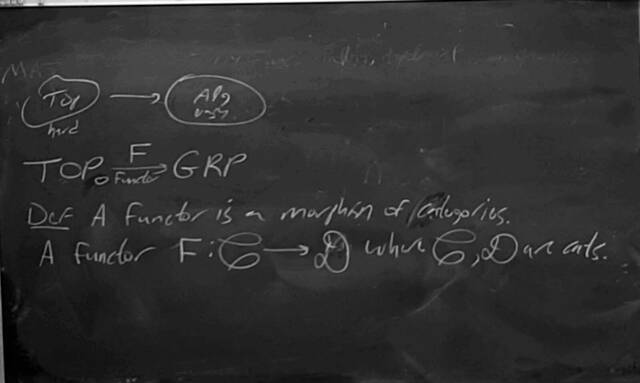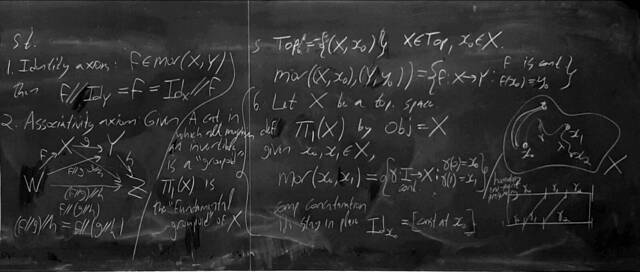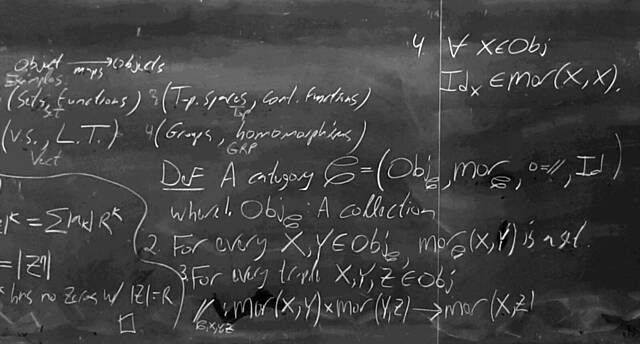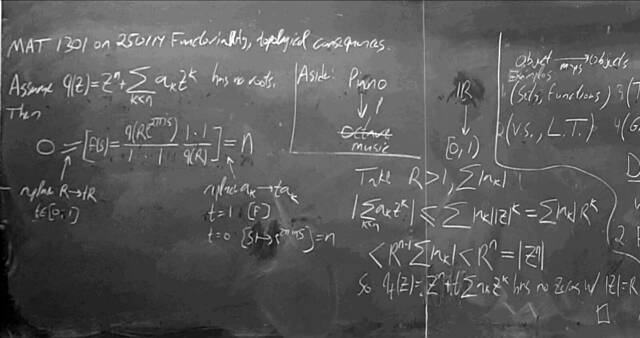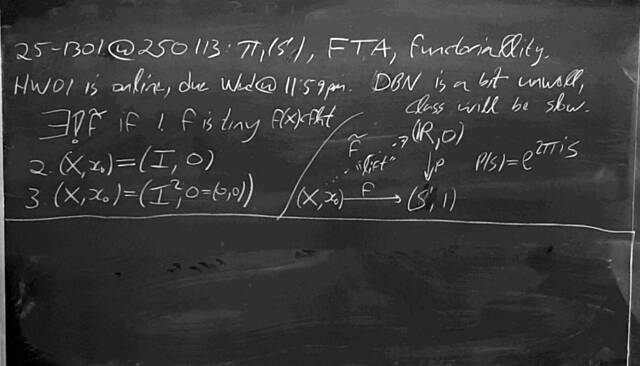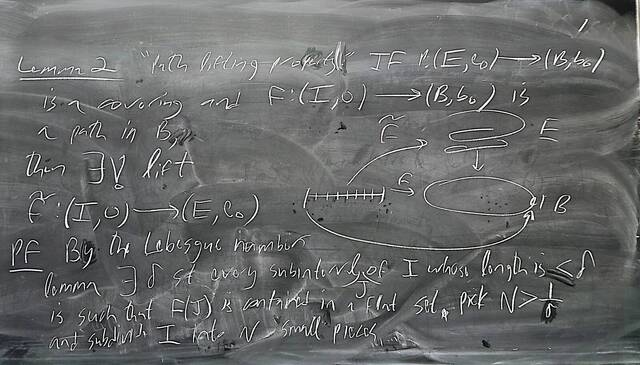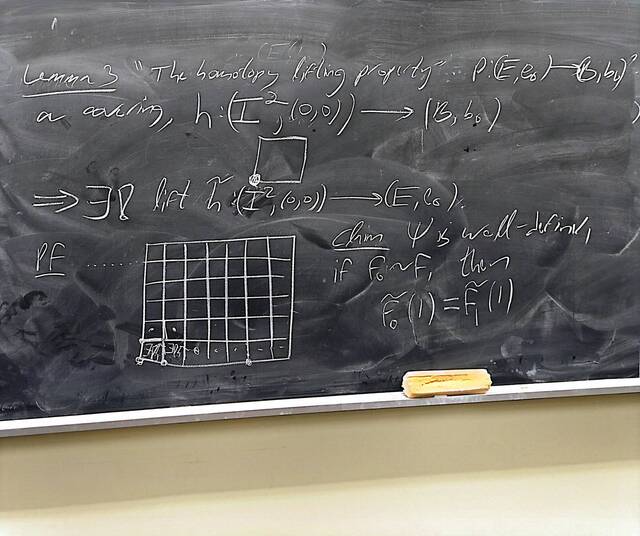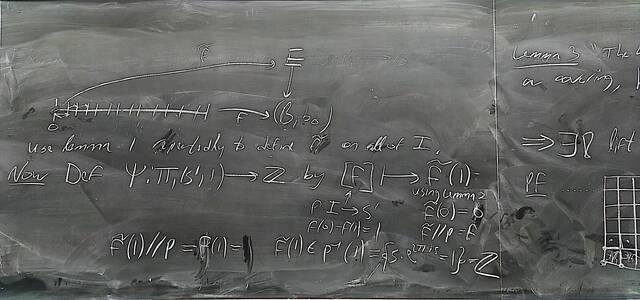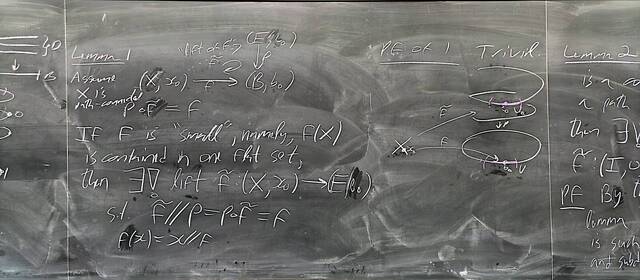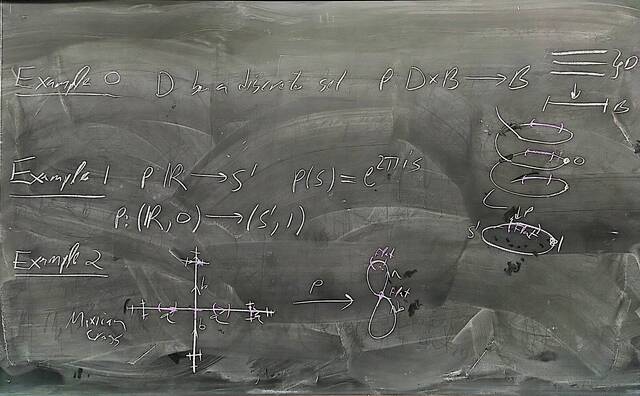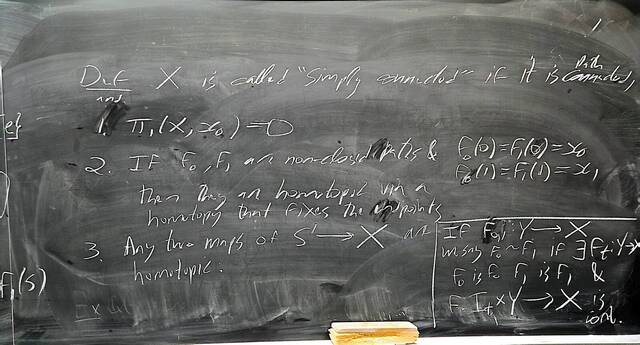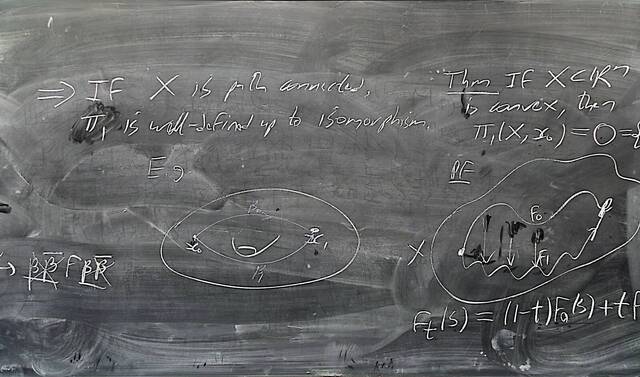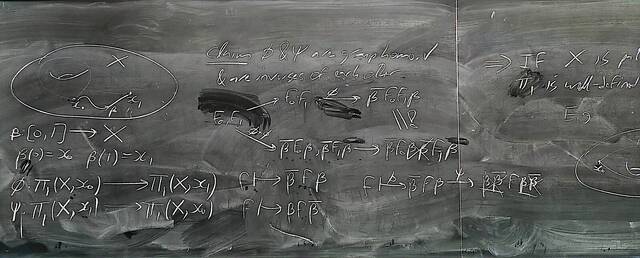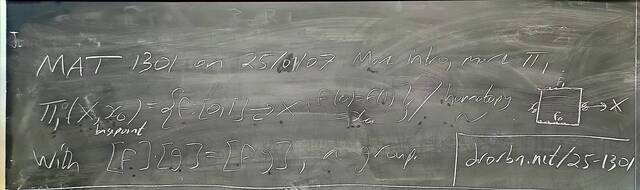Blackboard Shots with Prefix "25-1301"

250401-095204: Hour 34: Axiomatics (4).

250401-095203: Hour 34: Axiomatics (3).

250401-095202: Hour 34: Axiomatics (2).

250401-095201: Hour 34: Axiomatics.

250326-140557: Hours 32-33: Degrees, CW complexes (12).

250326-140556: Hours 32-33: Degrees, CW complexes (11).

250326-140555: Hours 32-33: Degrees, CW complexes (10).

250326-140554: Hours 32-33: Degrees, CW complexes (9).

250326-140553: Hours 32-33: Degrees, CW complexes (8).

250326-140552: Hours 32-33: Degrees, CW complexes (7).

250326-140551: Hours 32-33: Degrees, CW complexes (6).

250326-140550: Hours 32-33: Degrees, CW complexes (5).

250326-140549: Hours 32-33: Degrees, CW complexes (4).

250326-140548: Hours 32-33: Degrees, CW complexes (3).

250326-140547: Hours 32-33: Degrees, CW complexes (2).

250326-140546: Hours 32-33: Degrees, CW complexes.

250325-110650: Hour 31: Proof of Borsuk-Ulam (9).

250325-110649: Hour 31: Proof of Borsuk-Ulam (8).

250325-110648: Hour 31: Proof of Borsuk-Ulam (7).

250325-110647: Hour 31: Proof of Borsuk-Ulam (6).

250325-110646: Hour 31: Proof of Borsuk-Ulam (5).

250325-110645: Hour 31: Proof of Borsuk-Ulam (4).

250325-110644: Hour 31: Proof of Borsuk-Ulam (3).

250325-110643: Hour 31: Proof of Borsuk-Ulam (2).

250325-110642: Hour 31: Proof of Borsuk-Ulam.

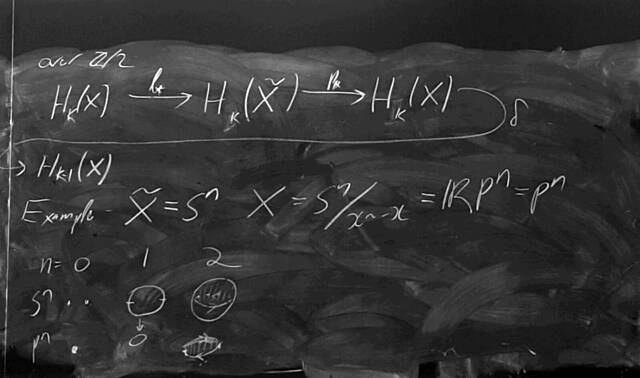250318-185347: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (15).

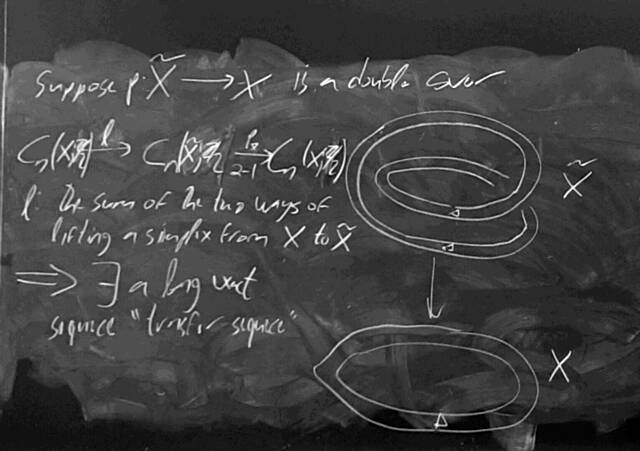250318-185346: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (14).

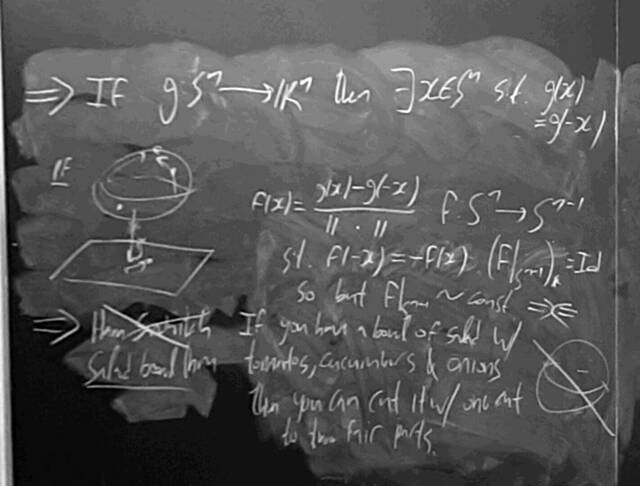250318-185345: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (13).

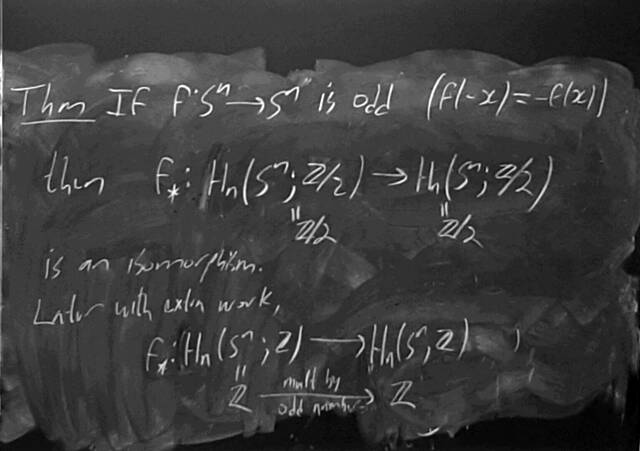250318-185344: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (12).

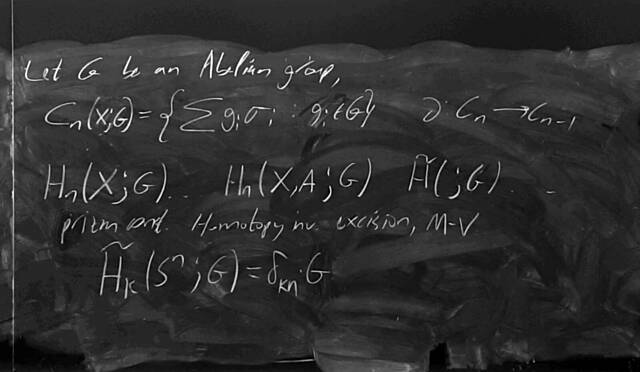250318-185343: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (11).

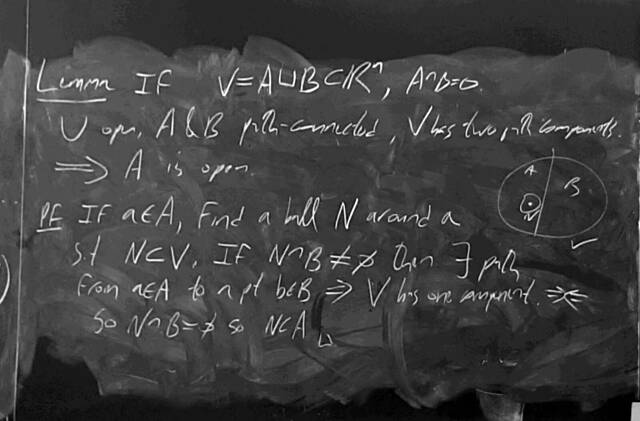250318-185342: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (10).

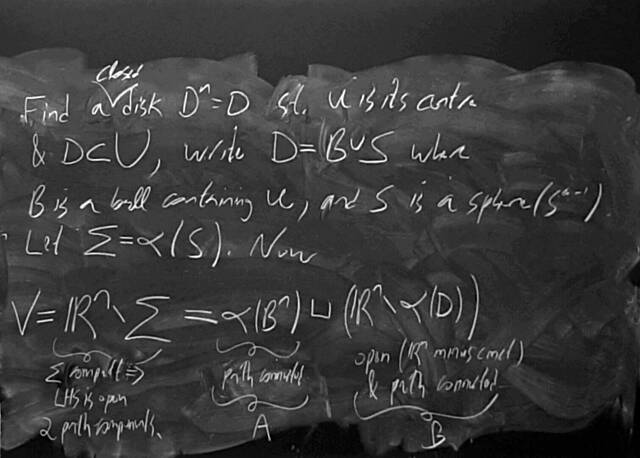250318-185341: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (9).

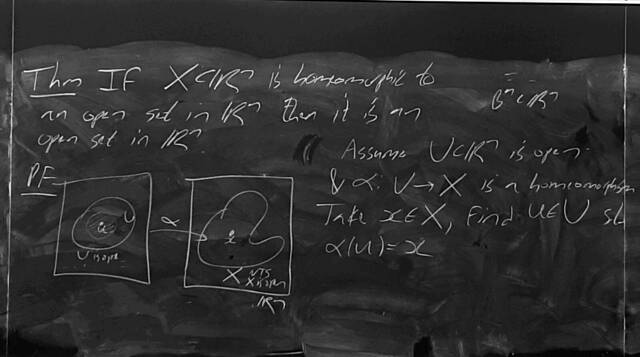250318-185340: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (8).

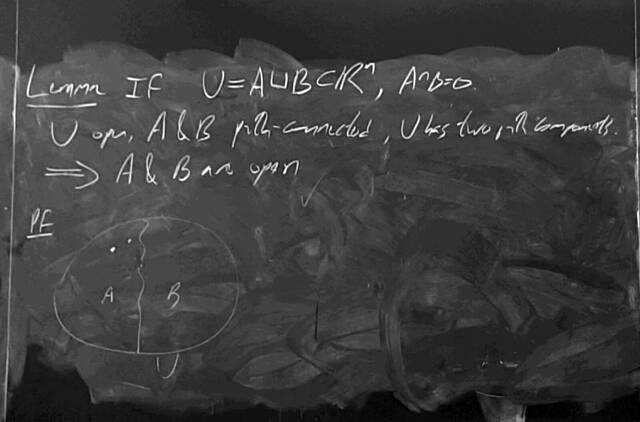250318-185339: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (7).

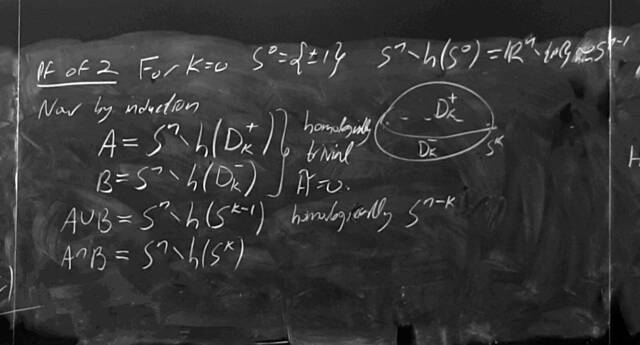250318-185338: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (6).

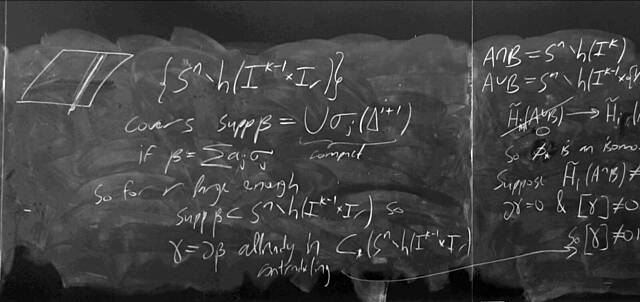250318-185337: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (5).

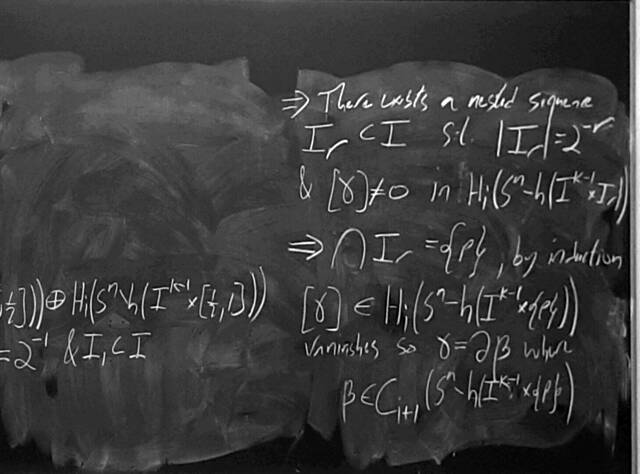250318-185336: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (4).

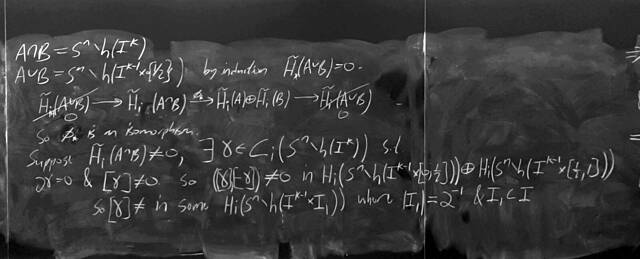250318-185335: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (3).

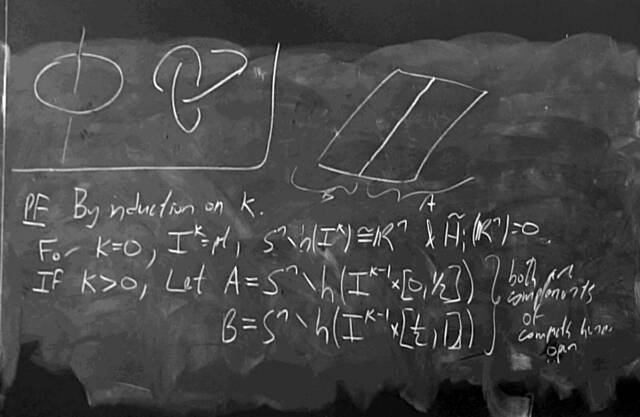250318-185334: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam (2).

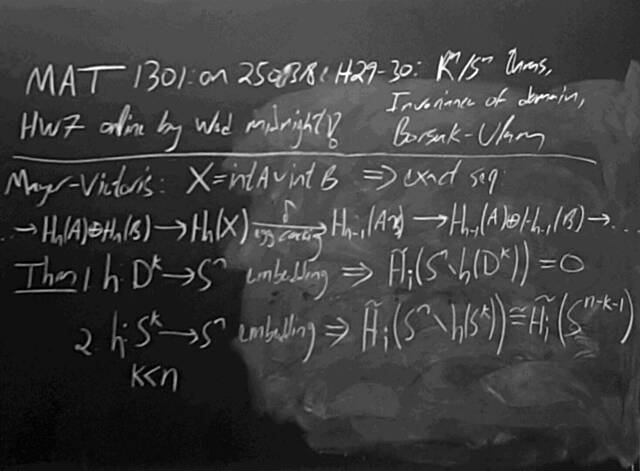250318-185333: Hours 29-30: The ${\mathbb R}^n/S^n$ theorems, invariance of domain, and Borsuk-Ulam.

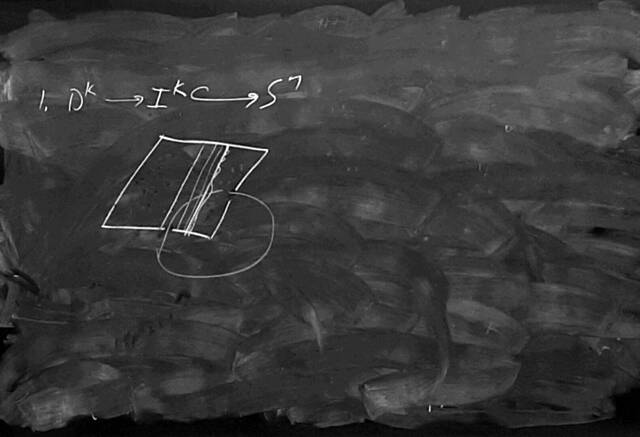250318-112344: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems (6).

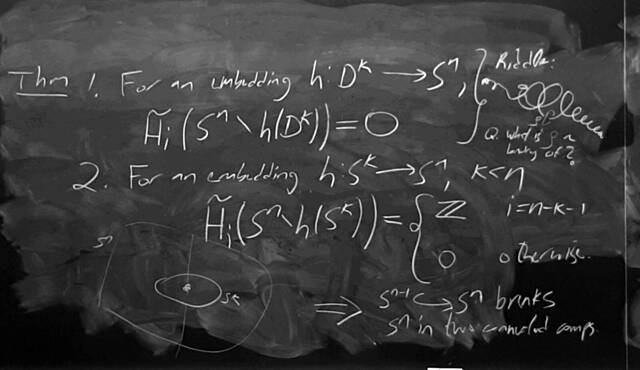250318-112343: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems (5).

250318-112342: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems (4).

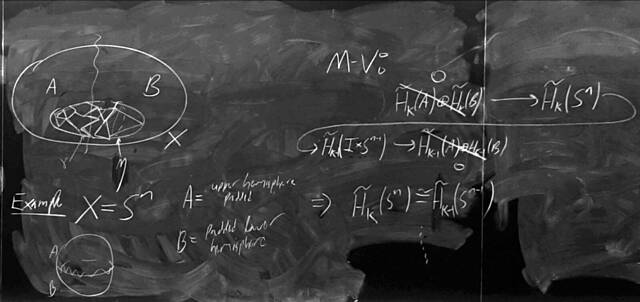250318-112341: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems (3).

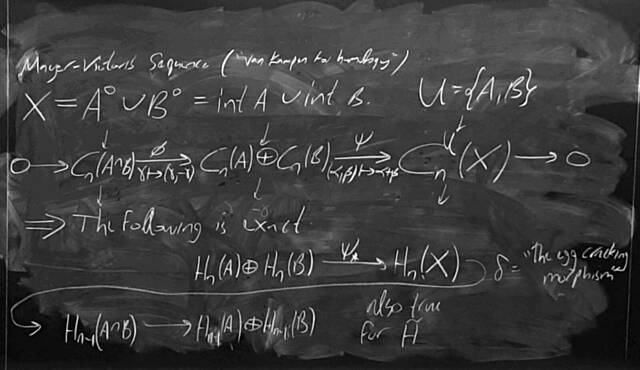250318-112340: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems (2).

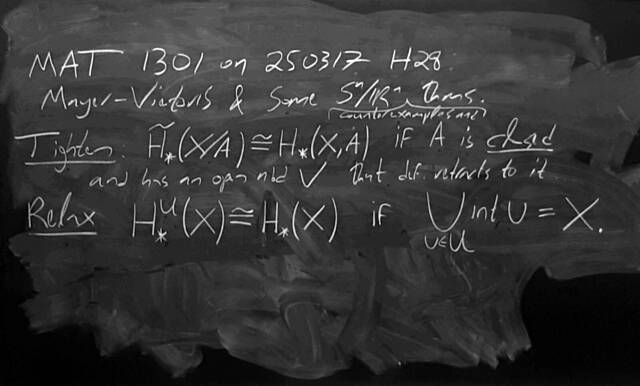250318-112339: Hour 28: Mayer-Vietoris and some ${\mathbb R}^n/S^n$ theorems.

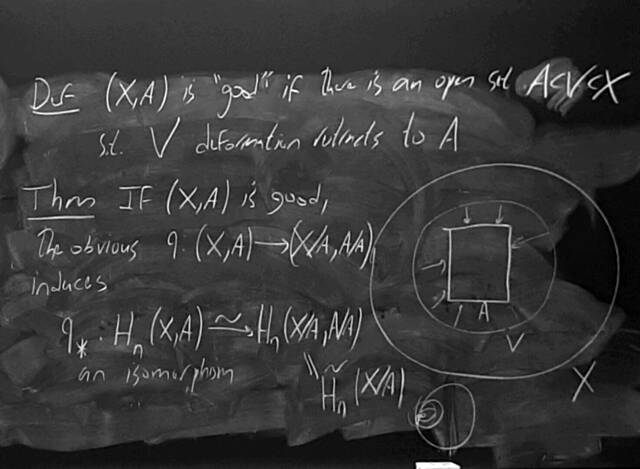250314-155310: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (10).

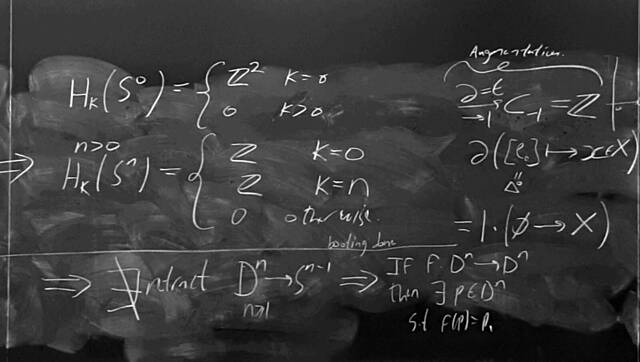250314-155309: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (9).

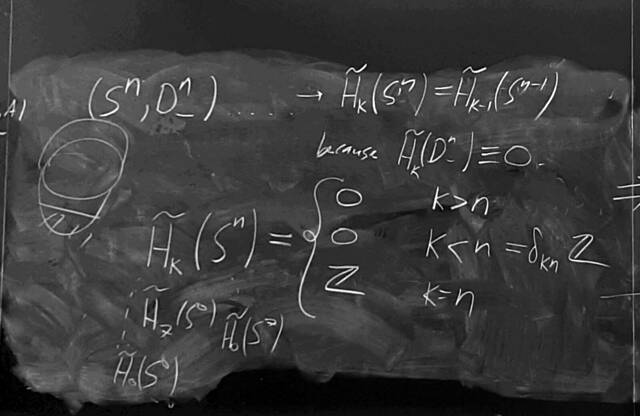250314-155308: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (8).

250314-155307: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (7).

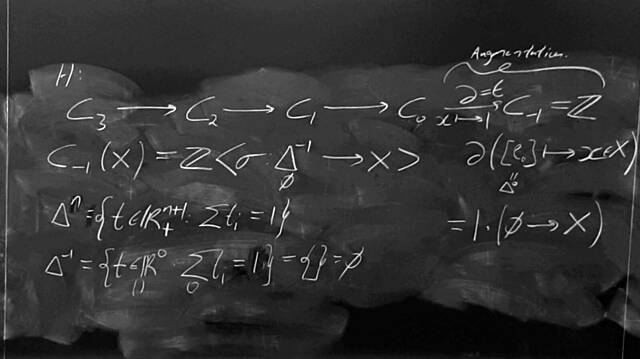250314-155306: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (6).

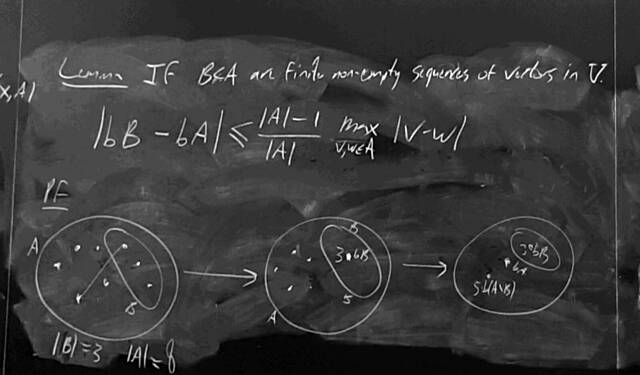250314-155305: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (5).

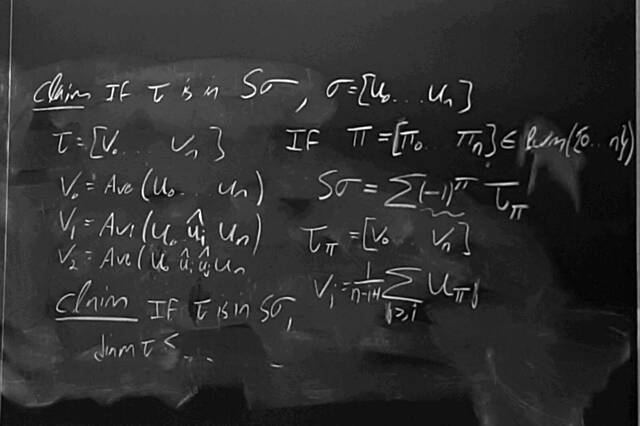250314-155304: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (4).

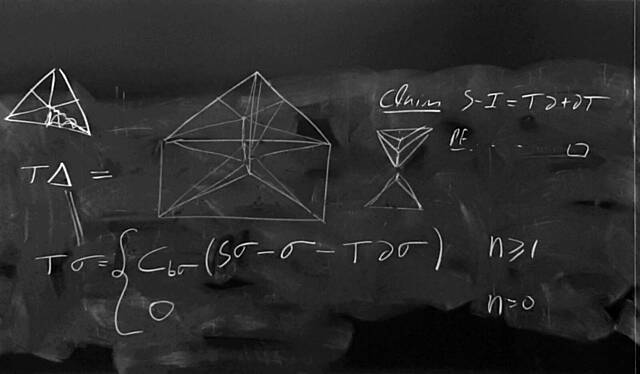250314-155303: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (3).

250314-155302: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology (2).

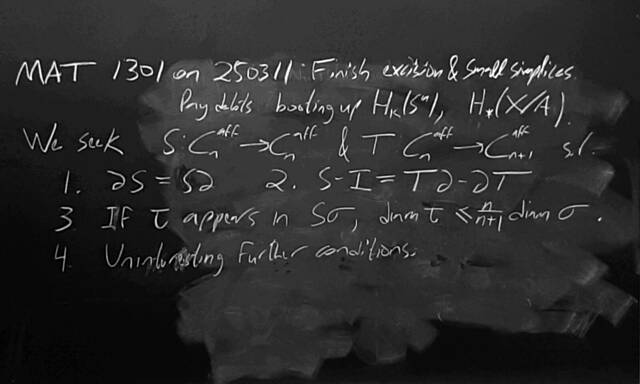250314-155301: Hours 26-27: barycentric details, reduced homology, quotients vs. relative homology.

250311-111221: Hour 25: More on excision (5).

250311-111220: Hour 25: More on excision (4).

250311-111219: Hour 25: More on excision (3).

250311-111218: Hour 25: More on excision (2).

250311-111217: Hour 25: More on excision.

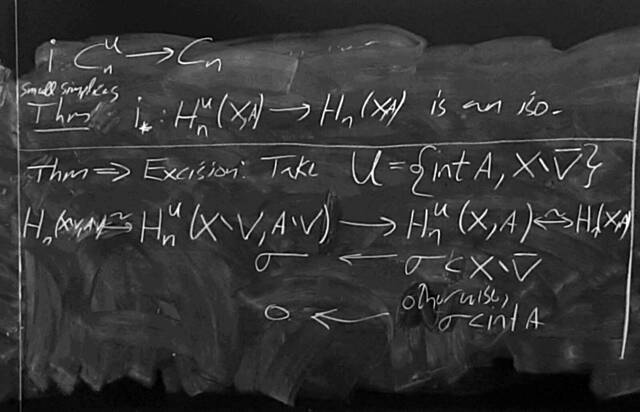250305-172545: Hours 23-24: short and long exact sequences, excision (12).

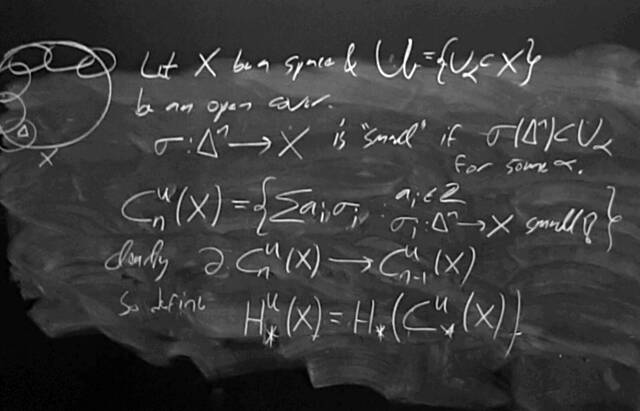250305-172544: Hours 23-24: short and long exact sequences, excision (11).

250305-172543: Hours 23-24: short and long exact sequences, excision (10).

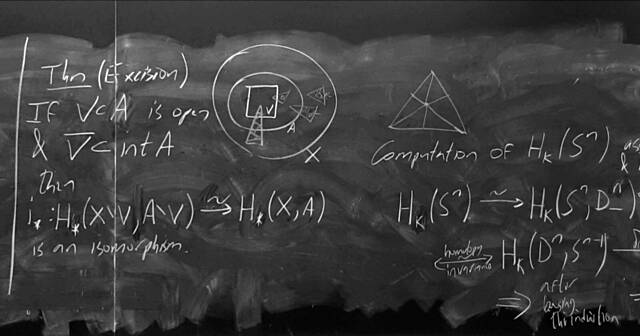250305-172542: Hours 23-24: short and long exact sequences, excision (9).

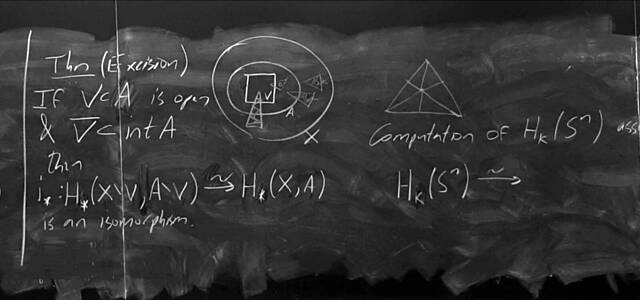250305-172541: Hours 23-24: short and long exact sequences, excision (8).

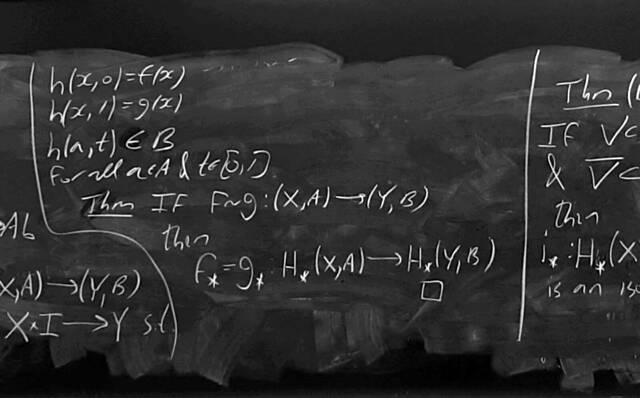250305-172540: Hours 23-24: short and long exact sequences, excision (7).

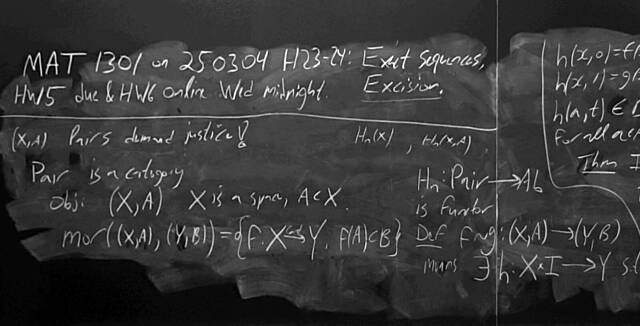250305-172539: Hours 23-24: short and long exact sequences, excision (6).

250305-172538: Hours 23-24: short and long exact sequences, excision (5).

250305-172537: Hours 23-24: short and long exact sequences, excision (4).

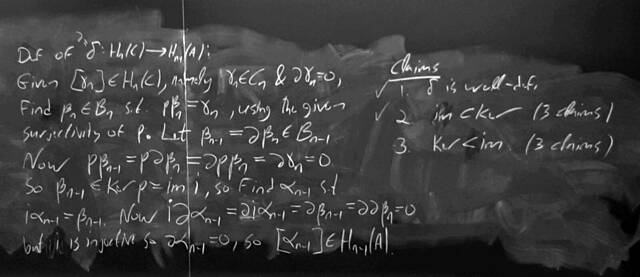250305-172536: Hours 23-24: short and long exact sequences, excision (3).

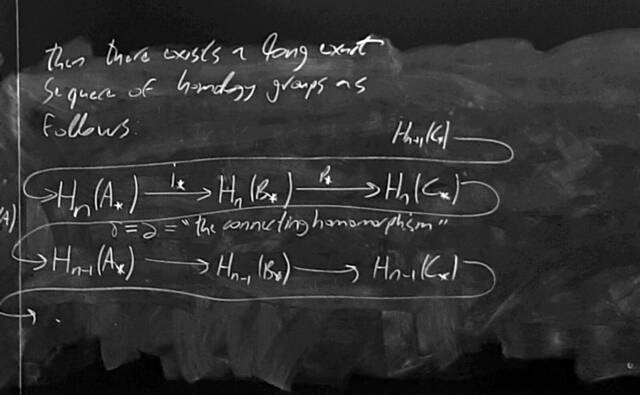250305-172535: Hours 23-24: short and long exact sequences, excision (2).

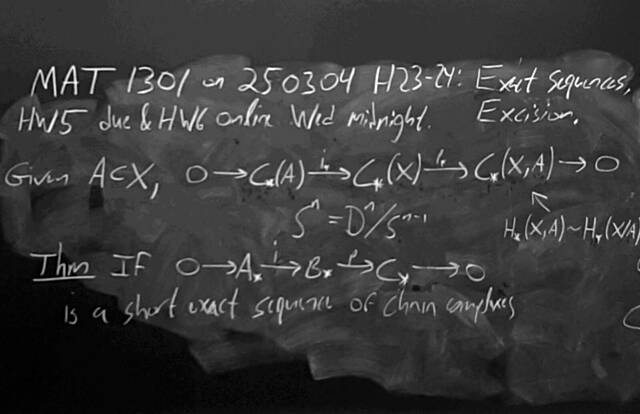250305-172534: Hours 23-24: short and long exact sequences, excision.

250303-143832: Hour 22: Prizms and exact sequences (6).

250303-143831: Hour 22: Prizms and exact sequences (5).

250303-143830: Hour 22: Prizms and exact sequences (4).

250303-143829: Hour 22: Prizms and exact sequences (3).

250303-143828: Hour 22: Prizms and exact sequences (2).

250303-143827: Hour 22: Prizms and exact sequences.

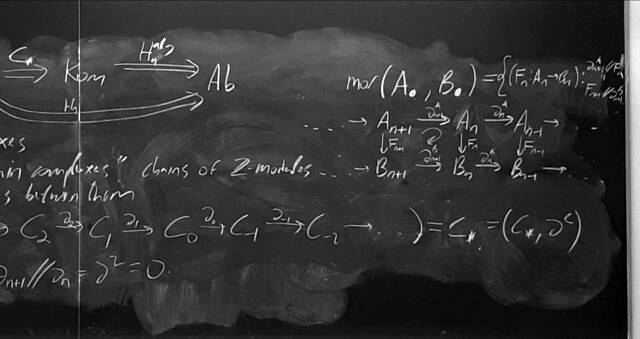250303-143312: Hours 20-21: Functoriallity, invariance under homotopy (7).

250303-143311: Hours 20-21: Functoriallity, invariance under homotopy (6).

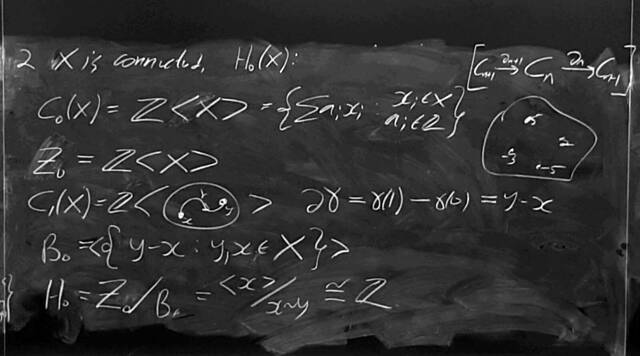250303-143310: Hours 20-21: Functoriallity, invariance under homotopy (5).

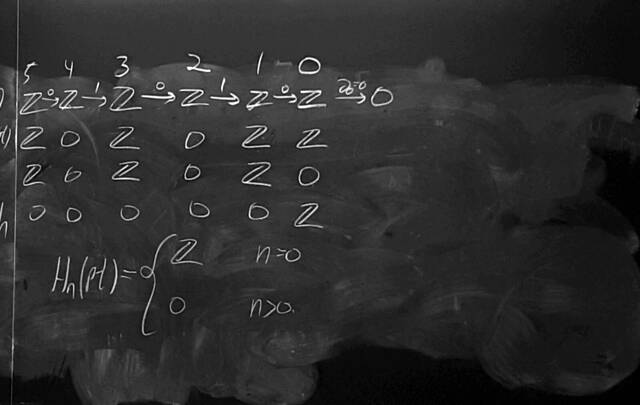250303-143309: Hours 20-21: Functoriallity, invariance under homotopy (4).

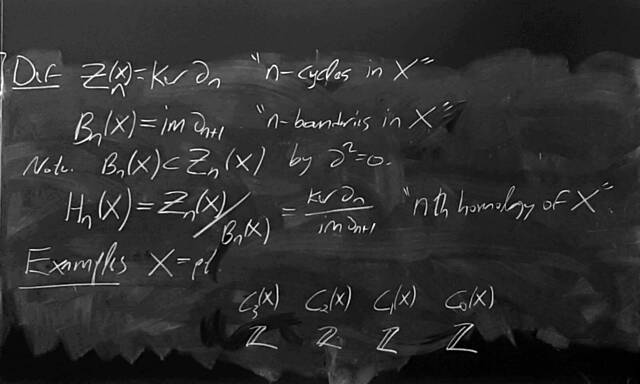250303-143308: Hours 20-21: Functoriallity, invariance under homotopy (3).

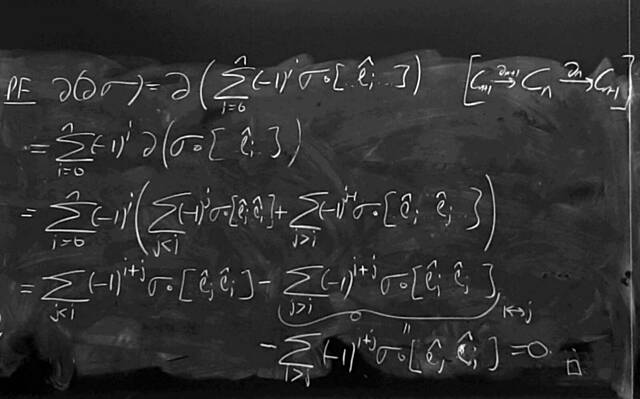250303-143307: Hours 20-21: Functoriallity, invariance under homotopy (2).

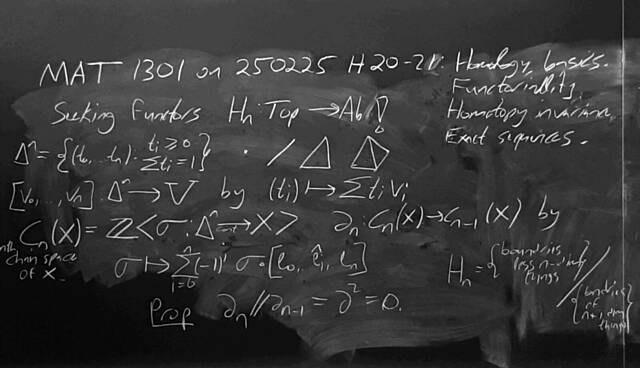250303-143306: Hours 20-21: Functoriallity, invariance under homotopy.

250224-165159: Hour 19: Homology basics (6).

250224-165158: Hour 19: Homology basics (5).

250224-165157: Hour 19: Homology basics (4).

250224-165156: Hour 19: Homology basics (3).

250224-165155: Hour 19: Homology basics (2).

250224-165154: Hour 19: Homology basics.

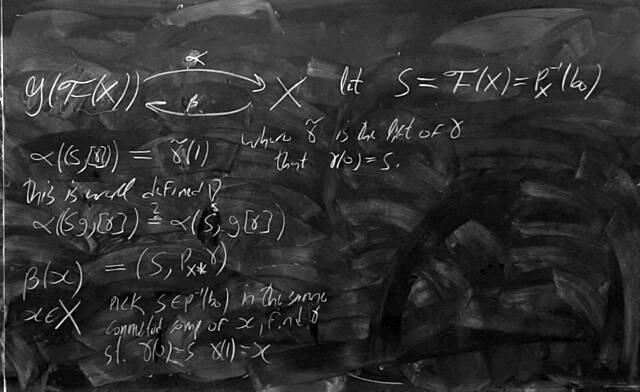250213-084759: Hours 17-18: Proof of the fundamental theorems on covering spaces (5).

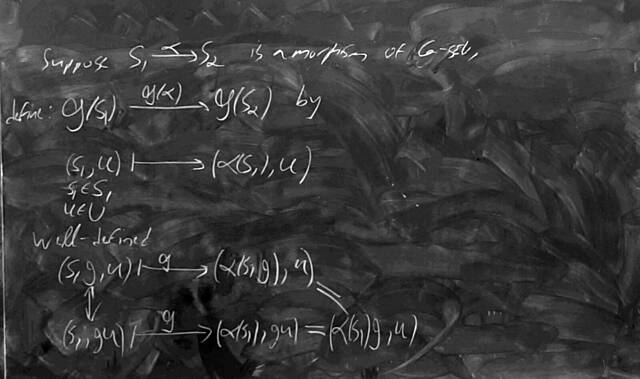250213-084758: Hours 17-18: Proof of the fundamental theorems on covering spaces (4).

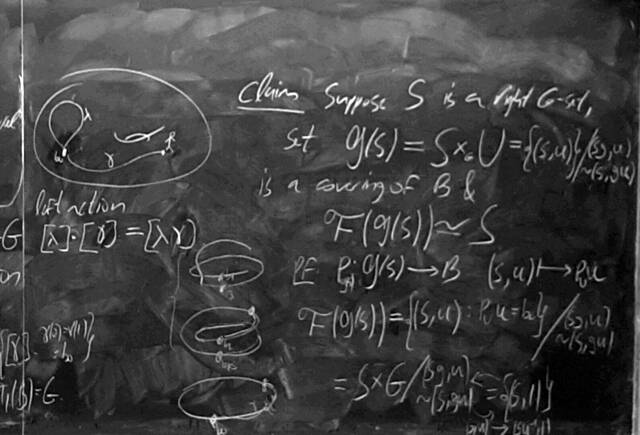250213-084757: Hours 17-18: Proof of the fundamental theorems on covering spaces (3).

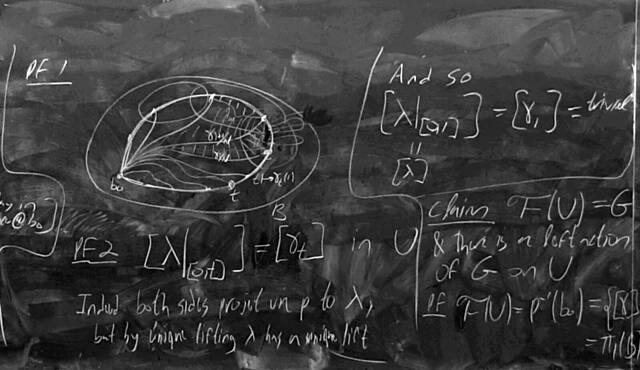250213-084756: Hours 17-18: Proof of the fundamental theorems on covering spaces (2).

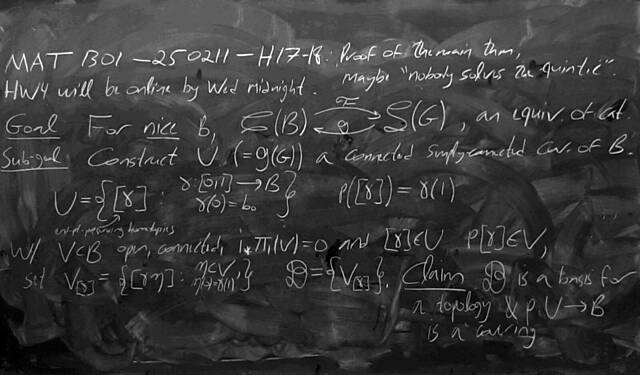250213-084755: Hours 17-18: Proof of the fundamental theorems on covering spaces.

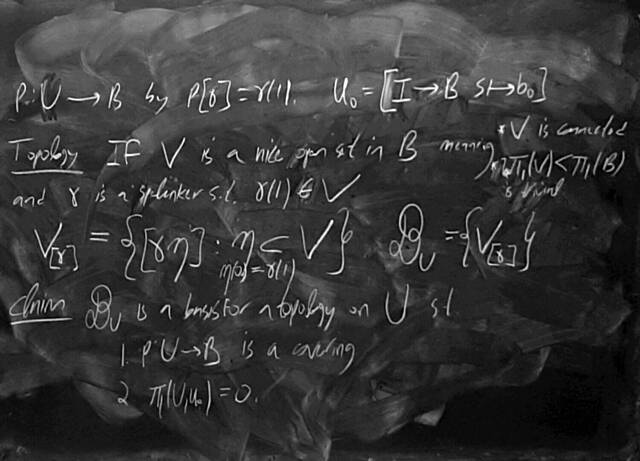250213-084628: Hour 16: Proof of the fundamental theorems on covering spaces (4).

250213-084627: Hour 16: Proof of the fundamental theorems on covering spaces (3).

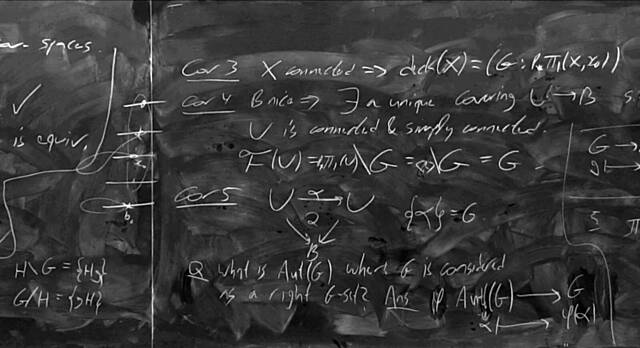250213-084626: Hour 16: Proof of the fundamental theorems on covering spaces (2).

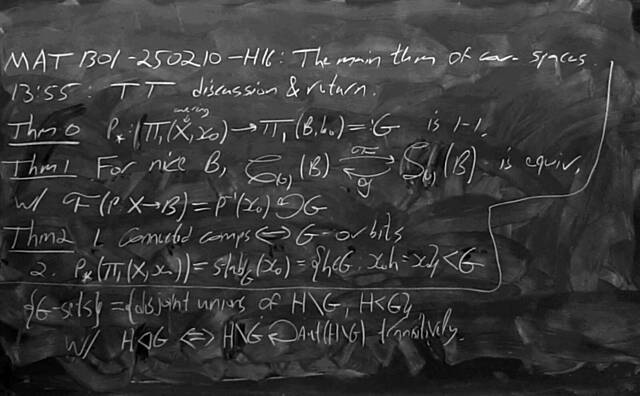250213-084625: Hour 16: Proof of the fundamental theorems on covering spaces.

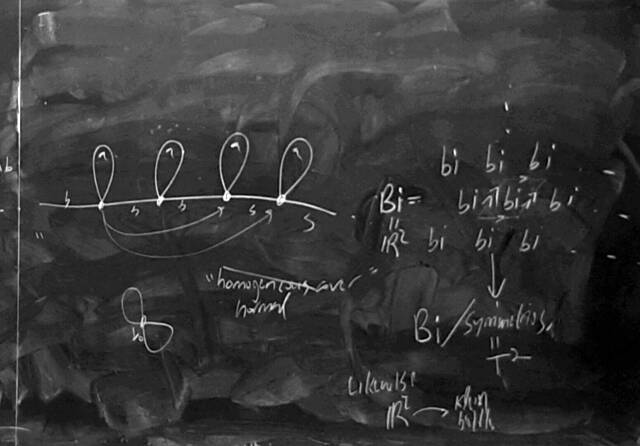
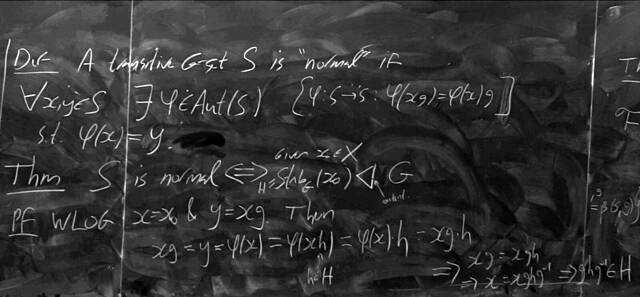250206-073359: Hours 14-15: The fundamental theorems on covering spaces (10).

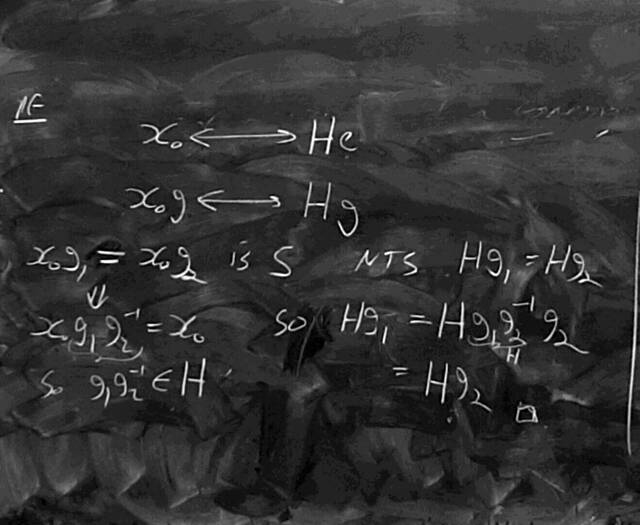250206-073358: Hours 14-15: The fundamental theorems on covering spaces (9).

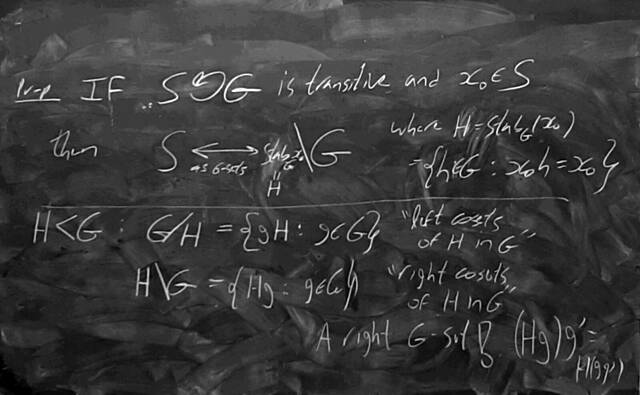250206-073357: Hours 14-15: The fundamental theorems on covering spaces (8).

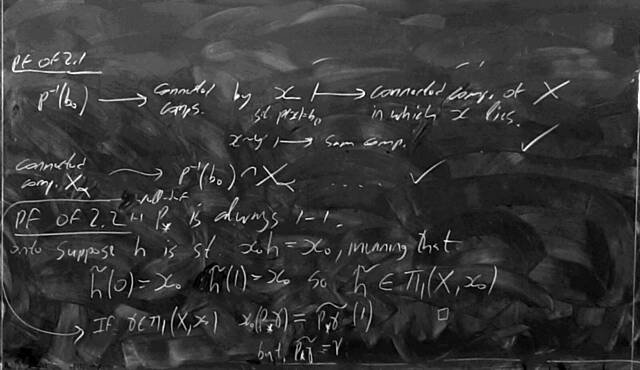250206-073356: Hours 14-15: The fundamental theorems on covering spaces (7).

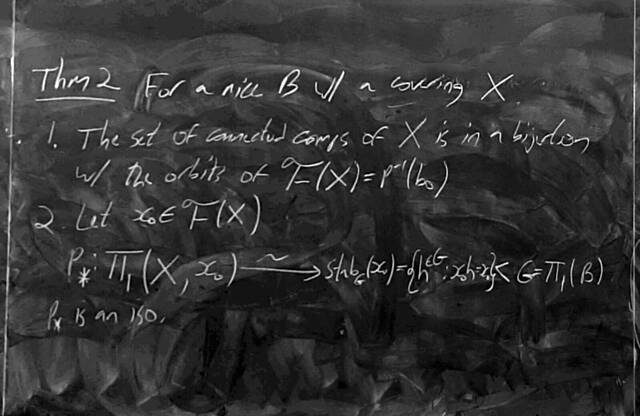250206-073355: Hours 14-15: The fundamental theorems on covering spaces (6).

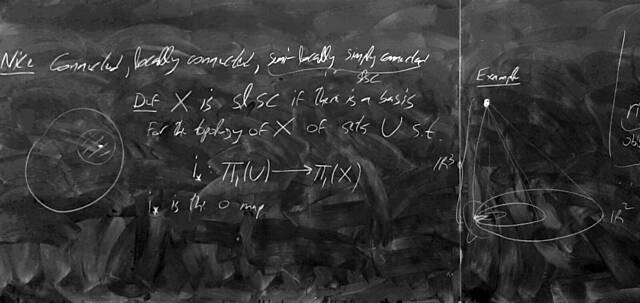250206-073354: Hours 14-15: The fundamental theorems on covering spaces (5).

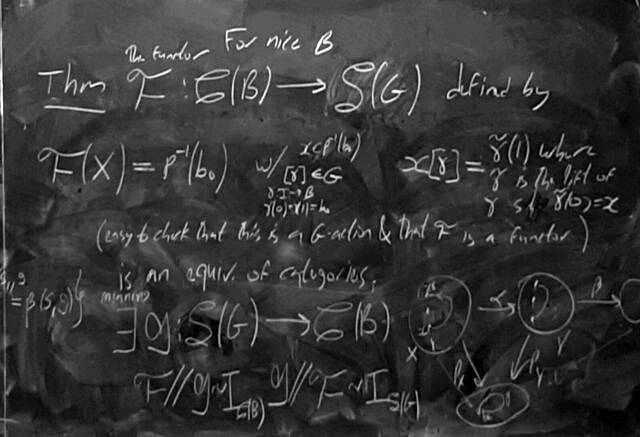250206-073353: Hours 14-15: The fundamental theorems on covering spaces (4).

250206-073352: Hours 14-15: The fundamental theorems on covering spaces (3).

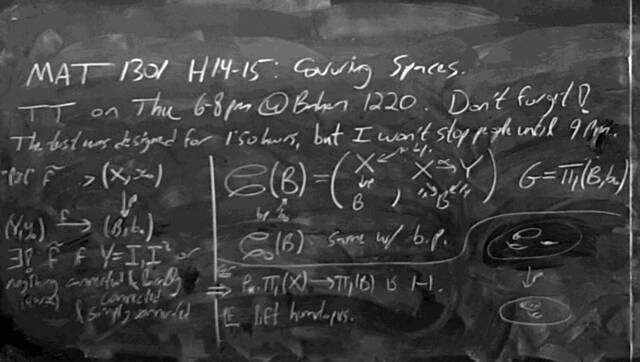250206-073351: Hours 14-15: The fundamental theorems on covering spaces (2).

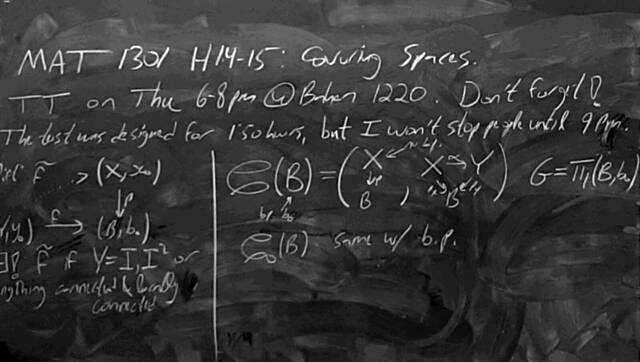250206-073350: Hours 14-15: The fundamental theorems on covering spaces.

250206-072721: Hour 13: Examples of covering spaces (4).

250206-072720: Hour 13: Examples of covering spaces (3).

250206-072719: Hour 13: Examples of covering spaces (2).

250206-072718: Hour 13: Examples of covering spaces.

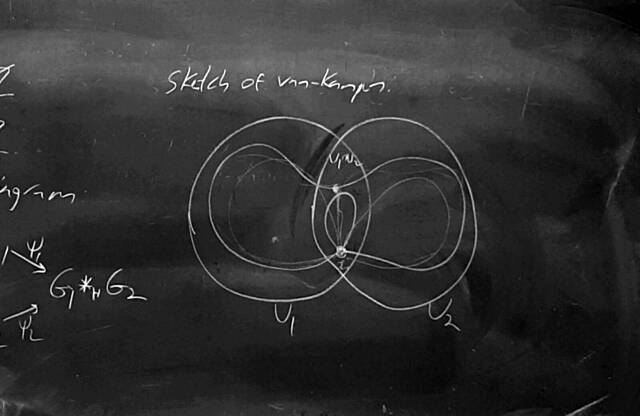
250130-154049: Hours 11-12: More van Kampen examples; proof of van Kampen (11).

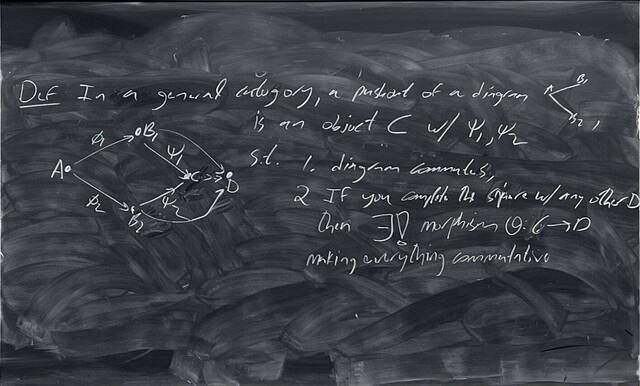250130-154048: Hours 11-12: More van Kampen examples; proof of van Kampen (10).

250130-154047: Hours 11-12: More van Kampen examples; proof of van Kampen (9).

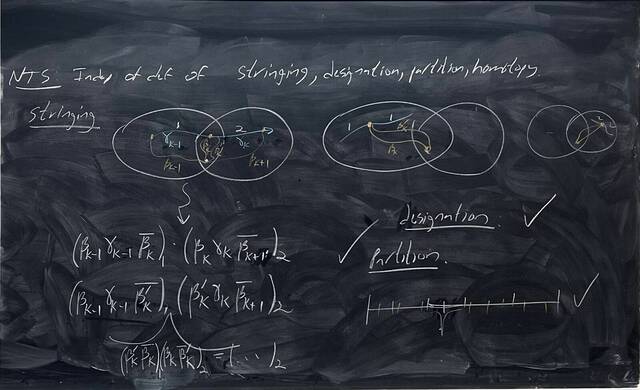250130-154046: Hours 11-12: More van Kampen examples; proof of van Kampen (8).

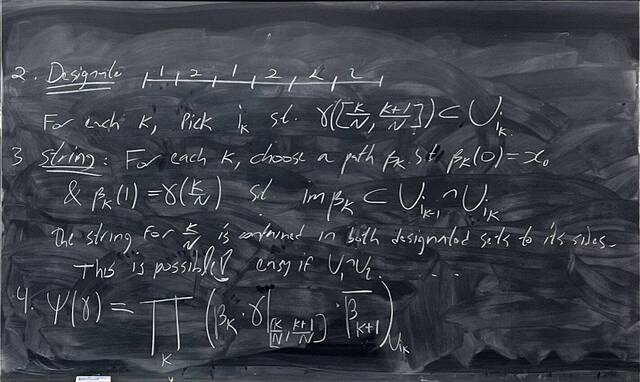250130-154045: Hours 11-12: More van Kampen examples; proof of van Kampen (7).

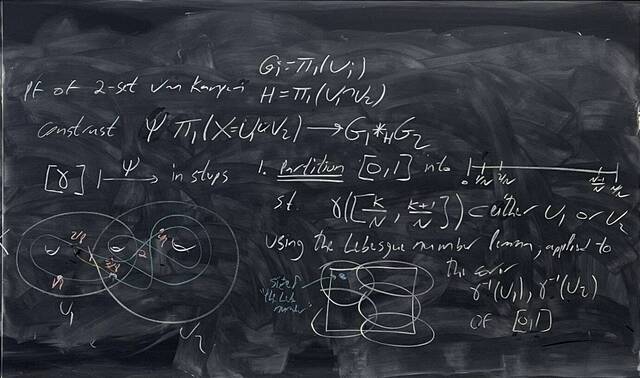250130-154044: Hours 11-12: More van Kampen examples; proof of van Kampen (6).

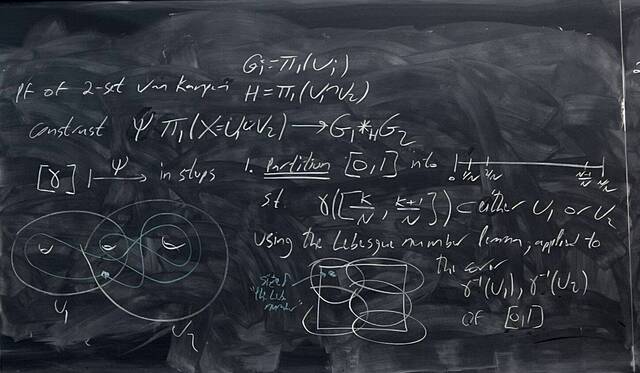250130-154043: Hours 11-12: More van Kampen examples; proof of van Kampen (5).

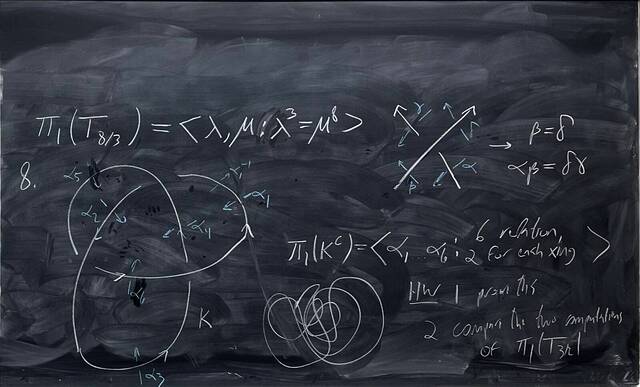250130-154042: Hours 11-12: More van Kampen examples; proof of van Kampen (4).

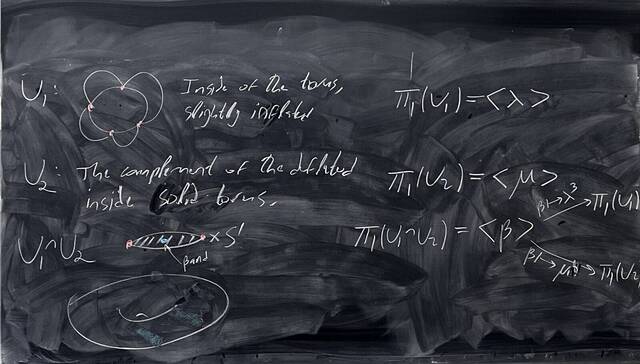250130-154041: Hours 11-12: More van Kampen examples; proof of van Kampen (3).

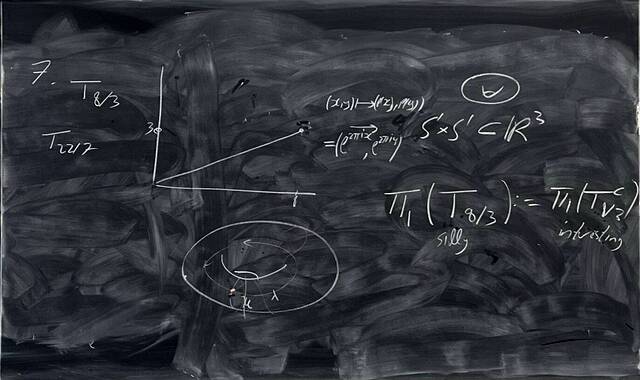250130-154040: Hours 11-12: More van Kampen examples; proof of van Kampen (2).

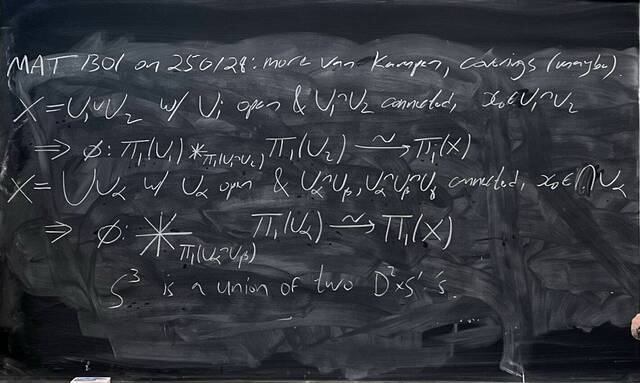250130-154039: Hours 11-12: More van Kampen examples; proof of van Kampen.

250130-145613: Hour 10: van Kampen examples (6).

250130-145612: Hour 10: van Kampen examples (5).

250130-145611: Hour 10: van Kampen examples (4).

250130-145610: Hour 10: van Kampen examples (3).

250130-145609: Hour 10: van Kampen examples (2).

250130-145608: Hour 10: van Kampen examples.

250122-064610: Hours 8-9: Homotopy equivalences, van Kampen (8).

250122-064609: Hours 8-9: Homotopy equivalences, van Kampen (7).

250122-064608: Hours 8-9: Homotopy equivalences, van Kampen (6).

250122-064607: Hours 8-9: Homotopy equivalences, van Kampen (5).

250122-064606: Hours 8-9: Homotopy equivalences, van Kampen (4).

250122-064605: Hours 8-9: Homotopy equivalences, van Kampen (3).

250122-064604: Hours 8-9: Homotopy equivalences, van Kampen (2).

250122-064603: Hours 8-9: Homotopy equivalences, van Kampen.

250121-162901: Mon 250120 H7: Borsuk-Ulam (6).

250121-162900: Mon 250120 H7: Borsuk-Ulam (5).

250121-162859: Mon 250120 H7: Borsuk-Ulam (4).

250121-162858: Mon 250120 H7: Borsuk-Ulam (3).

250121-162857: Mon 250120 H7: Borsuk-Ulam (2).

250121-162856: Mon 250120 H7: Borsuk-Ulam.

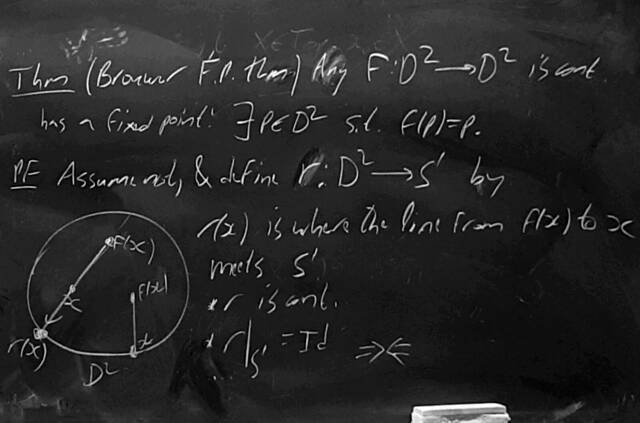250115-112346: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (10).

250115-112345: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (9).

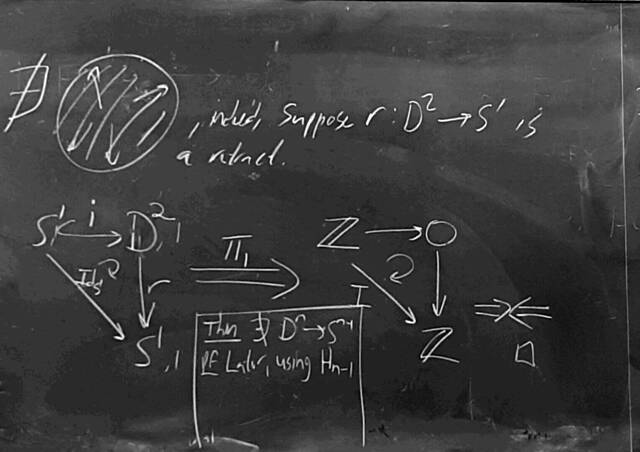250115-112344: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (8).

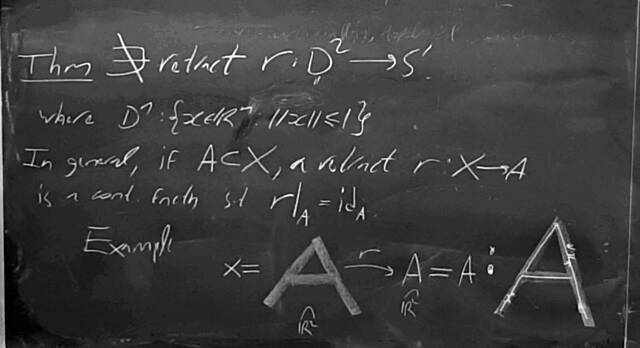250115-112343: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (7).

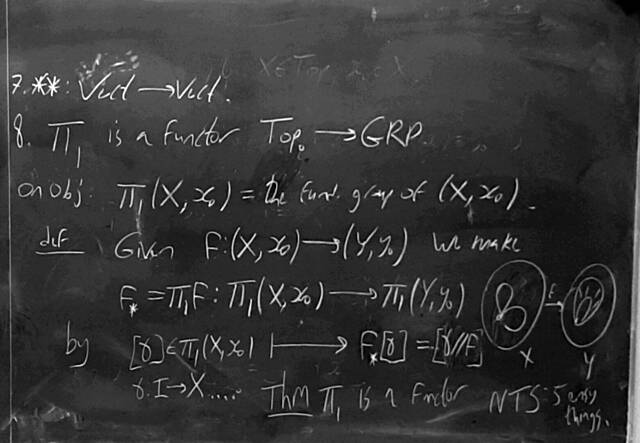250115-112342: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (6).

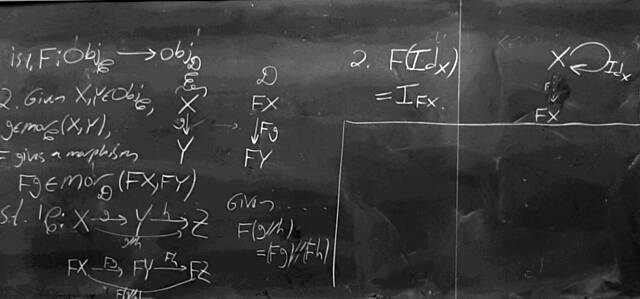250115-112341: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (5).

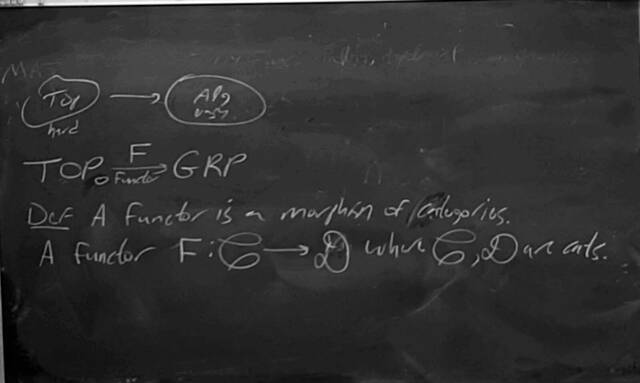250115-112340: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (4).

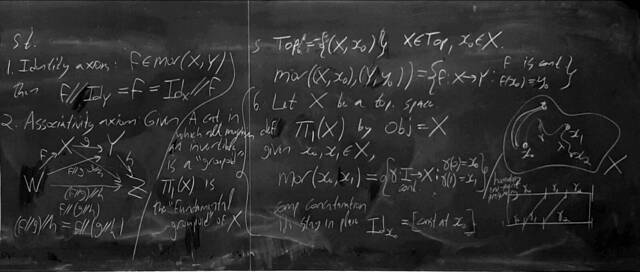250115-112339: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (3).

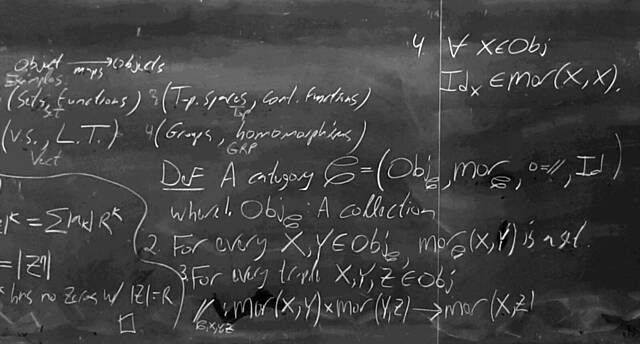250115-112338: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem (2).

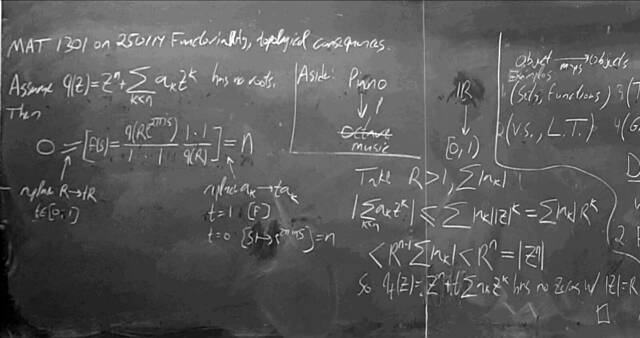250115-112337: Tue 250114 H5-6: Categories and functors, the Brouwer fixed point theorem.

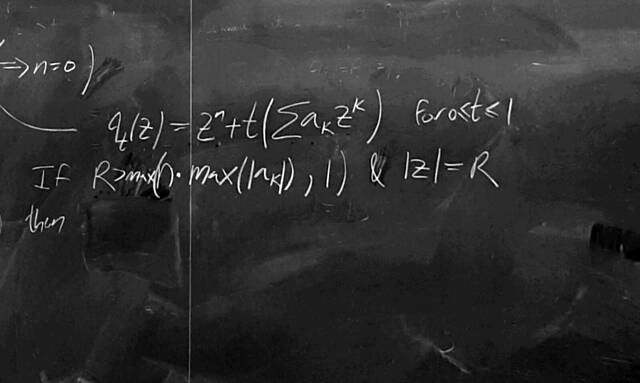250114-075239: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (7).

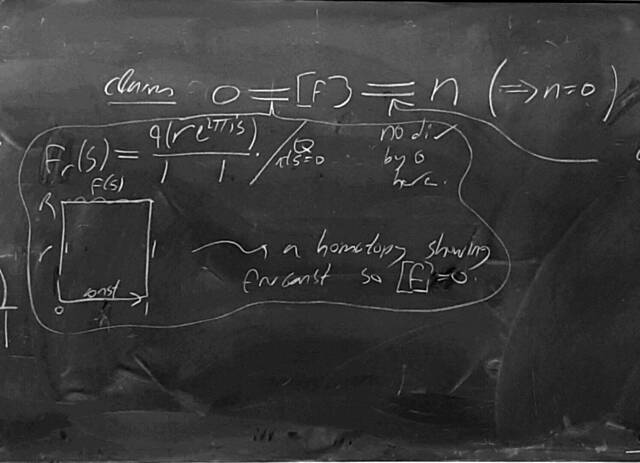250114-075238: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (6).

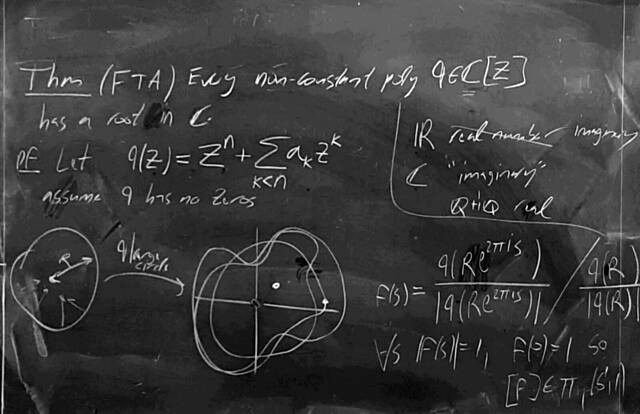250114-075237: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (5).

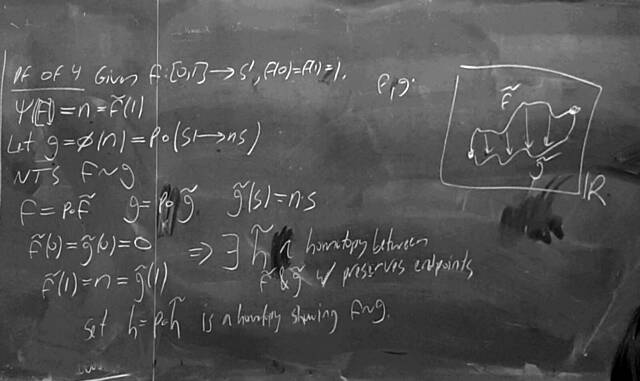250114-075236: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (4).

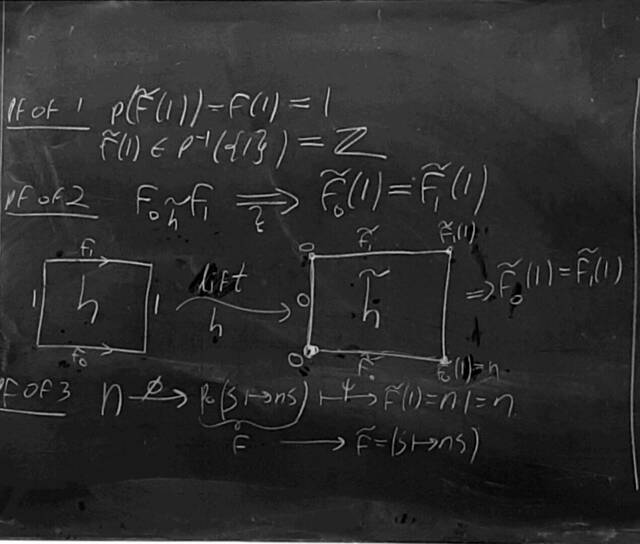250114-075235: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (3).

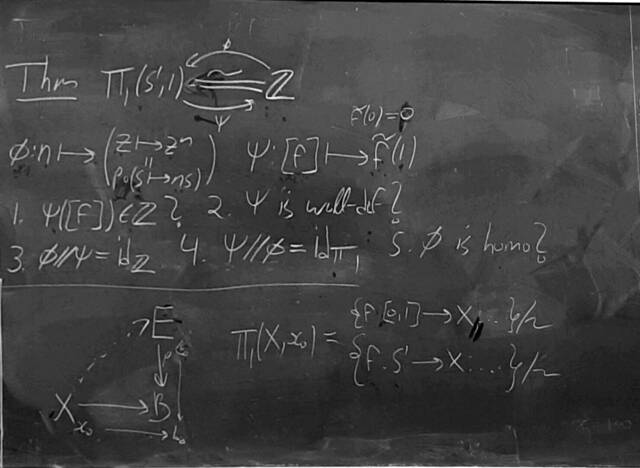250114-075234: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra (2).

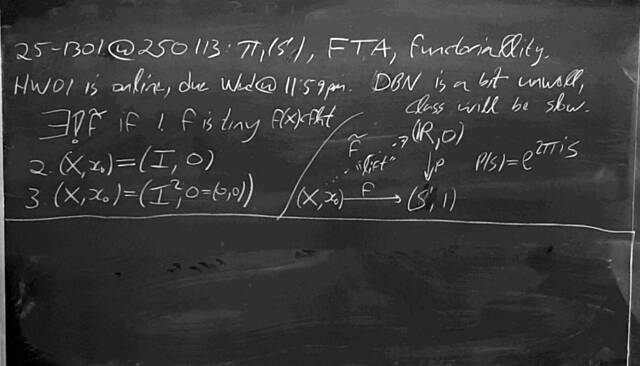250114-075233: Mon 250113 H4: $\pi_1(S^1,1)\simeq{\mathbb Z}$, the fundamental theorem of algebra.

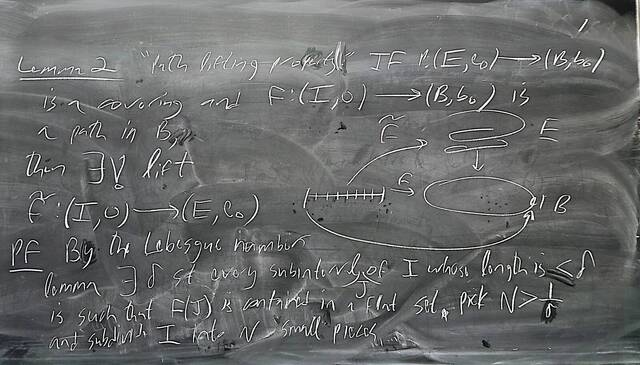250107-162817: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (14).

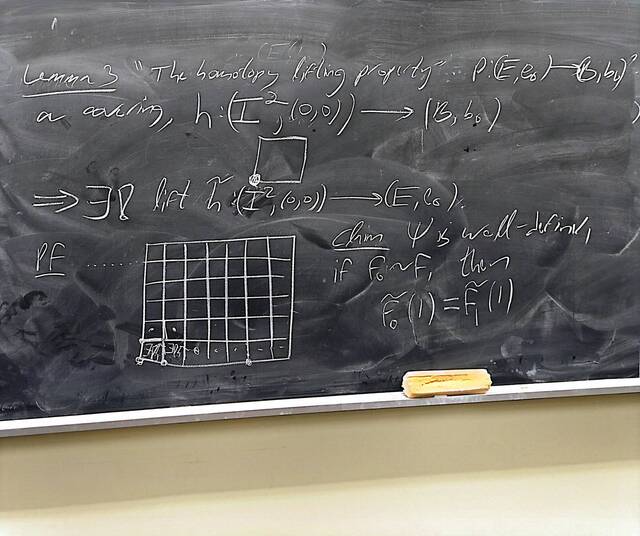250107-162816: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (13).

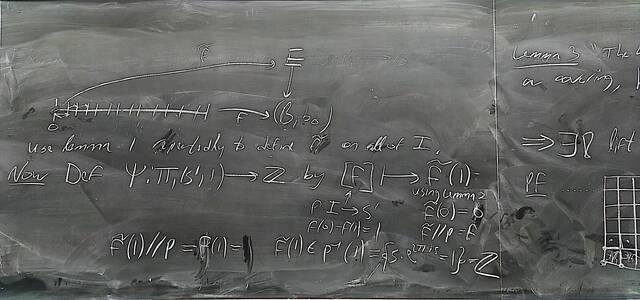250107-162815: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (12).

250107-162814: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (11).

250107-162813: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (10).

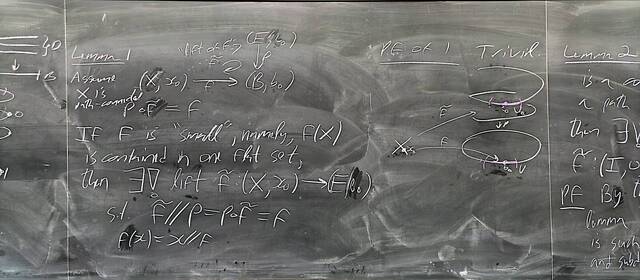250107-162812: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (9).

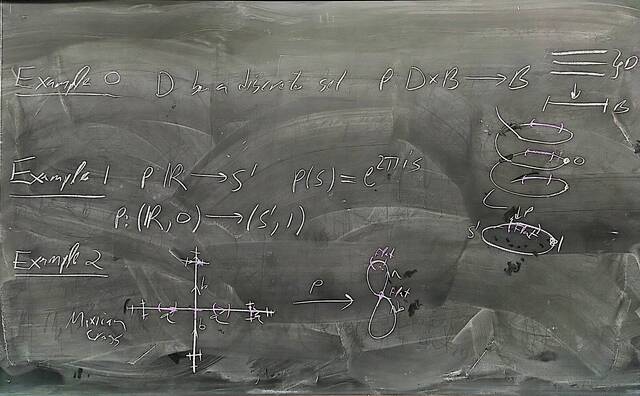250107-162811: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (8).

250107-162810: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (7).

250107-162809: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (6).

250107-162808: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (5).

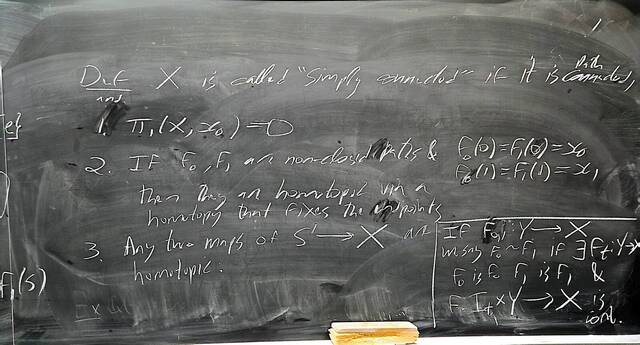250107-162807: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (4).

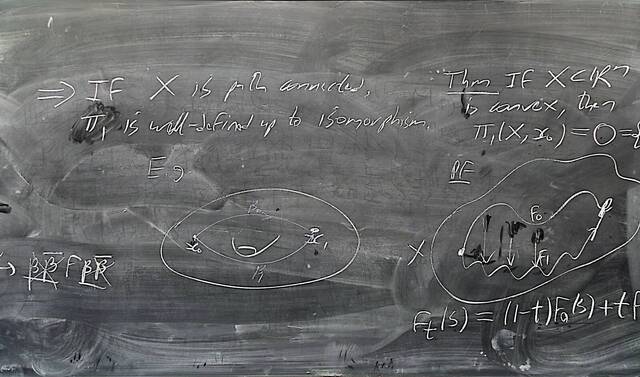250107-162806: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (3).

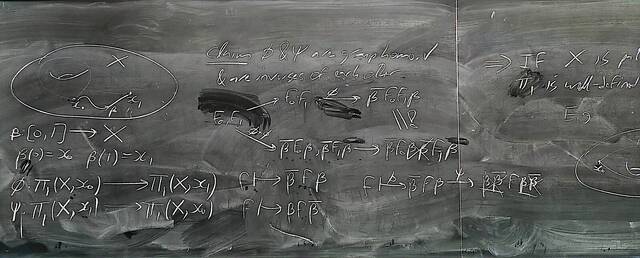250107-162805: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$ (2).

250107-162804: Tue 250107 H2-3: $\pi_1(S^1,1)\simeq{\mathbb Z}$.

250106-142329: Mon 250106 H1: The definition of $\pi_1$ (7).

250106-142328: Mon 250106 H1: The definition of $\pi_1$ (6).

250106-142327: Mon 250106 H1: The definition of $\pi_1$ (5).

250106-142326: Mon 250106 H1: The definition of $\pi_1$ (4).

250106-142325: Mon 250106 H1: The definition of $\pi_1$ (3).

250106-142324: Mon 250106 H1: The definition of $\pi_1$ (2).

250106-142323: Mon 250106 H1: The definition of $\pi_1$.