The Kontsevich Integral for Knotted Trivalent Graphs: Difference between revisions
No edit summary |
|||
| Line 7: | Line 7: | ||
==Step 1 - The Naive Kontsevich Integral== |
==Step 1 - The Naive Kontsevich Integral== |
||
{{07-1352/Schematics of the Kontsevich Integral}} |
|||
| ⚫ | |||
| ⚫ | |||
===The Good=== |
===The Good=== |
||
| Line 21: | Line 23: | ||
==Step 2 - The Renormalized Kontsevich Integral== |
==Step 2 - The Renormalized Kontsevich Integral== |
||
[[Image:07-1352 Renormalizing the Kontsevich Integral.png|400px|center]] |
|||
| ⚫ | |||
| ⚫ | |||
===The Good=== |
===The Good=== |
||
Revision as of 11:50, 6 March 2007
|
The information below is preliminary and cannot be trusted! (v)
Abstract
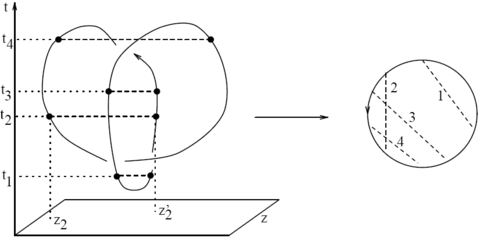
We construct a (very) well-behaved invariant of knotted trivalent graphs using only the Kontsevich integral, in three steps.
Step 1 - The Naive Kontsevich Integral
We define the "naive Kontsevich integral" of a knotted trivalent graph or a slice thereof as in the "standard" picture above, except generalized to graphs in the obvious manner.
The Good
- It has a factorization property.
- For the "braid-like" factors, it has invariance under horizontal deformations.
- It is morally a universal finite type invariant.
The Bad
- It is divergent.
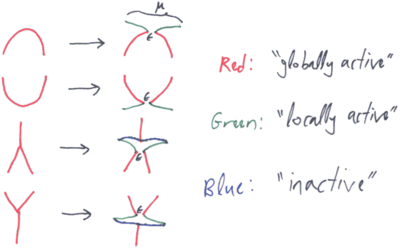
Step 2 - The Renormalized Kontsevich Integral
After choosing a scale and using ideas from renormalization theory as practiced by quantum field theorists, we define the "renormalized Kontsevich integral" of a knotted trivalent graph or a slice thereof as summarized by the picture above.
The Good
- It retains all the good properties of the naive Kontsevich integral.
- It is convergent.
- It is invariant under rigid motions of critical points and trivalent vertices.
- It has sensible behaviour under vertical connect sum, delete and unzip operations.
- It has a sensible behaviour under the changing of the scale .
The Bad
- It is not invariant under non-rigid motions of vertices and critical points.
Step 3 - The Corrected Kontsevich Integral
By correcting caps and cups with factors and , and the two types of trivalent vertices with factors and , we define the "corrected Kontsevich integral" of a knotted trivalent graph or a slice thereof. It has all the good properties we can wish for.
Aside - The Relationship with Drinfel'd's KZ Associator
The Drinfel'd KZ associator is reproduced already by .