0708-1300/Class notes for Tuesday, January 22

0708-1300/Navigation Panel   Add your name / see who's in! # Week of... Links Fall Semester 1 Sep 10 About, Tue, Thu 2 Sep 17 Tue, HW1, Thu 3 Sep 24 Tue, Photo, Thu 4 Oct 1 Questionnaire, Tue, HW2, Thu 5 Oct 8 Thanksgiving, Tue, Thu 6 Oct 15 Tue, HW3, Thu 7 Oct 22 Tue, Thu 8 Oct 29 Tue, HW4, Thu, Hilbert sphere 9 Nov 5 Tue,Thu, TE1 10 Nov 12 Tue, Thu 11 Nov 19 Tue, Thu, HW5 12 Nov 26 Tue, Thu 13 Dec 3 Tue, Thu, HW6 Spring Semester 14 Jan 7 Tue, Thu, HW7 15 Jan 14 Tue, Thu 16 Jan 21 Tue, Thu, HW8 17 Jan 28 Tue, Thu 18 Feb 4 Tue 19 Feb 11 TE2, Tue, HW9, Thu, Feb 17: last chance to drop class R Feb 18 Reading week 20 Feb 25 Tue, Thu, HW10 21 Mar 3 Tue, Thu 22 Mar 10 Tue, Thu, HW11 23 Mar 17 Tue, Thu 24 Mar 24 Tue, HW12, Thu 25 Mar 31 Referendum,Tue, Thu 26 Apr 7 Tue, Thu R Apr 14 Office hours R Apr 21 Office hours F Apr 28 Office hours, Final (Fri, May 2) Register of Good Deeds Errata to Bredon's Book

Announcements go here

Pictures for a Van-Kampen Computation

In[1]:=

<< KnotTheory`

Loading KnotTheory` version of January 13, 2008, 20:30:12.1353.
Read more at http://katlas.org/wiki/KnotTheory.

In[2]:=	TubePlot[TorusKnot[8, 3]]
Out[2]=

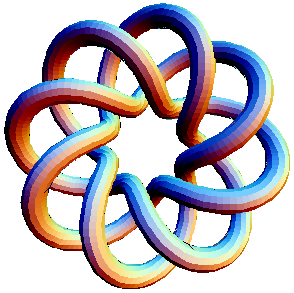
In[3]:=

TC[r1_, t1_,r2_,t2_ ] := { (r1 +r2 Cos[2Pi t2])Cos[2Pi t1], (r1 +r2 Cos[2Pi t2])Sin[2Pi t1], r2 Sin[2Pi t2] };

In[4]:=

InflatedTorus[p_, q_, b_] := ParametricPlot3D[ TC[ 2, p t - q s, 1 + b(p^2 + q^2)s(1 - (p^2 + q^2)s), q t + p s ], {t, 0, 1}, {s, 0, 1/(p^2 + q^2)}, PlotPoints -> {6(p^2 + q^2) + 1, 7}, DisplayFunction -> Identity ];

In[5]:=	GraphicsArray[{{InflatedTorus[3,8,1], InflatedTorus[3,8,-1]}}]
Out[5]=

Typed Notes

First Hour

Today's Agenda:

1) More Examples of Van-Kampen Theorem

2) More Diagrams

3) Proof of Van-Kampen (was not done)

We began by recalling the examples from last class. I will not repeat that here, merely making a few additional comments that came out:

Notation:

Technically, $A*_{H}B$ is poor notion as it implies that knowledge of A, B and H is sufficient to construct $A*_{H}B$. In fact, we ALSO need to know the maps from H into A and B respectively in order for $A*_{H}B$ to be defined.

Aside

Last class we simply wrote down the schematic for the two holed torus as an octagon with the identifications on the edges given last class. We now consider how one arrives at this schematic.

To create the two holed torus one begins with two tori. One then cuts out a small open disk from each torus and then glues the two boundaries together. Let us consider what this looks like when considering a torus as the normal schematic with a square in the plane with the normal identification of the sides. Removing an open disk is equivalent to removing the inside of a loop starting at one of the corners and finishing at that same corner. This is equivalent to making a pentagon with sides $aba^{-1}b^{-1}c$ where c is the added edge.

Consider two such pentagons, gluing along the edge c forms precisely the octagon we had for the two holed torus last class.

Proposition

Letting $\Sigma _{g}$ denote the g holed torus, then $\Sigma _{g}\neq \Sigma _{g'}$

(Note, I used the symbol $\neq$ to as the normal \ncong command doesn't seem to work. Take its meaning in context.)

Aside: Consider a functor from the category of groups to the category of Abelian groups via

$G\mapsto G^{ab}=G/(ab=ba)$

If we have a (homo)morphism from $G\rightarrow H$ then the functor takes $H\rightarrow H^{ab}$ and yields a map $G^{ab}\rightarrow H^{ab}$ such that everything commutes.

Hence we know that $\pi _{1}^{ab}(\Sigma _{g})\cong \mathbb {Z} ^{2g}\neq \mathbb {Z} ^{2g'}\cong \pi _{1}^{ab}(\Sigma _{g'})$

Of course, we need to know that in fact $\mathbb {Z} ^{m}\neq \mathbb {Z} ^{n}$ if $m\neq n$

As such, since the abelianizations are not isomorphic,neither are the original groups and the spaces themselves are not homeomorphic.

Example

$\pi _{1}(\mathbb {R} P^{2})$ is $\pi _{1}$ of the space which can be written as a disk with two antipodal points on the boundary circle on it with the identification that the top path a (going clockwise along the boundary) is glued to the bottom path (also going clock wise). But $\pi _{1}$ of this is just $<a>/(a^{2}=e)\cong \mathbb {Z} /2$

Claim:

Puncturing an n-manifold, $n\geq 3$, does not change $\pi _{1}(M)$. I.e., if $p\in M^{n}$ then $\pi _{1}(M)\cong \pi _{1}(M-\{p\})$

Proof:

Let $U_{1}=M-\{p\}$

$U_{2}$ = a coordinate patch about p.

Then $U_{1}\cap U_{2}=B^{n}-\{p\}\cong S^{n-1}$

If n=3, $\pi _{1}(S^{2})=\{e\}$ as we have computed before.

Hence, $\pi _{1}(M)=\pi _{1}(U_{1})*_{\{\}}\{\}=\pi _{1}(U_{1})$

Now, $\pi _{1}(S^{3})\cong \pi _{1}(S^{3}-\{p\})=\pi _{1}(B^{3})=\{e\}$

Continuing inductively the theorem holds for all n.

Aside:

If X is connected and $b_{1},\ b_{2}\in X$ then $\pi _{1}(X,b_{1})=\pi _{1}(X,b_{2})$. I.e., it does not matter which base point we choose in a connected space, the fundamental group is invariant of this.

Proof

Consider a path $\eta$ from $b_{1}$ to $b_{2}$. The returning path is denoted ${\bar {\eta }}$

Consider a loop from $b_{2}$ called $\gamma$.

Then get a loop from $b_{1}$ via $\gamma \mapsto {\bar {\eta }}\gamma \eta$

Similarly about $b_{2},\gamma \mapsto \eta \gamma {\bar {\eta }}$

Considering the composition we get $\eta {\bar {\eta }}\gamma \eta {\bar {\eta }}\sim \gamma$.