06-1350/Class Notes for Thursday November 16
From Drorbn
Jump to navigationJump to search
| ||||||||||||||||||||||||||||||||||||||||||||||||||||||
Today's Agenda
Formulas are a Chore (Bore?)
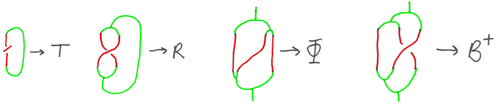
- Sweeping clean a tree and .
- is a VS-algebra (see more at VS, TS and TG Algebras).
- In the coordinates above, write the relations in various algebraic notations.
- R4: or .
- R3: or .
- R2: or .
- R1: .
- But for now, skip the writing of the following relations:
- Symmetry of and of .
- , and
- Idempotence for , , and .
- in terms of and and in terms of .
Exponentiation is a Miracle
- Description of the problem.
- Beads and strands.
- The perturbative approach, linearization.
- The syzygies: relations between the errors.
- The Hochschild complex and homology.