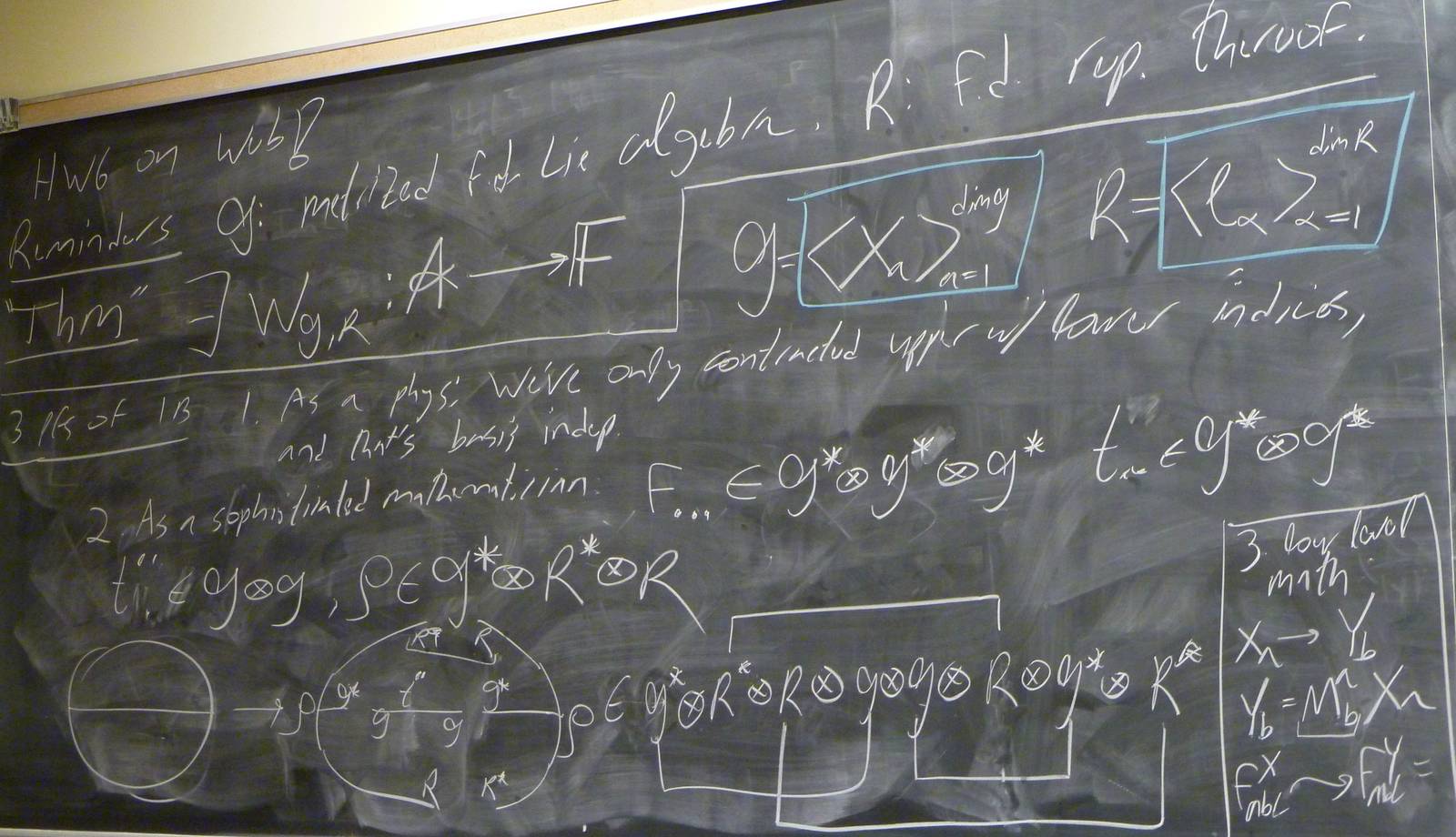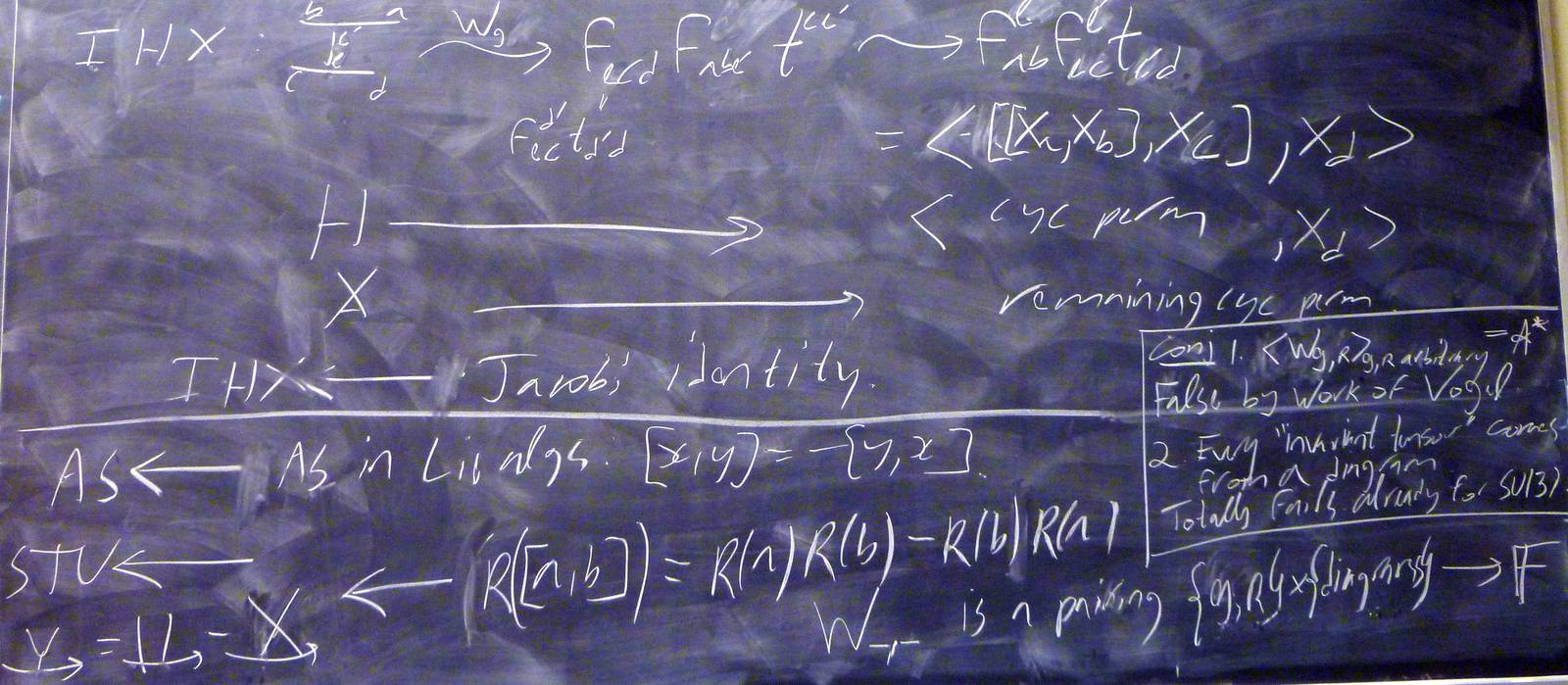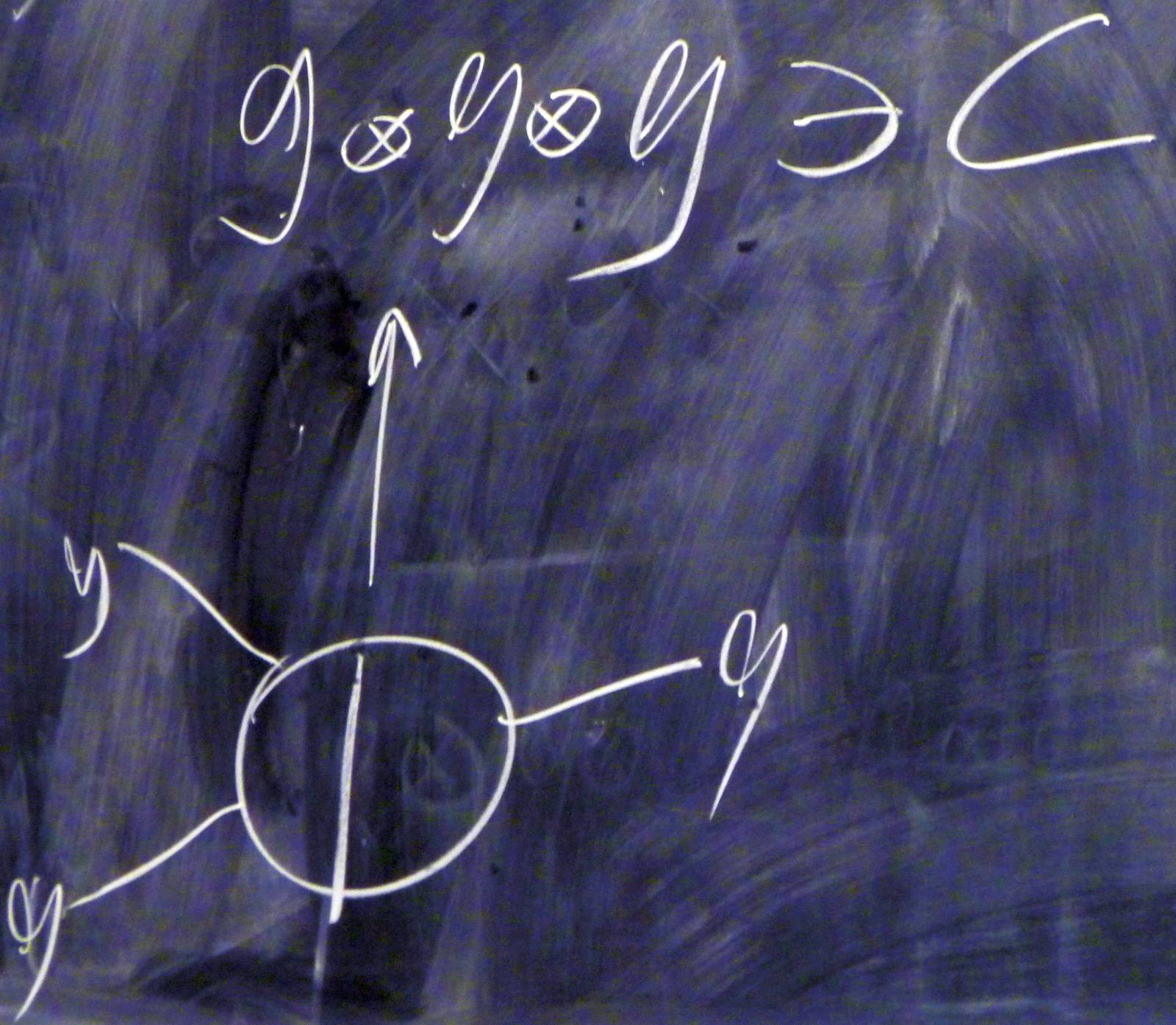refresh
panel Managed by dbnvp: Tip: blackboard shots are taken when the discussion of their content has just ended. To see the beginning of the discussion on a certain blackboard, roll the video to the time of the preceeding blackboard.
panel Managed by dbnvp: Tip: blackboard shots are taken when the discussion of their content has just ended. To see the beginning of the discussion on a certain blackboard, roll the video to the time of the preceeding blackboard.